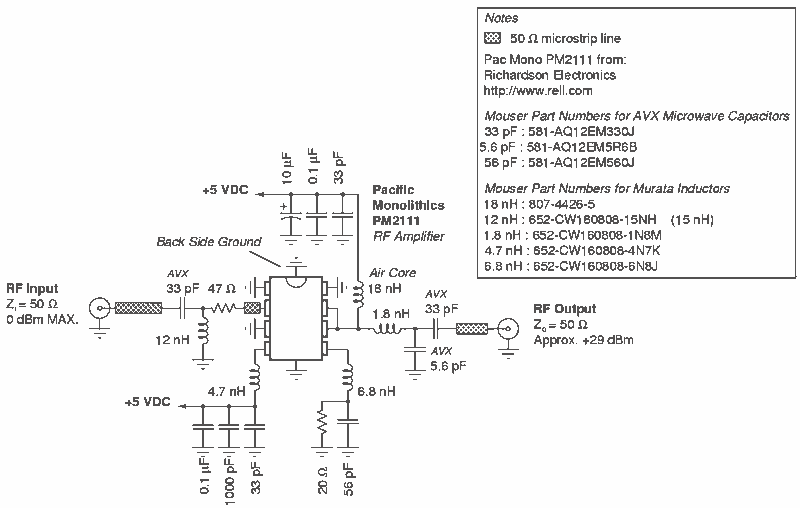
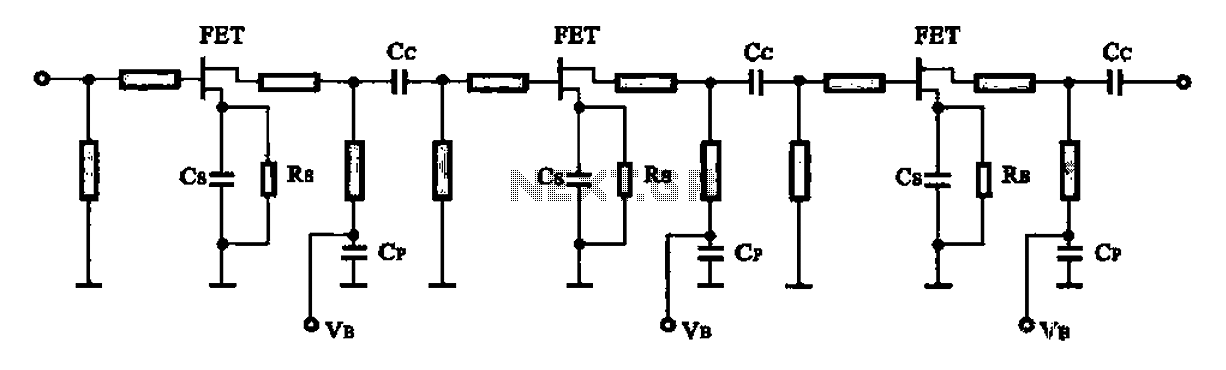
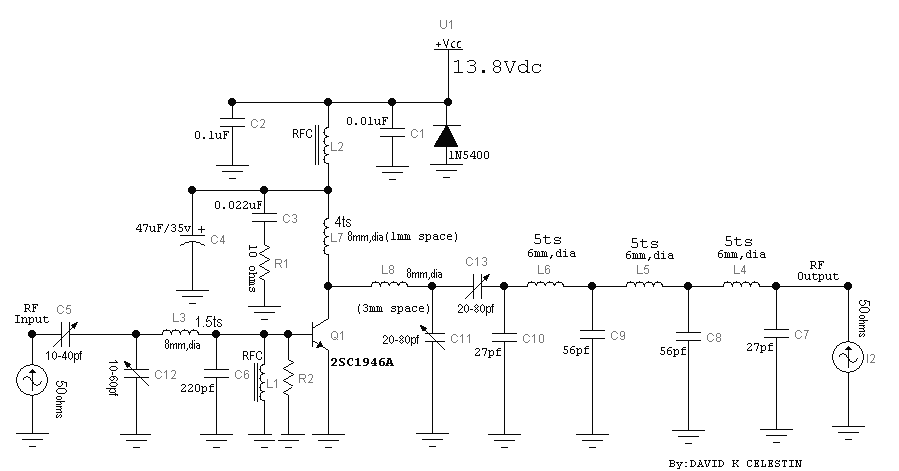
Impedance Matching Networks

1. Definition and Importance of Impedance Matching

Definition and Importance of Impedance Matching

Impedance matching is the process of designing a network that ensures maximum power transfer from a source to a load by making their impedances complex conjugates of each other. In high-frequency systems, mismatched impedances lead to reflected waves, standing waves, and inefficient power delivery, degrading signal integrity and system performance.

Fundamental Principles

For a source with impedance ZS = RS + jXS and a load impedance ZL = RL + jXL, maximum power transfer occurs when:

$$ Z_S = Z_L^* $$

where ZL* denotes the complex conjugate of the load impedance. This condition ensures that the reactive components cancel out, and the real parts are equal, minimizing reflections.

Reflection Coefficient and VSWR

The degree of impedance mismatch is quantified by the reflection coefficient (Γ) and Voltage Standing Wave Ratio (VSWR). The reflection coefficient is given by:

$$ \Gamma = \frac{Z_L - Z_S}{Z_L + Z_S} $$

A perfectly matched load yields Γ = 0, while a complete mismatch results in |Γ| = 1. VSWR, defined as:

$$ \text{VSWR} = \frac{1 + |\Gamma|}{1 - |\Gamma|} $$

provides a measure of standing wave amplitude due to reflections, with an ideal value of 1:1.

Practical Importance

Impedance matching is critical in:

Historical Context

The concept dates back to Oliver Heaviside’s work on transmission lines in the late 19th century. Modern applications extend to telecommunications, radar systems, and integrated circuit design, where impedance matching ensures optimal performance in miniaturized and high-frequency environments.

Mathematical Derivation of Power Transfer

The power delivered to the load PL is maximized when the source and load impedances satisfy the conjugate matching condition. Starting from the power expression:

$$ P_L = \frac{1}{2} \text{Re}\{V_L I_L^*\} $$

Substituting VL = VS Z_L / (Z_S + Z_L) and I_L = V_S / (Z_S + Z_L), we derive:

$$ P_L = \frac{|V_S|^2 R_L}{2 |Z_S + Z_L|^2} $$

Maximizing PL with respect to ZL confirms that ZL = Z_S^* yields the highest power transfer.

Source (ZS) Load (ZL) Transmission Line

The diagram illustrates a basic impedance matching scenario between a source and a load connected via a transmission line. Reflections occur if ZL ≠ Z_S^*, reducing efficiency.

1.2 Reflection Coefficient and VSWR

When an electromagnetic wave encounters an impedance discontinuity along a transmission line, a portion of the incident wave reflects back toward the source. The reflection coefficient (Γ) quantifies this mismatch, defined as the ratio of the reflected voltage wave (Vr) to the incident voltage wave (Vi):

$$ \Gamma = \frac{V_r}{V_i} = \frac{Z_L - Z_0}{Z_L + Z_0} $$

Here, ZL is the load impedance and Z0 is the characteristic impedance of the transmission line. The reflection coefficient is a complex quantity, with magnitude |Γ| ranging from 0 (perfect match) to 1 (total reflection), and phase indicating the relative timing of the reflected wave.

Voltage Standing Wave Ratio (VSWR)

VSWR measures the standing wave pattern resulting from the interference of incident and reflected waves. It is defined as the ratio of maximum to minimum voltage amplitudes along the line:

$$ \text{VSWR} = \frac{1 + |\Gamma|}{1 - |\Gamma|} $$

For a perfectly matched load (Γ = 0), VSWR equals 1, indicating no standing waves. As mismatch increases, VSWR rises toward infinity for total reflection (|Γ| = 1). Practical systems often specify VSWR limits—for instance, a VSWR below 2:1 is typical in RF systems to minimize power loss.

Relationship Between Γ and VSWR

The reflection coefficient and VSWR are directly related. Given VSWR, |Γ| can be derived as:

$$ |\Gamma| = \frac{\text{VSWR} - 1}{\text{VSWR} + 1} $$

This relationship is particularly useful in measurements, where VSWR is often easier to observe directly using a slotted line or network analyzer.

Practical Implications

High VSWR leads to several operational challenges:

In antenna systems, for example, a VSWR of 1.5:1 corresponds to roughly 4% reflected power, while 3:1 reflects 25% of the incident power. Modern impedance matching networks aim to minimize Γ and VSWR across the operating bandwidth.

Measurement Techniques

Common methods for determining VSWR include:

VNAs are now the standard for laboratory measurements, offering Smith chart displays that visualize impedance and reflection coefficient simultaneously.

Standing Wave Pattern and Reflection Coefficient Visualization A diagram showing a transmission line with incident and reflected waves, and the resulting standing wave pattern with labeled voltage maxima and minima. Transmission Line V_i V_r V_max V_max V_min Z_L Z_0 Γ = V_r/V_i
Diagram Description: The section discusses standing wave patterns and reflection phenomena, which are inherently spatial and visual concepts.

1.3 Power Transfer and Efficiency

Maximum power transfer occurs when the load impedance ZL is the complex conjugate of the source impedance ZS. This condition, known as the conjugate matching theorem, ensures that the reactive components cancel while the resistive components match. For a source impedance ZS = RS + jXS, the optimal load impedance is ZL = RS − jXS.

$$ P_{\text{max}} = \frac{|V_S|^2}{4R_S} $$

where VS is the source voltage and RS is the real part of the source impedance. The efficiency η under matched conditions is only 50%, since half the power is dissipated in the source resistance. This trade-off between power transfer and efficiency is critical in RF systems, where maximizing signal strength often takes precedence over minimizing losses.

Derivation of Maximum Power Transfer

Consider a Thévenin-equivalent source with impedance ZS = RS + jXS driving a load ZL = RL + jXL. The power delivered to the load is:

$$ P_L = I^2 R_L = \left( \frac{V_S}{\sqrt{(R_S + R_L)^2 + (X_S + X_L)^2}} \right)^2 R_L $$

To maximize PL, the denominator must be minimized. This occurs when XL = −XS (canceling reactance) and RL = RS (resistive matching). Substituting these conditions yields the maximum power formula above.

Practical Considerations

While conjugate matching maximizes power transfer, it is not always desirable:

Efficiency in Mismatched Conditions

When ZL ≠ ZS*, the power transfer efficiency is given by:

$$ \eta = \frac{P_L}{P_{\text{in}}} = \frac{4 R_S R_L}{|Z_S + Z_L|^2} $$

Reflection coefficient Γ quantifies impedance mismatch:

$$ \Gamma = \frac{Z_L - Z_S^*}{Z_L + Z_S} $$

Power loss due to reflections is |Γ|2, making Γ a key metric in RF design. Smith charts graphically represent Γ and aid in matching network synthesis.

Case Study: Antenna Matching

A 50 Ω transmitter driving a 75 Ω antenna via a quarter-wave transformer demonstrates practical matching. The transformer’s characteristic impedance Z0 is:

$$ Z_0 = \sqrt{Z_{\text{in}} Z_{\text{out}}} = \sqrt{50 \times 75} \approx 61.2 \, \Omega $$

This eliminates reflections at the design frequency, though bandwidth is limited by the transformer’s frequency response.

Impedance Matching and Power Transfer Schematic diagram illustrating impedance matching between a Thévenin source (Z_S) and a load (Z_L), showing power flow and reflection coefficient (Γ). ZS ZS = RS + jXS ZL ZL = RL + jXL Pmax Γ
Diagram Description: The section involves complex impedance relationships and power transfer conditions that are best visualized with vector diagrams or Smith chart representations.

2. L-Section Matching Networks

2.1 L-Section Matching Networks

L-section matching networks are the simplest and most widely used impedance matching structures, consisting of just two reactive components—either a series inductor with a shunt capacitor or a series capacitor with a shunt inductor. Their name derives from the "L" shape formed by the components in the circuit diagram. These networks are particularly useful in RF and microwave applications where compact, low-loss matching is required.

Fundamental Operation

The L-section network transforms a load impedance ZL = RL + jXL to a desired source impedance ZS = RS + jXS by canceling the reactive part and adjusting the resistive part. The design relies on the quality factor Q, which is determined by the impedance transformation ratio:

$$ Q = \sqrt{\frac{R_{\text{high}}}{R_{\text{low}}} - 1 $$

where Rhigh is the larger of the two resistances (RS or RL), and Rlow is the smaller one. The reactive components are then calculated using:

$$ X_{\text{series}} = Q R_{\text{low}} $$ $$ X_{\text{shunt}} = \frac{R_{\text{high}}}{Q} $$

Design Procedure

For a given load impedance ZL and source impedance ZS, the design steps are as follows:

  1. Determine the resistance ratio: Identify whether RS > RL or vice versa to decide the topology (shunt-first or series-first).
  2. Calculate Q: Use the equation above to find the required quality factor.
  3. Compute reactances: Derive the series and shunt reactances based on the chosen configuration.
  4. Select component types: Choose inductors or capacitors to realize the reactances at the operating frequency.

Topology Variations

Two primary configurations exist:

Practical Considerations

While L-sections are simple, they have limitations:

Example Calculation

Consider matching a 50 Ω source to a 200 Ω load at 100 MHz. Since RL > RS, we use a series-shunt (low-pass) configuration:

$$ Q = \sqrt{\frac{200}{50} - 1} = \sqrt{3} \approx 1.732 $$ $$ X_{\text{series}} = 1.732 \times 50 = 86.6 \, \Omega $$ $$ X_{\text{shunt}} = \frac{200}{1.732} \approx 115.5 \, \Omega $$

At 100 MHz, this translates to a series inductor of 137.8 nH and a shunt capacitor of 13.8 pF.

L C

2.2 Pi and T-Section Matching Networks

Pi (π) and T-section networks are widely used in RF and microwave engineering to achieve impedance matching between a source and load with minimal reflections. These networks consist of reactive components (inductors and capacitors) arranged in specific topologies to transform impedances over a desired bandwidth.

Pi-Network Configuration

The Pi-network consists of two shunt components (typically capacitors) and one series component (typically an inductor). The admittance transformation follows a step-by-step process:

$$ Y_{in} = j\omega C_1 + \frac{1}{j\omega L + \frac{1}{j\omega C_2 + Y_L}} $$

To match a load impedance ZL to a source impedance ZS, the component values are derived by solving the matching conditions:

$$ Q = \sqrt{\frac{R_{high}}{R_{low}} - 1} $$ $$ C_1 = \frac{Q}{\omega R_{high}}, \quad C_2 = \frac{Q}{\omega R_{low}}, \quad L = \frac{R_{low}}{\omega Q} $$

where Rhigh is the larger of ZS or ZL, and Rlow is the smaller value. The Pi-network is particularly useful in high-power applications due to its ability to handle large voltage swings across the shunt capacitors.

T-Network Configuration

The T-network consists of two series components (typically inductors) and one shunt component (typically a capacitor). Its impedance transformation follows:

$$ Z_{in} = j\omega L_1 + \frac{1}{j\omega C + \frac{1}{j\omega L_2 + Z_L}} $$

The matching equations for the T-network are derived similarly, but with a focus on impedance rather than admittance:

$$ Q = \sqrt{\frac{R_{high}}{R_{low}} - 1} $$ $$ L_1 = \frac{R_{low} Q}{\omega}, \quad L_2 = \frac{R_{high} Q}{\omega}, \quad C = \frac{1}{\omega Q \sqrt{R_{high} R_{low}}} $$

T-networks are advantageous in low-impedance environments, such as antenna matching, where series inductors help mitigate resistive losses.

Design Trade-offs and Practical Considerations

Both Pi and T-networks introduce a finite Q, which determines bandwidth and selectivity. Higher Q yields narrower bandwidth but sharper roll-off, while lower Q provides broader matching at the cost of reduced attenuation of out-of-band signals.

Modern implementations often combine these networks with transmission line stubs or distributed elements for broadband performance in microwave circuits.

Pi and T-Network Topologies Schematic comparison of Pi-network (C-L-C) and T-network (L-C-L) impedance matching configurations with labeled components and source/load impedances. Z_S C1 L C2 Z_L Pi-Network (C-L-C) Z_S L1 C L2 Z_L T-Network (L-C-L) Pi and T-Network Topologies
Diagram Description: The Pi and T-network configurations are highly visual topologies with specific component arrangements that are difficult to visualize from equations alone.

2.3 Transformer-Based Matching

Transformer-based impedance matching leverages the turns ratio of a transformer to scale impedances between source and load. The ideal transformer model assumes no losses, infinite inductance, and perfect coupling, allowing impedance transformation purely through the square of the turns ratio n.

Impedance Transformation Principle

For an ideal transformer with primary-to-secondary turns ratio n = Np/Ns, the impedance transformation follows:

$$ Z_{in} = n^2 Z_L $$

where Zin is the input impedance seen at the primary and ZL is the load impedance connected to the secondary. This relationship holds for both resistive and complex loads.

Practical Transformer Considerations

Real transformers exhibit non-idealities that affect matching performance:

The quality factor Q of the transformer determines its frequency selectivity:

$$ Q = \frac{\omega L}{R} $$

where L is the leakage inductance and R is the equivalent series resistance.

Broadband Matching with Transmission Line Transformers

For wideband applications (e.g., RF systems), transmission line transformers (TLTs) provide superior performance over conventional transformers. A TLT combines transmission line behavior with magnetic coupling, achieving bandwidths exceeding 10:1. The characteristic impedance Z0 of the transmission line section must satisfy:

$$ Z_0 = \sqrt{Z_{in} Z_L} $$

Common configurations include the Guanella 1:4 transformer and Ruthroff 1:9 transformer, which use stacked transmission lines to achieve precise impedance ratios.

Design Example: 50 Ω to 200 Ω Matching

To match a 50 Ω source to a 200 Ω load:

  1. Calculate required turns ratio: n = √(200/50) = 2
  2. Select a ferrite core with sufficient permeability for the operating frequency
  3. Wind primary with N turns and secondary with N/2 turns
  4. Verify bandwidth using network analyzer measurements

For a TLT implementation, two 50 Ω coaxial cables can be connected in series at the load and parallel at the source to achieve the 1:4 impedance ratio.

Applications in RF Systems

Transformer matching networks are ubiquitous in:

The table below compares common transformer types for RF applications:

Type Bandwidth Power Handling Typical Applications
Ferrite Core Narrowband High HF/VHF power amplifiers
Air Core Medium Medium UHF matching networks
TLT Wideband Low-Medium Broadband receivers
Transformer Impedance Matching Principle Schematic diagram showing a transformer with primary and secondary windings, illustrating the impedance matching principle with turns ratio and impedance transformation formula. Z_in Z_L n = N_p/N_s N_p N_s Z_in = n²Z_L
Diagram Description: The diagram would physically show the transformer turns ratio and impedance transformation relationship, illustrating how primary and secondary windings connect to source/load.

2.4 Stub Matching Techniques

Stub matching is a widely used method for impedance matching in RF and microwave circuits, where a transmission line stub—either open or short-circuited—is strategically placed to cancel the reactive component of the load impedance. The technique leverages the standing wave properties of transmission lines to achieve precise matching without lumped elements.

Single-Stub Matching

The simplest form involves a single stub placed at a specific distance from the load. The stub's length and position are calculated to introduce an admittance that cancels the load's susceptance. For a load impedance ZL, the normalized admittance YL = 1/ZL is plotted on the Smith chart. The stub's position d is determined by moving toward the generator until the real part of the admittance equals the characteristic admittance Y0.

$$ Y_{in} = Y_0 + jB $$

The stub's susceptance jB must satisfy:

$$ jB = -j\text{Im}(Y_{in}) $$

For a short-circuited stub, the length l is derived from:

$$ l = \frac{\lambda}{2\pi} \arctan\left(\frac{B}{Y_0}\right) $$

For an open-circuited stub, replace arctan with arccot.

Double-Stub Matching

Double-stub matching provides greater flexibility by using two stubs separated by a fixed distance (typically λ/8 or 3λ/8). The first stub adjusts the susceptance to a value that the second stub can fully cancel. The design involves iterative Smith chart manipulations or analytical solutions to the matching equations:

$$ Y_1 = Y_0 \frac{Y_L + jY_0 \tan(\beta d_1)}{Y_0 + jY_L \tan(\beta d_1)} $$
$$ Y_2 = Y_0 + jB_2 $$

where d1 is the distance to the first stub, and B2 is the susceptance introduced by the second stub.

Practical Considerations

Example: 50 Ω to 75 Ω Matching

For a 75 Ω load at 1 GHz on a 50 Ω line (εr = 4.5), the stub position d ≈ 0.125λ (15.8 mm) yields a normalized admittance of 1 + j1.22. A short-circuited stub of length 0.148λ (18.7 mm) cancels the susceptance. The physical length accounts for the substrate's effective permittivity:

$$ l_{phys} = l \cdot \frac{c}{f \sqrt{\epsilon_{eff}}} $$
Smith chart illustration of single-stub matching Normalized load (1.5 + j0) Matching point (1 + j1.22)
Smith Chart with Single-Stub Matching Process A Smith chart showing the single-stub matching process, including the normalized load point, matching trajectory, and admittance circles. Y₀ Circle Matching Path Normalized Load (1.5 + j0) Matching Point (1 + j1.22) Stub Position Re Im
Diagram Description: The section describes Smith chart manipulations and stub positioning, which are inherently spatial concepts requiring visualization of admittance transformations and stub effects.

3. Smith Chart Applications

3.1 Smith Chart Applications

Graphical Representation of Complex Impedance

The Smith Chart is a polar plot of the complex reflection coefficient Γ, where normalized impedance (Z/Z0) is mapped onto a unit circle. Each point on the chart corresponds to a unique impedance value, with the center representing Z = Z0 (perfect match). The horizontal axis denotes pure resistance, while the outer circle represents purely reactive components.

$$ \Gamma = \frac{Z_L - Z_0}{Z_L + Z_0} $$

Key Features and Conventions

Practical Applications in Matching Networks

1. Single-Stub Matching

For a load impedance ZL, the Smith Chart simplifies locating the stub position (d) and length (l):

  1. Plot ZL and convert to admittance (YL).
  2. Move toward the generator until intersecting the unity conductance circle.
  3. Calculate the stub’s susceptance to cancel the residual reactance.
$$ Y_{in} = Y_0 + jB $$

2. L-Section Design

The chart enables rapid component selection for L-networks by tracing paths along constant resistance/reactance circles. For a target impedance Zin:

Advanced Techniques

Impedance Transformation with Transmission Lines

The Smith Chart visualizes the impedance variation along a transmission line of length l as a rotation by 2βl radians, where β is the propagation constant. This aids in designing quarter-wave transformers:

$$ Z_{in} = Z_0 \frac{Z_L + jZ_0 an(\beta l)}{Z_0 + jZ_L an(\beta l)} $$

Noise Figure Optimization

By overlaying noise figure contours (for transistor amplifiers), the chart identifies source impedances that minimize noise while maintaining gain. Trade-offs between Γopt (optimal noise) and ΓMS (maximum gain) become visually apparent.

Z/Z₀=1
Smith Chart with Key Features A polar plot of the Smith Chart showing resistance circles, reactance arcs, wavelength scale, and normalized impedance points. 0λ 0.25λ 0.5λ 0.75λ R=2 R=1 R=0.5 R=0.2 +jX -jX Γ Z/Z₀=1
Diagram Description: The diagram would physically show the Smith Chart's resistance circles, reactance arcs, and wavelength scales, which are spatial relationships central to impedance matching.

3.2 Analytical Design Methods

Analytical design methods for impedance matching networks rely on closed-form mathematical solutions to transform a given load impedance ZL to a desired source impedance ZS. These methods are particularly useful when designing narrowband matching networks, where precise control over the frequency response is required.

L-Section Matching

The L-section is the simplest matching network, consisting of two reactive elements (inductor and capacitor) arranged in an L-configuration. The analytical design procedure involves solving for the component values that satisfy the matching condition:

$$ Z_{in} = Z_S^* $$

where Zin is the input impedance of the network when terminated with ZL. For a given load impedance ZL = RL + jXL, the required reactances X1 and X2 can be derived as:

$$ X_1 = \pm \sqrt{R_L (R_S - R_L)} - X_L $$ $$ X_2 = \mp \frac{R_S \sqrt{R_L (R_S - R_L)}}{R_S - R_L} $$

The signs depend on whether the first element is a series inductor/capacitor or a shunt inductor/capacitor. This method guarantees a perfect match at a single frequency but provides no control over bandwidth.

Pi and T-Section Matching

For higher-Q matching or when additional degrees of freedom are needed, Pi and T-section networks are employed. These consist of three reactive elements, allowing control over both the matching condition and the quality factor Q.

The design equations for a Pi-network are derived by solving the equivalent admittance transformation. Given a desired Q, the susceptances B1, B2, and reactance X are:

$$ B_1 = \frac{Q}{R_S} + \frac{1}{X_L} $$ $$ B_2 = \frac{Q}{R_L} + \frac{1}{X_L} $$ $$ X = \frac{Q + \sqrt{(1 + Q^2) R_S / R_L - 1}}{1 + Q^2} \cdot R_S $$

Similarly, T-section networks use impedance transformations instead of admittance, with analogous design equations.

Smith Chart-Based Design

The Smith chart provides a graphical method for impedance matching, allowing rapid visualization of complex impedance transformations. Analytical design using the Smith chart involves:

For a series inductor, the impedance transformation follows:

$$ Z_{new} = Z_L + j\omega L $$

while a shunt capacitor follows:

$$ Y_{new} = Y_L + j\omega C $$

Bandwidth Considerations

The analytical methods above assume a single-frequency match. To estimate bandwidth, the quality factor Q of the matching network must be considered. For an L-section, the fractional bandwidth is approximately:

$$ \text{BW} \approx \frac{2}{Q} $$

where Q is determined by the ratio of the center frequency to the 3-dB bandwidth. Higher-order networks (Pi, T) allow for explicit control over Q, enabling wider or narrower bandwidths as needed.

Impedance Matching Network Configurations and Smith Chart Transformations Illustration of L-section, Pi, and T-section impedance matching networks with corresponding Smith chart transformations. Z_S Z_L X1 X2 L-Section Z_S Z_L B1 X B2 Pi Network Z_S Z_L X1 X2 T Network Impedance Matching Path Z_S Z_L Intermediate Q=1 Q=2 Smith Chart Transformations
Diagram Description: The section describes L-section, Pi, and T-section configurations which are spatial arrangements of components, and the Smith chart's graphical impedance transformations.

3.3 Simulation and Optimization Tools

Modern impedance matching network design relies heavily on computational tools to simulate performance, optimize component values, and validate theoretical models. Analytical solutions often fall short when dealing with complex, multi-stage networks or broadband matching requirements, necessitating the use of specialized software.

Electromagnetic (EM) and Circuit Simulators

Full-wave electromagnetic simulators like ANSYS HFSS, CST Microwave Studio, and COMSOL Multiphysics solve Maxwell's equations numerically, providing accurate S-parameter extraction for distributed matching structures. For lumped-element networks, circuit simulators such as SPICE, ADS (Keysight Advanced Design System), and QUCS solve Kirchhoff's laws with nonlinear device models.

The scattering parameters (S-parameters) of a matching network can be derived from the voltage and current solutions. For a two-port network:

$$ S_{11} = \frac{b_1}{a_1} \bigg|_{a_2=0}, \quad S_{21} = \frac{b_2}{a_1} \bigg|_{a_2=0} $$

where a and b represent incident and reflected waves, respectively. Simulators compute these parameters across frequency sweeps, enabling visualization of impedance transformations.

Optimization Algorithms

Gradient-based methods (e.g., conjugate gradient, quasi-Newton) and evolutionary algorithms (e.g., genetic algorithms, particle swarm optimization) automate component value selection. The objective function typically minimizes reflection coefficient magnitude:

$$ \min \left( \max \left( |\Gamma(f)| \right) \right), \quad \Gamma(f) = \frac{Z_{\text{in}}(f) - Z_0}{Z_{\text{in}}(f) + Z_0} $$

where Zin(f) is the input impedance and Z0 is the reference impedance. Constraints may include Q-factor limits, physical component tolerances, or fabrication rules.

Practical Workflow

For example, a 50Ω to 75Ω match at 1 GHz might begin with a single-stub tuner in ADS, then transition to a stepped-impedance microstrip model in HFSS for layout-aware optimization.

Commercial vs. Open-Source Tools

High-frequency industrial designs often require proprietary tools like Cadence AWR or Sonnet for their validated device libraries and process design kits (PDKs). Academic researchers frequently use OpenEMS or ngSPICE due to their flexibility and zero licensing costs. Hybrid workflows, such as optimizing in Python (scikit-rf, PyAEDT) and validating in commercial tools, are increasingly common.

Real-world case studies demonstrate that automated optimization can reduce matching network size by 30–50% compared to manual tuning in multi-band RF front-ends, while maintaining >15 dB return loss across all target bands.

3.3 Simulation and Optimization Tools

Modern impedance matching network design relies heavily on computational tools to simulate performance, optimize component values, and validate theoretical models. Analytical solutions often fall short when dealing with complex, multi-stage networks or broadband matching requirements, necessitating the use of specialized software.

Electromagnetic (EM) and Circuit Simulators

Full-wave electromagnetic simulators like ANSYS HFSS, CST Microwave Studio, and COMSOL Multiphysics solve Maxwell's equations numerically, providing accurate S-parameter extraction for distributed matching structures. For lumped-element networks, circuit simulators such as SPICE, ADS (Keysight Advanced Design System), and QUCS solve Kirchhoff's laws with nonlinear device models.

The scattering parameters (S-parameters) of a matching network can be derived from the voltage and current solutions. For a two-port network:

$$ S_{11} = \frac{b_1}{a_1} \bigg|_{a_2=0}, \quad S_{21} = \frac{b_2}{a_1} \bigg|_{a_2=0} $$

where a and b represent incident and reflected waves, respectively. Simulators compute these parameters across frequency sweeps, enabling visualization of impedance transformations.

Optimization Algorithms

Gradient-based methods (e.g., conjugate gradient, quasi-Newton) and evolutionary algorithms (e.g., genetic algorithms, particle swarm optimization) automate component value selection. The objective function typically minimizes reflection coefficient magnitude:

$$ \min \left( \max \left( |\Gamma(f)| \right) \right), \quad \Gamma(f) = \frac{Z_{\text{in}}(f) - Z_0}{Z_{\text{in}}(f) + Z_0} $$

where Zin(f) is the input impedance and Z0 is the reference impedance. Constraints may include Q-factor limits, physical component tolerances, or fabrication rules.

Practical Workflow

For example, a 50Ω to 75Ω match at 1 GHz might begin with a single-stub tuner in ADS, then transition to a stepped-impedance microstrip model in HFSS for layout-aware optimization.

Commercial vs. Open-Source Tools

High-frequency industrial designs often require proprietary tools like Cadence AWR or Sonnet for their validated device libraries and process design kits (PDKs). Academic researchers frequently use OpenEMS or ngSPICE due to their flexibility and zero licensing costs. Hybrid workflows, such as optimizing in Python (scikit-rf, PyAEDT) and validating in commercial tools, are increasingly common.

Real-world case studies demonstrate that automated optimization can reduce matching network size by 30–50% compared to manual tuning in multi-band RF front-ends, while maintaining >15 dB return loss across all target bands.

4. Frequency Response and Bandwidth

Frequency Response and Bandwidth

The frequency response of an impedance matching network defines its ability to efficiently transfer power across a range of frequencies. At the center frequency f₀, the network achieves perfect matching, but deviations from this frequency introduce reflections and power loss. The bandwidth (BW) is defined as the frequency range over which the reflection coefficient remains below a specified threshold, typically |Γ| ≤ 0.5 (corresponding to a VSWR ≤ 3:1).

Quality Factor and Bandwidth Relationship

The quality factor (Q) of a matching network determines its bandwidth. For a series or parallel RLC network, the fractional bandwidth is inversely proportional to Q:

$$ \text{BW} = \frac{f_0}{Q} $$

For a double-tuned matching network, the relationship becomes:

$$ Q = \frac{f_0}{\Delta f} = \frac{1}{2} \sqrt{\frac{Z_{\text{high}}}{Z_{\text{low}}}} $$

where Zhigh and Zlow are the higher and lower impedances being matched. A high Q results in a narrow bandwidth, which is undesirable for wideband applications.

Broadband Matching Techniques

To achieve wideband impedance matching, engineers employ:

For example, a Chebyshev polynomial-based matching network provides equiripple response, ensuring minimal reflection across the desired band.

Practical Bandwidth Limitations

In real-world applications, parasitic capacitance and inductance constrain achievable bandwidth. For instance, a lumped-element L-network may exhibit a usable bandwidth of only 5–10% of f₀, while distributed designs (e.g., microstrip stubs) can achieve 20–30%.

Frequency (f) |Γ| Center Frequency (f₀) Bandwidth (Δf)

Case Study: Antenna Matching Network

A 50 Ω to 75 Ω matching network for a dipole antenna operating at 100 MHz with a 10% bandwidth requires:

$$ Q = \frac{1}{2} \sqrt{\frac{75}{50}}} \approx 0.612 $$
$$ \Delta f = \frac{f_0}{Q} = \frac{100 \text{ MHz}}{0.612} \approx 163 \text{ MHz} $$

This exceeds the desired 10 MHz bandwidth, indicating the need for a multi-section design or a low-pass filter to suppress harmonics.

Frequency Response and Bandwidth of Impedance Matching Network A frequency response curve showing the reflection coefficient (|Γ|) versus frequency, with center frequency (f₀), bandwidth (Δf), and |Γ| ≤ 0.5 threshold marked. Frequency (f) |Γ| f₀ Δf |Γ| ≤ 0.5 BW = f₀/Q
Diagram Description: The section includes a frequency response curve and bandwidth visualization, which are inherently graphical concepts.

Frequency Response and Bandwidth

The frequency response of an impedance matching network defines its ability to efficiently transfer power across a range of frequencies. At the center frequency f₀, the network achieves perfect matching, but deviations from this frequency introduce reflections and power loss. The bandwidth (BW) is defined as the frequency range over which the reflection coefficient remains below a specified threshold, typically |Γ| ≤ 0.5 (corresponding to a VSWR ≤ 3:1).

Quality Factor and Bandwidth Relationship

The quality factor (Q) of a matching network determines its bandwidth. For a series or parallel RLC network, the fractional bandwidth is inversely proportional to Q:

$$ \text{BW} = \frac{f_0}{Q} $$

For a double-tuned matching network, the relationship becomes:

$$ Q = \frac{f_0}{\Delta f} = \frac{1}{2} \sqrt{\frac{Z_{\text{high}}}{Z_{\text{low}}}} $$

where Zhigh and Zlow are the higher and lower impedances being matched. A high Q results in a narrow bandwidth, which is undesirable for wideband applications.

Broadband Matching Techniques

To achieve wideband impedance matching, engineers employ:

For example, a Chebyshev polynomial-based matching network provides equiripple response, ensuring minimal reflection across the desired band.

Practical Bandwidth Limitations

In real-world applications, parasitic capacitance and inductance constrain achievable bandwidth. For instance, a lumped-element L-network may exhibit a usable bandwidth of only 5–10% of f₀, while distributed designs (e.g., microstrip stubs) can achieve 20–30%.

Frequency (f) |Γ| Center Frequency (f₀) Bandwidth (Δf)

Case Study: Antenna Matching Network

A 50 Ω to 75 Ω matching network for a dipole antenna operating at 100 MHz with a 10% bandwidth requires:

$$ Q = \frac{1}{2} \sqrt{\frac{75}{50}}} \approx 0.612 $$
$$ \Delta f = \frac{f_0}{Q} = \frac{100 \text{ MHz}}{0.612} \approx 163 \text{ MHz} $$

This exceeds the desired 10 MHz bandwidth, indicating the need for a multi-section design or a low-pass filter to suppress harmonics.

Frequency Response and Bandwidth of Impedance Matching Network A frequency response curve showing the reflection coefficient (|Γ|) versus frequency, with center frequency (f₀), bandwidth (Δf), and |Γ| ≤ 0.5 threshold marked. Frequency (f) |Γ| f₀ Δf |Γ| ≤ 0.5 BW = f₀/Q
Diagram Description: The section includes a frequency response curve and bandwidth visualization, which are inherently graphical concepts.

4.2 Component Selection and Tolerances

Impact of Component Tolerances on Network Performance

The quality factor (Q) of an impedance matching network is highly sensitive to component tolerances. For a simple L-section matching network, the loaded Q is given by:

$$ Q = \frac{1}{2} \sqrt{\frac{R_{\text{high}}}{R_{\text{low}}} $$

Where Rhigh and Rlow are the higher and lower resistances being matched. A 5% tolerance in inductance or capacitance can lead to a 10-15% deviation in the achieved Q, resulting in suboptimal power transfer or increased reflections. For narrowband applications, this necessitates tighter component tolerances (<1%) or active tuning mechanisms.

Selection Criteria for Inductors and Capacitors

The self-resonant frequency (SRF) of components must exceed the operating frequency to avoid parasitic effects. For inductors, the SRF is:

$$ \text{SRF} = \frac{1}{2\pi \sqrt{L C_{\text{parasitic}}}} $$

Key selection parameters include:

Parasitic Effects and Mitigation

Parasitic capacitance in inductors and parasitic inductance in capacitors (Llead) modify the effective impedance. For a capacitor with lead inductance, the impedance becomes:

$$ Z_{\text{eff}} = \frac{1}{j\omega C} + j\omega L_{\text{lead}} $$

Surface-mount devices (SMDs) minimize these effects by reducing lead lengths. For frequencies above 1 GHz, component footprints must be modeled as distributed elements.

Temperature and Aging Considerations

Temperature coefficients (TC) of components can shift the matching frequency. For example, a capacitor with TC = ±30 ppm/°C will drift by 0.3% over a 100°C range. Aging in ceramic capacitors (e.g., X7R) can further degrade performance by 2-5% over time. Military-grade components or oven-controlled oscillators may be required for critical applications.

Practical Trade-offs in Component Selection

High-Q components (e.g., silver mica capacitors) reduce losses but increase cost. For broadband matching, lower-Q components with wider tolerances may suffice. Monte Carlo simulations are often employed to assess yield under tolerance variations. A typical design flow involves:

Frequency Response Under Component Tolerances Nominal ±5% Tolerance
Frequency Response Deviation Due to Component Tolerances A waveform plot comparing nominal and tolerance-affected frequency response curves, showing amplitude deviation across frequencies. Frequency (Hz) Amplitude (dB) 1k 10k 100k 1M -20 -10 0 10 20 Nominal Response ±5% Tolerance Response Frequency Response Deviation Due to Component Tolerances
Diagram Description: The section discusses the impact of component tolerances on frequency response, which is inherently visual and would benefit from a labeled comparison of nominal vs. tolerance-affected performance.

4.2 Component Selection and Tolerances

Impact of Component Tolerances on Network Performance

The quality factor (Q) of an impedance matching network is highly sensitive to component tolerances. For a simple L-section matching network, the loaded Q is given by:

$$ Q = \frac{1}{2} \sqrt{\frac{R_{\text{high}}}{R_{\text{low}}} $$

Where Rhigh and Rlow are the higher and lower resistances being matched. A 5% tolerance in inductance or capacitance can lead to a 10-15% deviation in the achieved Q, resulting in suboptimal power transfer or increased reflections. For narrowband applications, this necessitates tighter component tolerances (<1%) or active tuning mechanisms.

Selection Criteria for Inductors and Capacitors

The self-resonant frequency (SRF) of components must exceed the operating frequency to avoid parasitic effects. For inductors, the SRF is:

$$ \text{SRF} = \frac{1}{2\pi \sqrt{L C_{\text{parasitic}}}} $$

Key selection parameters include:

Parasitic Effects and Mitigation

Parasitic capacitance in inductors and parasitic inductance in capacitors (Llead) modify the effective impedance. For a capacitor with lead inductance, the impedance becomes:

$$ Z_{\text{eff}} = \frac{1}{j\omega C} + j\omega L_{\text{lead}} $$

Surface-mount devices (SMDs) minimize these effects by reducing lead lengths. For frequencies above 1 GHz, component footprints must be modeled as distributed elements.

Temperature and Aging Considerations

Temperature coefficients (TC) of components can shift the matching frequency. For example, a capacitor with TC = ±30 ppm/°C will drift by 0.3% over a 100°C range. Aging in ceramic capacitors (e.g., X7R) can further degrade performance by 2-5% over time. Military-grade components or oven-controlled oscillators may be required for critical applications.

Practical Trade-offs in Component Selection

High-Q components (e.g., silver mica capacitors) reduce losses but increase cost. For broadband matching, lower-Q components with wider tolerances may suffice. Monte Carlo simulations are often employed to assess yield under tolerance variations. A typical design flow involves:

Frequency Response Under Component Tolerances Nominal ±5% Tolerance
Frequency Response Deviation Due to Component Tolerances A waveform plot comparing nominal and tolerance-affected frequency response curves, showing amplitude deviation across frequencies. Frequency (Hz) Amplitude (dB) 1k 10k 100k 1M -20 -10 0 10 20 Nominal Response ±5% Tolerance Response Frequency Response Deviation Due to Component Tolerances
Diagram Description: The section discusses the impact of component tolerances on frequency response, which is inherently visual and would benefit from a labeled comparison of nominal vs. tolerance-affected performance.

4.3 Common Applications in RF and Microwave Systems

Antenna Feed Networks

Impedance matching is critical in antenna feed networks to maximize power transfer and minimize reflections. A mismatched antenna system results in standing waves, reducing radiation efficiency and potentially damaging transmitter components. For instance, a quarter-wave transformer is often employed to match the characteristic impedance of a transmission line (Z0) to the antenna's input impedance (ZL). The transformer's impedance (ZT) is given by:

$$ Z_T = \sqrt{Z_0 Z_L} $$

In phased-array antennas, impedance matching ensures uniform power distribution across elements, preventing beam distortion. Microstrip-based matching networks, such as tapered lines or stub tuners, are commonly used due to their compact form factor and ease of integration with planar circuits.

Amplifier Design

RF and microwave amplifiers require precise impedance matching at both input and output ports to achieve optimal gain, noise figure, and stability. The conjugate matching condition ensures maximum power transfer:

$$ \Gamma_{\text{in}} = \Gamma_{\text{S}}^*, \quad \Gamma_{\text{out}} = \Gamma_{\text{L}}^* $$

where Γin and Γout are the reflection coefficients of the amplifier, while ΓS and ΓL correspond to the source and load. Low-noise amplifiers (LNAs) often use LC matching networks to minimize noise by presenting the optimal source impedance (Zopt) to the transistor.

Filter and Diplexer Integration

Impedance matching networks are integral to RF filters and diplexers to ensure minimal insertion loss and maximum out-of-band rejection. A Chebyshev filter, for example, relies on impedance transformations to maintain ripple specifications. The normalized element values (gk) for a low-pass prototype are scaled to the system impedance (Z0) and cutoff frequency (ωc):

$$ L_k = \frac{g_k Z_0}{\omega_c}, \quad C_k = \frac{g_k}{\omega_c Z_0} $$

Diplexers use matching networks to isolate transmit and receive paths while maintaining impedance continuity across frequency bands. This is particularly vital in duplex communication systems like radar and cellular base stations.

Waveguide and Coaxial Transitions

Transition structures between waveguides and coaxial lines require broadband impedance matching to suppress higher-order modes and reduce return loss. A stepped impedance transformer or ridged waveguide is often employed to gradually transition between dissimilar impedances. For a multi-section transformer with N sections, the reflection coefficient (Γ) is minimized when:

$$ \Gamma(\theta) = e^{-jN\theta} \sum_{n=0}^{N} \Gamma_n e^{j2n\theta} $$

where θ is the electrical length of each section. This technique is widely used in satellite communications and high-power RF systems.

Power Dividers and Couplers

Wilkinson power dividers and directional couplers rely on impedance matching to achieve equal power splitting and high isolation. A two-way Wilkinson divider, for instance, uses λ/4 lines with Z0√2 impedance and a isolation resistor (R = 2Z0). The scattering matrix (S) for an ideal divider is:

$$ S = \frac{-j}{\sqrt{2}} \begin{bmatrix} 0 & 1 & 1 \\ 1 & 0 & 0 \\ 1 & 0 & 0 \end{bmatrix} $$

Modern designs incorporate multi-section matching to enhance bandwidth, critical for wideband applications like software-defined radios (SDRs).

Impedance Matching Network Applications in RF Systems Schematic diagram showing side-by-side comparison of quarter-wave transformer, microstrip stub tuner, stepped impedance transformer, and Wilkinson power divider with labeled components. Z₀ Z_T (λ/4) Z_L Quarter-wave Transformer Z₀ Z_L Stub (λ/4) Microstrip Stub Tuner Z₀ Z₁ Z_L Stepped Impedance Transformer Z₀ Z₀ Z₀ R=2Z₀ Wilkinson Power Divider Impedance Matching Network Applications in RF Systems
Diagram Description: The section describes multiple spatial and structural concepts like quarter-wave transformers, microstrip-based matching networks, and waveguide transitions that are highly visual.

4.3 Common Applications in RF and Microwave Systems

Antenna Feed Networks

Impedance matching is critical in antenna feed networks to maximize power transfer and minimize reflections. A mismatched antenna system results in standing waves, reducing radiation efficiency and potentially damaging transmitter components. For instance, a quarter-wave transformer is often employed to match the characteristic impedance of a transmission line (Z0) to the antenna's input impedance (ZL). The transformer's impedance (ZT) is given by:

$$ Z_T = \sqrt{Z_0 Z_L} $$

In phased-array antennas, impedance matching ensures uniform power distribution across elements, preventing beam distortion. Microstrip-based matching networks, such as tapered lines or stub tuners, are commonly used due to their compact form factor and ease of integration with planar circuits.

Amplifier Design

RF and microwave amplifiers require precise impedance matching at both input and output ports to achieve optimal gain, noise figure, and stability. The conjugate matching condition ensures maximum power transfer:

$$ \Gamma_{\text{in}} = \Gamma_{\text{S}}^*, \quad \Gamma_{\text{out}} = \Gamma_{\text{L}}^* $$

where Γin and Γout are the reflection coefficients of the amplifier, while ΓS and ΓL correspond to the source and load. Low-noise amplifiers (LNAs) often use LC matching networks to minimize noise by presenting the optimal source impedance (Zopt) to the transistor.

Filter and Diplexer Integration

Impedance matching networks are integral to RF filters and diplexers to ensure minimal insertion loss and maximum out-of-band rejection. A Chebyshev filter, for example, relies on impedance transformations to maintain ripple specifications. The normalized element values (gk) for a low-pass prototype are scaled to the system impedance (Z0) and cutoff frequency (ωc):

$$ L_k = \frac{g_k Z_0}{\omega_c}, \quad C_k = \frac{g_k}{\omega_c Z_0} $$

Diplexers use matching networks to isolate transmit and receive paths while maintaining impedance continuity across frequency bands. This is particularly vital in duplex communication systems like radar and cellular base stations.

Waveguide and Coaxial Transitions

Transition structures between waveguides and coaxial lines require broadband impedance matching to suppress higher-order modes and reduce return loss. A stepped impedance transformer or ridged waveguide is often employed to gradually transition between dissimilar impedances. For a multi-section transformer with N sections, the reflection coefficient (Γ) is minimized when:

$$ \Gamma(\theta) = e^{-jN\theta} \sum_{n=0}^{N} \Gamma_n e^{j2n\theta} $$

where θ is the electrical length of each section. This technique is widely used in satellite communications and high-power RF systems.

Power Dividers and Couplers

Wilkinson power dividers and directional couplers rely on impedance matching to achieve equal power splitting and high isolation. A two-way Wilkinson divider, for instance, uses λ/4 lines with Z0√2 impedance and a isolation resistor (R = 2Z0). The scattering matrix (S) for an ideal divider is:

$$ S = \frac{-j}{\sqrt{2}} \begin{bmatrix} 0 & 1 & 1 \\ 1 & 0 & 0 \\ 1 & 0 & 0 \end{bmatrix} $$

Modern designs incorporate multi-section matching to enhance bandwidth, critical for wideband applications like software-defined radios (SDRs).

Impedance Matching Network Applications in RF Systems Schematic diagram showing side-by-side comparison of quarter-wave transformer, microstrip stub tuner, stepped impedance transformer, and Wilkinson power divider with labeled components. Z₀ Z_T (λ/4) Z_L Quarter-wave Transformer Z₀ Z_L Stub (λ/4) Microstrip Stub Tuner Z₀ Z₁ Z_L Stepped Impedance Transformer Z₀ Z₀ Z₀ R=2Z₀ Wilkinson Power Divider Impedance Matching Network Applications in RF Systems
Diagram Description: The section describes multiple spatial and structural concepts like quarter-wave transformers, microstrip-based matching networks, and waveguide transitions that are highly visual.

5. Key Textbooks and Papers

5.1 Key Textbooks and Papers

5.1 Key Textbooks and Papers

5.2 Online Resources and Tools

5.2 Online Resources and Tools

5.3 Advanced Topics and Research Directions

5.3 Advanced Topics and Research Directions