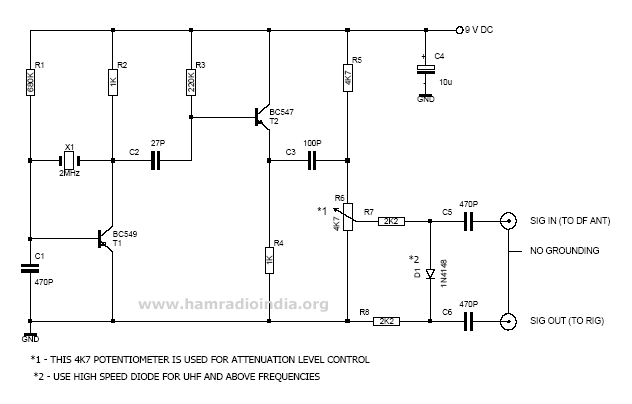
Passive Attenuators
1. Definition and Purpose of Attenuators
Definition and Purpose of Attenuators
A passive attenuator is an electronic circuit that reduces the amplitude or power of a signal without introducing significant distortion or noise. Unlike amplifiers, attenuators dissipate energy rather than providing gain, typically using resistive networks to achieve precise signal reduction. They are fundamental in RF, microwave, and audio systems where signal level control is critical.
Core Principles
Attenuators operate based on voltage division, power dissipation, and impedance matching. The attenuation (A) in decibels (dB) is defined as:
where \(V_{in}\) and \(V_{out}\) are input and output voltages, respectively. For power attenuation:
Key Characteristics
- Frequency Independence: Resistive attenuators maintain consistent performance across broad bandwidths.
- Impedance Matching: Designed to present matched input/output impedances (e.g., 50Ω or 75Ω) to prevent reflections.
- Power Handling: Determined by resistor power ratings and thermal dissipation.
Practical Applications
Attenuators are essential in:
- Test equipment calibration (e.g., reducing signal generator output)
- Receiver protection from high-power inputs
- Impedance matching in transmission lines
- Controlling signal levels in RF cascades
Mathematical Derivation: T-Pad Attenuator
For a symmetric T-pad attenuator with impedance \(Z_0\) and attenuation factor \(K\) (where \(K = 10^{A/20}\)):
Derived from solving simultaneous equations for input/output impedance matching and voltage division.
1.2 Key Characteristics: Attenuation and Impedance
Attenuation: Definition and Mathematical Representation
The primary function of a passive attenuator is to reduce signal amplitude by a known ratio, expressed in decibels (dB). The attenuation factor A is defined as the ratio of output power Pout to input power Pin:
For voltage or current signals, this translates to:
where Vout and Vin are the output and input voltages, respectively. A 3 dB attenuation corresponds to halving the power, while 6 dB reduces voltage by half.
Impedance Matching in Attenuators
Passive attenuators must maintain consistent impedance at both input and output ports to prevent signal reflections. The characteristic impedance Z0 (typically 50 Ω or 75 Ω) governs resistor network design. For a T-pad attenuator with impedance Z0 and attenuation factor K (linear scale), the resistor values are derived as:
For a π-pad configuration, the resistors follow:
Frequency Response and Limitations
While ideal attenuators are frequency-independent, parasitic capacitance and inductance introduce deviations at high frequencies. The cutoff frequency fc of an attenuator is approximated by:
where Lparasitic and Cparasitic arise from component packaging and PCB layout. For example, a 20 dB attenuator with 0.5 pF stray capacitance and 2 nH inductance has a theoretical bandwidth limit of ~1.6 GHz.
Power Handling and Thermal Considerations
The power rating of an attenuator is determined by its resistor network’s thermal dissipation capacity. For a given attenuation A (in dB) and maximum input power Pmax, the power dissipated Pdiss is:
For instance, a 10 dB attenuator handling 10 W input dissipates 9 W as heat, necessitating heat sinks or high-power resistors in RF applications.
Practical Design Example: 10 dB T-Pad Attenuator
For a 50 Ω system (Z0 = 50 Ω), a 10 dB attenuator (K = 1010/20 ≈ 3.162) requires:
These values ensure matched impedance while delivering the desired attenuation. Precision resistors (≤1% tolerance) are critical to minimize deviation from theoretical performance.
1.3 Passive vs. Active Attenuators
Fundamental Operating Principles
Passive attenuators rely solely on resistive networks to reduce signal amplitude without external power. The attenuation is frequency-independent within the network's operational limits, governed by the voltage division principle:
where R1 and R2 form the divider network. Active attenuators, in contrast, employ transistors or operational amplifiers with feedback loops to achieve programmable attenuation, introducing frequency-dependent gain stages:
Performance Trade-offs
- Linearity: Passive attenuators exhibit superior linearity (THD < 0.01%) due to the absence of active components. Active designs may introduce harmonic distortion from semiconductor nonlinearities.
- Bandwidth: Resistive networks in passive attenuators support multi-GHz bandwidths, while active versions are limited by gain-bandwidth product (GBW) of amplifiers.
- Noise Figure: Passive implementations maintain the system's inherent noise floor, whereas active stages add thermal and flicker noise.
Practical Implementation Considerations
In RF systems, passive attenuators dominate above 1 GHz due to their inherent broadband performance. The image below shows a π-network attenuator commonly used in 50Ω systems:
Active designs find use in baseband applications (DC-100MHz) where variable gain and impedance matching are critical. Modern IC-based solutions integrate digital control interfaces (e.g., SPI/I²C) for real-time attenuation adjustment.
Thermal and Power Handling
Power dissipation in passive attenuators follows:
requiring careful resistor selection for high-power applications. Active variants inherently limit power handling due to semiconductor junction constraints, typically below +30dBm.
Historical Context
The first waveguide attenuators (1930s) used carbon-loaded cards as passive loss elements. Active designs emerged with the proliferation of vacuum tube amplifiers in 1940s radar systems, later miniaturized using transistor technology.
2. Fixed Attenuators
Fixed Attenuators
Fixed attenuators are passive two-port networks designed to introduce a precise, unchanging reduction in signal amplitude. Unlike variable attenuators, their attenuation level is determined by a fixed resistor network, making them ideal for applications requiring consistent signal level control without adjustment. The most common configurations are the T-type, π-type, and bridged-T topologies, each offering distinct impedance-matching and power-handling characteristics.
Resistive Network Analysis
The fundamental operation of a fixed attenuator relies on resistive voltage division. For a matched system with characteristic impedance Z0, the attenuation A in decibels relates to the voltage ratio:
For a symmetric T-network, the resistor values R1 (series) and R2 (shunt) are derived from the desired attenuation factor K (where K = 10^{A/20}):
Impedance Matching and Power Dissipation
Fixed attenuators must maintain impedance matching to prevent reflections. For a 50 Ω system with 3 dB attenuation (K ≈ 1.414), the T-network resistors calculate to:
Power handling is limited by resistor tolerances and thermal dissipation. For a 1 W input at 3 dB attenuation, each R1 dissipates ~86 mW, while R2 handles ~357 mW. High-power designs use non-inductive wirewound or thick-film resistors.
Applications and Practical Considerations
- Test Equipment Calibration: Used in vector network analyzers to reduce signal levels without distorting phase.
- Receiver Protection: Limits input power to sensitive RF front-ends (e.g., LNAs) during overload.
- Impedance Bridging: Matches 75 Ω video lines to 50 Ω instrumentation with minimal reflections.
Temperature stability is critical; thin-film resistors with ±25 ppm/°C coefficients are preferred for precision applications. Connectorized attenuators (N-type, SMA) often specify VSWR < 1.2:1 up to 18 GHz.
Variable Attenuators
Variable attenuators provide adjustable signal reduction, essential in applications requiring dynamic control over signal amplitude, such as RF testing, audio engineering, and telecommunications. Unlike fixed attenuators, these devices allow continuous or step-wise adjustment of attenuation levels while maintaining impedance matching.
Continuously Variable Attenuators
Continuously variable attenuators (CVAs) use resistive elements whose values change smoothly, typically via mechanical or electronic means. A common implementation employs a potentiometer or voltage-controlled resistor to adjust the attenuation dynamically. The attenuation A in decibels (dB) for a simple resistive divider is given by:
where Vin and Vout are the input and output voltages, respectively. For a potentiometer-based attenuator, the output voltage varies linearly with the wiper position, but the logarithmic nature of decibels results in a nonlinear attenuation curve.
Step Variable Attenuators
Step variable attenuators provide discrete attenuation levels, often controlled by switches or relays. These are widely used in automated test systems where precise, repeatable attenuation is required. A typical design uses a cascaded network of fixed attenuators, with each stage contributing a specific dB value (e.g., 1 dB, 10 dB). The total attenuation is the sum of the engaged stages:
where Ai is the attenuation of the i-th stage. High-precision step attenuators achieve accuracy within ±0.1 dB by using thin-film resistors and low-insertion-loss switches.
Electronic Variable Attenuators
Electronic variable attenuators (EVAs) leverage semiconductor devices such as PIN diodes or FETs to adjust attenuation without mechanical parts. PIN diodes operate as voltage-controlled resistors at RF frequencies, with attenuation governed by the applied bias current. The relationship between bias current I and resistance R is approximately:
where K is a device-specific constant. FET-based attenuators exploit the channel resistance modulation via gate voltage, offering faster response times but limited linearity compared to PIN diodes.
Impedance Matching Considerations
Maintaining a constant impedance (typically 50 Ω or 75 Ω) across all attenuation settings is critical to prevent reflections. T-network or π-network topologies are common, with variable resistors adjusted symmetrically to preserve impedance. For a T-network:
where Z0 is the system impedance, and k is the voltage attenuation factor (10−A/20).
Applications and Trade-offs
- RF Testing: EVAs calibrate signal generators and spectrum analyzers, ensuring accurate power measurements.
- Broadcast Systems: Step attenuators manage signal levels in multi-channel transmitters.
- Trade-offs: CVAs offer smooth adjustment but suffer from mechanical wear; EVAs provide speed but may introduce harmonic distortion.
2.3 Step Attenuators
Step attenuators are precision passive devices designed to provide fixed, discrete levels of attenuation in signal paths. Unlike continuously variable attenuators, step attenuators offer well-defined attenuation steps, typically in logarithmic increments (e.g., 1 dB, 10 dB), making them indispensable in RF and microwave systems for signal level control, testing, and calibration.
Architecture and Switching Mechanisms
A step attenuator consists of multiple resistive networks, each corresponding to a specific attenuation value, and a switching mechanism to select between them. The most common topologies include:
- Binary-weighted ladder networks – Constructed using R-2R configurations for precise dB steps.
- Pi or T resistive networks – Switched in/out to achieve the desired attenuation.
- Monolithic IC implementations – Used in miniaturized high-frequency applications.
The switching mechanism can be electromechanical (relays), solid-state (PIN diodes, FETs), or MEMS-based, with trade-offs in speed, power handling, and linearity.
Mathematical Analysis of Attenuation Steps
For a step attenuator with N stages, the total attenuation Atotal in dB is the sum of the individual attenuations:
where Ai is the attenuation of the i-th stage. The impedance matching condition requires that each stage maintains the system characteristic impedance Z0 (typically 50 Ω or 75 Ω). For a Pi-network attenuator stage, the resistor values are given by:
Performance Characteristics
Key specifications include:
- Frequency flatness – Variation in attenuation across the operational bandwidth.
- Insertion loss – Minimum loss in the 0 dB state.
- VSWR – Voltage Standing Wave Ratio, indicating impedance matching.
- Switching speed – Critical for automated test equipment (ATE) applications.
Applications
Step attenuators are widely used in:
- RF test and measurement setups for dynamic range extension.
- Receiver protection by limiting input power levels.
- Radar systems for sensitivity adjustments.
- Telecommunication infrastructure for signal level optimization.
Practical Considerations
When selecting a step attenuator, engineers must consider:
- Power handling – Thermal limits of resistive elements and switches.
- Phase stability – Critical in phased-array and coherent systems.
- Repeatability – Essential for calibration purposes.
- Intermodulation distortion – Particularly in high-power applications.
Modern implementations often integrate digital control interfaces (e.g., SPI, USB) for automated adjustment in sophisticated test systems.
T-Pad and Pi-Pad Attenuators
T-Pad and Pi-Pad attenuators are symmetric resistive networks used to achieve precise signal attenuation while maintaining impedance matching. Unlike L-Pad attenuators, these configurations ensure consistent input and output impedance, making them ideal for high-frequency applications where reflections must be minimized.
Structure and Configuration
The T-Pad attenuator consists of three resistors arranged in a T-shaped topology. The series resistors (R1 and R2) are equal, while the shunt resistor (R3) bridges the midpoint to ground. The Pi-Pad attenuator, in contrast, uses a π-shaped configuration with two shunt resistors (R1 and R2) and one series resistor (R3). Both designs ensure that the input and output impedances (Z0) remain matched.
Design Equations
The resistor values for a T-Pad attenuator are derived from the desired attenuation factor (A) and characteristic impedance (Z0). The attenuation in decibels (N) relates to the voltage ratio as:
The series (R1 = R2) and shunt (R3) resistances are calculated as:
For a Pi-Pad attenuator, the shunt resistors (R1 = R2) and series resistor (R3) are determined by:
Practical Considerations
T-Pad attenuators are preferred in applications where the source and load impedances are well-defined and stable. Pi-Pad attenuators, however, are more suitable for variable loads due to their symmetrical impedance transformation. Both configurations are widely used in RF systems, audio engineering, and test equipment where precise signal control is critical.
In high-power applications, resistor power dissipation must be carefully evaluated. The power handling capability of each resistor is given by:
where I and V are the current and voltage across the respective resistors.
Comparison with Other Attenuator Types
Unlike L-Pad attenuators, T-Pad and Pi-Pad configurations maintain impedance matching in both directions, making them bidirectional. This property is essential in transmission lines and RF circuits where signal integrity must be preserved. The trade-off is increased component count and slightly higher insertion loss compared to simpler designs.
Bridged-T attenuators, a hybrid variant, offer improved bandwidth and are used in specialized applications requiring minimal phase distortion.
3. Resistor Network Configurations
3.1 Resistor Network Configurations
Passive attenuators rely on resistor networks to achieve precise signal reduction while maintaining impedance matching. The most common configurations include the L-pad, T-pad, and π-pad, each offering distinct advantages in terms of impedance matching, power handling, and frequency response.
L-Pad Attenuator
The L-pad consists of two resistors arranged in an "L" shape, providing a simple solution for impedance matching between source and load. The series resistor (R1) and shunt resistor (R2) are calculated based on the desired attenuation (A) and characteristic impedance (Z0). The design equations are derived from voltage division and impedance matching principles:
For example, a 6 dB attenuator in a 50 Ω system requires R1 ≈ 16.6 Ω and R2 ≈ 66.9 Ω. The L-pad is asymmetric, meaning reversing the input and output ports disrupts impedance matching.
T-Pad and π-Pad Attenuators
Symmetric configurations like the T-pad and π-pad maintain impedance matching regardless of signal direction. The T-pad uses three resistors (two series, one shunt), while the π-pad uses two shunt resistors and one series resistor. Their design equations ensure consistent input and output impedance:
These configurations are preferred in bidirectional systems, such as RF communication lines, where signal flow direction may vary. The π-pad is particularly efficient for high-power applications due to its distributed power dissipation.
Bridged-T Attenuator
A specialized variant, the bridged-T attenuator, combines series and shunt elements with a bridging resistor. This design is useful for fine-tuning attenuation while minimizing phase distortion. The bridged-T is often employed in precision measurement systems where signal integrity is critical.
The resistor values for a bridged-T attenuator are derived from:
This configuration provides improved return loss compared to traditional L-pads, making it suitable for high-frequency applications.
Practical Considerations
Resistor networks must account for parasitic effects, such as capacitance and inductance, which become significant at high frequencies. Thin-film resistors are often preferred for their low parasitic capacitance and stable temperature coefficients. Additionally, power dissipation must be evaluated to prevent resistor overheating in high-power scenarios.
For instance, in a 50 Ω system with 10 W input power and 6 dB attenuation, each resistor in a π-pad must handle at least 2.5 W to avoid thermal failure. Derating resistors to 50% of their rated power is a common practice for reliability.
3.2 Calculating Attenuation Values
The attenuation value of a passive attenuator is a fundamental parameter that quantifies the reduction in signal amplitude or power as it passes through the network. For advanced applications, precise calculation of attenuation is critical to ensure signal integrity, impedance matching, and minimal distortion.
Voltage and Power Attenuation
Attenuation can be expressed in terms of voltage ratio or power ratio, typically measured in decibels (dB). The voltage attenuation AV is given by:
where Vin and Vout are the input and output voltages, respectively. Similarly, power attenuation AP is defined as:
For a purely resistive attenuator, the relationship between voltage and power attenuation simplifies due to the quadratic dependence of power on voltage.
Resistive Divider Attenuation
A basic resistive voltage divider serves as the foundation for passive attenuators. The attenuation factor α for a simple two-resistor divider is:
where R1 and R2 form the divider network. To maintain impedance matching, the resistors must also satisfy:
where Z0 is the characteristic impedance of the system (typically 50 Ω or 75 Ω). Solving these equations yields the resistor values for a desired attenuation:
Pi and T-Attenuator Networks
For more precise control, Pi (π) and T-configuration attenuators are used. The resistor values for a symmetric T-attenuator are derived as follows:
For a Pi-attenuator, the resistances are:
These configurations ensure minimal reflection and consistent impedance across the frequency spectrum.
Practical Considerations
In real-world applications, parasitic capacitance and inductance affect high-frequency performance. For broadband attenuators, resistor selection must account for:
- Frequency-dependent losses due to skin effect and dielectric absorption.
- Thermal stability to prevent drift in attenuation values under varying power loads.
- Power handling to avoid resistor overheating at high signal levels.
Precision thin-film resistors are often preferred for their low parasitic effects and tight tolerances (±1% or better).
3.3 Impedance Matching Considerations
Impedance matching in passive attenuators is critical to minimize signal reflections and maximize power transfer. A mismatched system introduces standing waves, degrading signal integrity, particularly in high-frequency applications. The reflection coefficient (Γ) quantifies impedance mismatch and is given by:
where ZL is the load impedance and ZS is the source impedance. For perfect matching, Γ = 0, requiring ZL = ZS. In attenuator design, this imposes constraints on resistor networks.
L-Pad Attenuator Matching
The L-pad topology, consisting of series and shunt resistors, must satisfy both attenuation and impedance matching conditions. For a desired attenuation A (in dB) and system impedance Z0, the resistors R1 (series) and R2 (shunt) are calculated as:
These ensure the input and output impedances remain Z0 while providing the specified attenuation. Deviations from these values introduce mismatch errors, measurable via the voltage standing wave ratio (VSWR):
Frequency-Dependent Effects
At microwave frequencies, parasitic capacitance and inductance alter the attenuator’s impedance. For instance, a 50 Ω attenuator may exhibit reactive components, shifting its effective impedance. The normalized impedance z on a Smith chart reveals these deviations:
where r is the normalized resistance and x the normalized reactance. Compensation techniques, such as stub matching or tapered resistors, mitigate these effects.
Practical Case: 10 dB Pi-Attenuator
A 50 Ω Pi-attenuator with 10 dB loss requires resistors R1 = 71.15 Ω (shunt) and R2 = 96.25 Ω (series). The network’s S-parameters, simulated in SPICE, show S11 and S22 below −30 dB, confirming broadband matching. However, at 6 GHz, parasitic inductance raises S11 to −15 dB, necessitating layout optimizations.
3.4 Power Handling and Thermal Effects
Power Dissipation in Resistive Attenuators
In passive attenuators, power dissipation occurs entirely across the resistive network. For a voltage divider with resistors R1 and R2, the power P dissipated in each resistor depends on the input voltage Vin and the attenuation factor A. The total power handling capability is constrained by the weakest component, typically the smallest resistor.
Thermal Derating and Maximum Ratings
Resistors in attenuators exhibit thermal derating—their power handling capacity decreases as ambient temperature rises. Manufacturers specify a maximum surface temperature (e.g., 155°C for film resistors) and a derating curve. For example, a 1W resistor may only handle 0.5W at 100°C ambient. The thermal resistance θJA (junction-to-ambient) governs this relationship:
Thermal Runaway and Stability
In high-power applications, positive thermal coefficients can lead to thermal runaway. If a resistor's temperature coefficient (TCR) is too high, increased resistance causes further heating, creating a feedback loop. Stable attenuators use materials with low TCR (e.g., ±25 ppm/°C for precision metal-film resistors).
Practical Design Considerations
- Heat sinking: For attenuators handling >1W, thermal vias or metal-core PCBs dissipate heat.
- Resistor selection: Wirewound resistors handle higher power but introduce parasitic inductance.
- Transient overloads: Pulse withstand capability (e.g., 10× rated power for 5 ms) must be evaluated for RF applications.
Case Study: 30 dB Coaxial Attenuator
A 50Ω 30dB attenuator with 49.9kΩ (series) and 50.1Ω (shunt) resistors dissipates 2mW at 1V input. At 10W input, the shunt resistor must dissipate ≈10W—requiring a ceramic-composition resistor with >15W rating to account for derating.
4. Signal Level Adjustment in Audio Systems
Signal Level Adjustment in Audio Systems
Fundamentals of Passive Attenuation
Passive attenuators are resistive networks that reduce signal amplitude without active components. In audio systems, they are critical for impedance matching, preventing distortion, and ensuring optimal signal-to-noise ratio (SNR). The simplest form is a voltage divider:
where R1 and R2 form the divider network. For minimal signal degradation, the output impedance must be much lower than the load impedance (typically ≥10:1 ratio).
L-Pad vs. T-Pad Configurations
L-pad attenuators use two resistors to maintain constant impedance at both ports. The resistor values for a desired attenuation L (in dB) and system impedance Z are:
T-pads add a third resistor for bidirectional impedance matching, crucial in balanced audio lines. The center resistor R3 is calculated as:
Insertion Loss and Frequency Response
Non-ideal resistors introduce parasitic capacitance (Cp) and inductance (Lp), causing frequency-dependent attenuation. The −3 dB cutoff frequency for an L-pad is:
Carbon composition resistors (5–250 pF parasitic capacitance) are preferred over metal film for RF-sensitive applications due to lower Lp.
Practical Implementation
- Pro audio systems: Rotary L-pads in speaker crossovers (e.g., 8Ω, 100W) use wirewound resistors for power handling.
- Studio gear: Balanced T-pads with 0.1% tolerance resistors preserve SNR >110 dB in microphone preamps.
- DIY solutions: Logarithmic taper potentiometers (10kΩ–100kΩ) emulate L-pad behavior for variable attenuation.
Thermal Considerations
Power dissipation in resistors follows:
For a 20 dB attenuator handling +24 dBu (12.3Vrms) in 600Ω systems, R1 = 540Ω and R2 = 60Ω must dissipate 252 mW continuously. Metal oxide resistors (1W rating) are recommended for headroom.
Passive Attenuators in RF and Microwave Signal Conditioning
Fundamentals of Passive Attenuation
Passive attenuators are essential components in RF and microwave systems, designed to reduce signal power without introducing significant distortion or nonlinearity. Unlike active components, they rely solely on resistive networks to dissipate energy as heat. The attenuation A in decibels (dB) is defined as:
where Pin and Pout are the input and output power levels, respectively. For voltage signals, this translates to:
Resistive Attenuator Topologies
Three primary configurations dominate RF/microwave applications:
- T-pad attenuators: Symmetrical resistive network with series and shunt elements.
- π-pad attenuators: Dual configuration with shunt resistors at input/output and series resistor in between.
- Bridged-T attenuators: Hybrid design offering improved frequency response for precision applications.
The resistor values for a matched T-pad attenuator (50Ω system) are derived from:
Frequency-Dependent Considerations
At microwave frequencies (>1 GHz), parasitic effects dominate performance:
- Skin effect increases resistive losses in conductors
- Dielectric absorption in substrate materials
- Stray capacitance between components and ground planes
The cutoff frequency fc of an attenuator is determined by:
where Lpar and Cpar represent the equivalent parasitic inductance and capacitance.
Thermal Management in High-Power Applications
For continuous wave (CW) signals exceeding +30 dBm, power dissipation becomes critical. The maximum safe power handling is given by:
where ΔT is the allowable temperature rise and Rth is the thermal resistance. Thin-film resistors on beryllium oxide (BeO) substrates are commonly used for high-power applications due to their thermal conductivity of 250 W/m·K.
Practical Implementation Guidelines
When designing microwave attenuators:
- Use distributed elements for frequencies above 18 GHz
- Implement quarter-wave transformers for broadband matching
- Consider surface mount technology (SMT) for reduced parasitic effects
- Apply electromagnetic simulation (e.g., HFSS or ADS) to verify performance
The voltage standing wave ratio (VSWR) should be maintained below 1.5:1 across the operational bandwidth, calculated as:
where Γ is the reflection coefficient at the attenuator ports.
4.3 Test and Measurement Equipment
Characterizing Attenuator Performance
Accurate measurement of passive attenuators requires precision instrumentation to quantify insertion loss, return loss, and frequency response. A vector network analyzer (VNA) is the gold standard for characterizing RF/microwave attenuators, while audio and baseband applications may employ spectrum analyzers or dedicated impedance bridges. Key parameters include:
- Insertion Loss: Measured as the ratio of output to input power, typically in dB.
- Return Loss: Evaluates impedance matching at input and output ports.
- Flatness: Variation in attenuation across the specified frequency range.
- Power Handling: Maximum input power before nonlinear effects occur.
Network Analyzer Measurement Setup
For a two-port passive attenuator, the VNA measures S-parameters in a matched 50Ω (or 75Ω) system. The fundamental equations are:
where \( S_{21} \) represents forward transmission (attenuation) and \( S_{11} \) the input reflection coefficient. Calibration using thru-open-short-match (TOSM) standards eliminates systematic errors in the measurement path.
Time-Domain Reflectometry (TDR)
High-speed digital systems require TDR analysis to verify attenuator impedance characteristics. A step generator and sampling oscilloscope measure reflections caused by impedance mismatches:
where \( \rho \) is the reflection coefficient, \( Z_L \) the load impedance, and \( Z_0 \) the characteristic impedance. TDR resolutions below 10 ps reveal discontinuities in miniature surface-mount attenuators.
Power Meter Verification
For absolute power measurement traceability, a calibrated power meter with a thermistor or thermocouple sensor validates attenuator accuracy. The substitution method compares power readings with and without the device under test (DUT):
Uncertainty analysis must account for meter linearity, connector repeatability (typically ±0.01 dB), and thermal drift.
Environmental Stress Testing
Military/aerospace applications require temperature cycling (-55°C to +125°C) and vibration tests per MIL-STD-202. Monitoring attenuation drift with temperature reveals thermomechanical stability of thin-film resistors in precision attenuators:
where \( \alpha_T \) is the temperature coefficient of attenuation, typically <100 ppm/°C for high-reliability designs.
Intermodulation Distortion Analysis
Nonlinearities in power attenuators generate spurious tones measurable with a two-tone test. The third-order intercept point (TOI) characterizes dynamic range:
where \( P_{\text{IM3}} \) is the power level of third-order intermodulation products. High-power attenuators (>10W) require careful thermal management to maintain linearity.
5. Essential Textbooks on Attenuator Design
5.1 Essential Textbooks on Attenuator Design
- Passive Attenuators and Passive Attenuator Tutorial — Electronics Tutorial about the Passive Attenuator and Attenuator Design including T and Pi Attenuators used to control Impedance Mismatch ... Simple Passive Attenuator. Attenuators are the reverse of amplifiers in that they reduce gain with the resistive voltage divider circuit being a typical attenuator. ... 0.5: 1.0: 2.0: 3.0: 4.0: 5.0: 6.0 ...
- Download Electronic Communications by Dennis Roddy, John Coolen — Electronic Communications: Author: Dennis Roddy John Coolen: Language: English: ISBN: 9788177585582 / 9789332538030: Year: ... Passive Circuits 1.1 Introduction 1.2 Attenuator Pads The T-Attenuator The Pi-Attenuator The L-Attenuator 1.3 Series Tuned Circuit Impedance of a Series Tuned Circuit
- Experiment 8 - Attenuator | PDF | Transmission Line | Electrical ... — Experiment 8 - Attenuator - Free download as PDF File (.pdf), Text File (.txt) or read online for free. This document provides background information on attenuators, including: - Common types of fixed attenuators like T and π attenuators and their equivalent circuit models. - Describing an attenuator as a two-port network and defining important parameters like attenuation, input impedance ...
- Attenuator Design - sound-au.com — 5 2-Stage Attenuators. A 2-stage attenuator is just two 'simple' attenuators in series, separated by a buffer or gain stage. You need defined steps, typically 10dB for audio meters with a dB scale, or 1-2-5 sequence for oscilloscopes or other applications. The tables below show a 10dB 2-stage attenuator.
- RF Attenuator Linearization Circuits - SpringerLink — The LNA 50 Ω input impedance acts as a noiseless load (RL) for the passive attenuator. Assuming the 6 dB attenuation mode of operation, the variable resistance of R1 should equal 25 Ω. Comparing the NF for both configurations (a) and (b) using and for this gain setting results in a 7.7 dB NF for (a) and 5.8 dB NF for (b).
- 9.4: Terminations and Attenuators - Engineering LibreTexts — 9.4.2 Attenuators. An attenuator is a two-port network that reduces the amplitude of a signal and it does this by absorbing power and without distorting the signal. The input and output of the attenuator are both matched, so there are no reflections. An attenuator may be fixed, continuously variable, or discretely variable.
- Chapter 5.5.1: Capacitance Attenuator - MIT OpenCourseWare — Chapter 5.5.1: Capacitance Attenuator. Download video; Course Info Instructors Hermann A. Haus; James R. Melcher; Markus Zahn; Manuel L. Silva; Departments ... menu_book Online Textbook. theaters Lecture Videos. assignment_turned_in Problem Sets with Solutions. Download Course.
- NOOB DIY Attenuators - MOD WIGGLER — The Doepfer DIY page is a good shout - a simple attenuator is the first thing in section 2. To explain what's going on: in the case of passive attenuation, you're using the pot as a voltage divider. By moving the wiper, the resistance on either side of the divider alters, and you take the division out the middle pin.
- PDF ELECTRONICS ENGINEERS' HANDBOOK - dandelon.com — ELECTRONICS ENGINEERS' HANDBOOK Donald Christiansen Editor m chief President and principal, Informatica; Fellow, the Institute of Electrical and Electronics Engineers; Fellow, World Academy of Art and Science; Eminent
- PDF Colin˜May Passive Circuit Analysis with LTspice — This book is idiosyncratic in that it includes oddities not included in the general run of things, such as the Hamon voltage divider, the Murray loop test, the Elmoredelay and the Thiele-Small loudspeaker model. These are applications of passive circuits which were encountered during countless trawls through the Internet and seemed
5.2 Research Papers and Technical Articles
- (PDF) Phased Array Antennas for 5G and Beyond: Innovations and ... — 2018. This paper presents a new implementation of the beam-steerable two-dimensional phased antenna array for the forthcoming 5G networks. The antenna enables easy integration of phase shifters and other active electronics on a single PCB, low-loss feed network, low profile, and beam steering in both azimuth and elevation plane.
- Review on attenuation methods of low-frequency noise in passive ... — A database was constructed with a total of 142 articles published between 2016 and 2019 regarding low-frequency noise exposure and its effects on health. A total of 39 articles were analysed in depth.
- An S-K Band 6-Bit Digital Step Attenuator with Ultra Low ... - MDPI — This paper presents an ultra-wideband, low insertion loss, and high accuracy 6-bit digital step attenuator (DSA). To improve the accuracy of amplitude and phase shift of the attenuator, two innovative compensation structures are proposed in this paper: a series inductive compensation structure (SICS) designed to compensate for high frequency attenuation values and a small bit compensation ...
- A comprehensive review of improving power quality using active power ... — According to power circuit configurations and connection, this paper presents a comprehensive literature survey to classify and summary the existing APFs, analyze the application range, advantages, and disadvantages of various APFs, as well as the development prospects and trends of APF. To be specific, the paper is divided into eight parts.
- A MEMS Variable Optical Attenuator with Ultra-Low Wavelength ... - MDPI — A variable optical attenuator (VOA) is an important optical device for optical fiber communication and optical instrumentation [1,2].The main approaches for a VOA include: thermo-optically adjusted Mach-Zehnder interferometer (MZI) based on a planar lightwave circuit (PLC) [], optical fluid driven by a pump [4,5], liquid-core fiber driven by thermo-optical effect [], and MEMS technology.
- Design and Implementation of a Low-Power Biopotential Amplifier in 28 ... — Block diagram of the neural recorder. Each of the 49 pixels at each site contains a front-end circuit (A) with a biopotential amplifier, a sample-and-hold circuit, and a comparator.A ramp ADC (B) distributes a sawtooth waveform to all pixels, triggering the comparator when it intersects the amplified electrode signal.The readout circuit captures this transition, outputting the digitized value ...
- NDT.net - Open Access Archive and e-Library of Nondestructive Testing — The Largest Portal of Nondestructive Testing (NDT) Open Access Archive, Database, Conference Proceedings, Articles, News, Products and Services. Professional Networking, Exhibition, Forums
- An efficient FE approach for attenuation of acoustic radiation of thin ... — Some publications concerning acoustic radiation reduction by using shunted piezoelectric devices is briefly reviewed below. Ahmadian et al. [1] investigated a detailed analysis of the experimental results of sound transmission tests for three different test plates, namely an undamped plate, a plate with constrained layer damping, and a plate with electrically shunted piezoceramic materials.
- Study of Acoustic Emission Signal Noise Attenuation Based on ... — Acoustic emission (AE) technology, as a non-destructive testing methodology, is extensively utilized to monitor various materials' structural integrity. However, AE signals captured during experimental processes are often tainted with assorted noise factors that degrade the signal clarity and integrity, complicating precise analytical evaluations of the experimental outcomes. In response to ...
- Towards defect-free lattice structures in additive manufacturing: A ... — Additive manufacturing has transformed modern production by enabling the fabrication of complex and lightweight structures, particularly lattice geome…
5.3 Online Resources and Tutorials
- 5.5: Terminations and Attenuators - Engineering LibreTexts — An example of attenuator use in this situation is in a cable TV system, where it is critical that the integrity of the system is not compromised by a consumer disconnecting appliances from a cable outlet. Balanced and unbalanced resistive pads are shown in Figures 5.5.3 5.5. 3 and 5.5.4 5.5. 4 together with their design equations.
- Experiment 8 - Attenuator | PDF | Transmission Line | Electrical ... — This document provides background information on attenuators, including: - Common types of fixed attenuators like T and π attenuators and their equivalent circuit models. - Describing an attenuator as a two-port network and defining important parameters like attenuation, input impedance, and reflection coefficient. - Discussing characteristics of real resistors at high frequencies and their ...
- PDF The Art of Electronics — Perhaps of most interest to us in the context of circuit design; it includes the production of the voltages and currents needed in electronic circuit de-sign. Nearly all electronic circuits, from simple transistor and op-amp circuits up to elaborate digital and microproces-sor systems, require one or more sources of stable dc volt-age.
- PDF Mini-Circuits Programming Manual — Lab and test equipment setups for both manual and automated measurements. Control systems. Production test equipment. The attenuators can be used by anyone familiar with the basics of electronics measurements or electronic control systems.
- LOW PASS FILTERS - electronics tutorials — Low pass filter is two L networks It is simply two L networks added together like this to form a low pass pi network filter: Figure 2 - two L network low pass filters Where the reactances are C1 = C2 = 50 ohms each and L1a = L2a = 50 ohms each or a total of 100 ohms. The attenuation of this particular filter is given by the equation:
- Diode Clipping Circuits and Diode Clipper - Basic Electronics Tutorials ... — Diode Clipping Circuits The Diode Clipper, also known as a Diode Limiter, is a wave shaping circuit that takes an input waveform and clips or cuts off its top half, bottom half or both halves together.
- Class AB Amplifier - Basic Electronics Tutorials and Revision — As resistors are passive devices that convert electrical power into heat due to its power rating, the resistive biasing of a Class AB amplifier, either fixed or adjustable, can be very sensitive to changes in temperature.
- Attenuator unit iv | PDF - SlideShare — An attenuator is an electronic device that reduces the amplitude or power of a signal without distorting its waveform. It works in the opposite way of an amplifier by providing loss or gain less than 1. Attenuators are usually passive devices made from simple voltage divider networks using resistors. They are used to lower voltage, dissipate power, improve impedance matching, and enable safe ...
- PDF Lecture Notes for Analog Electronics - University of Oregon — You should convince yourself that this circuit attenuates low frequencies and \passes" (transmits with little attenuation) high frequencies, hence the term high-pass lter.