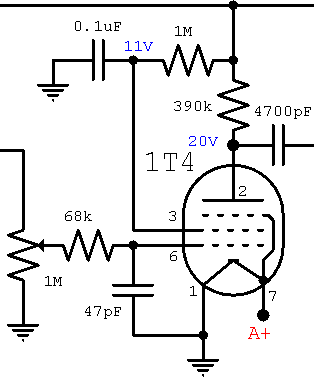
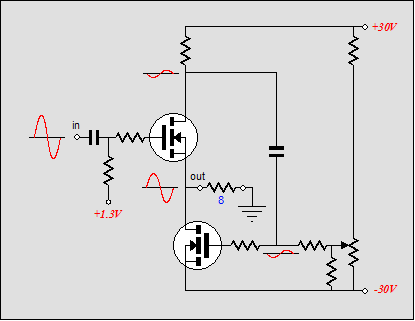
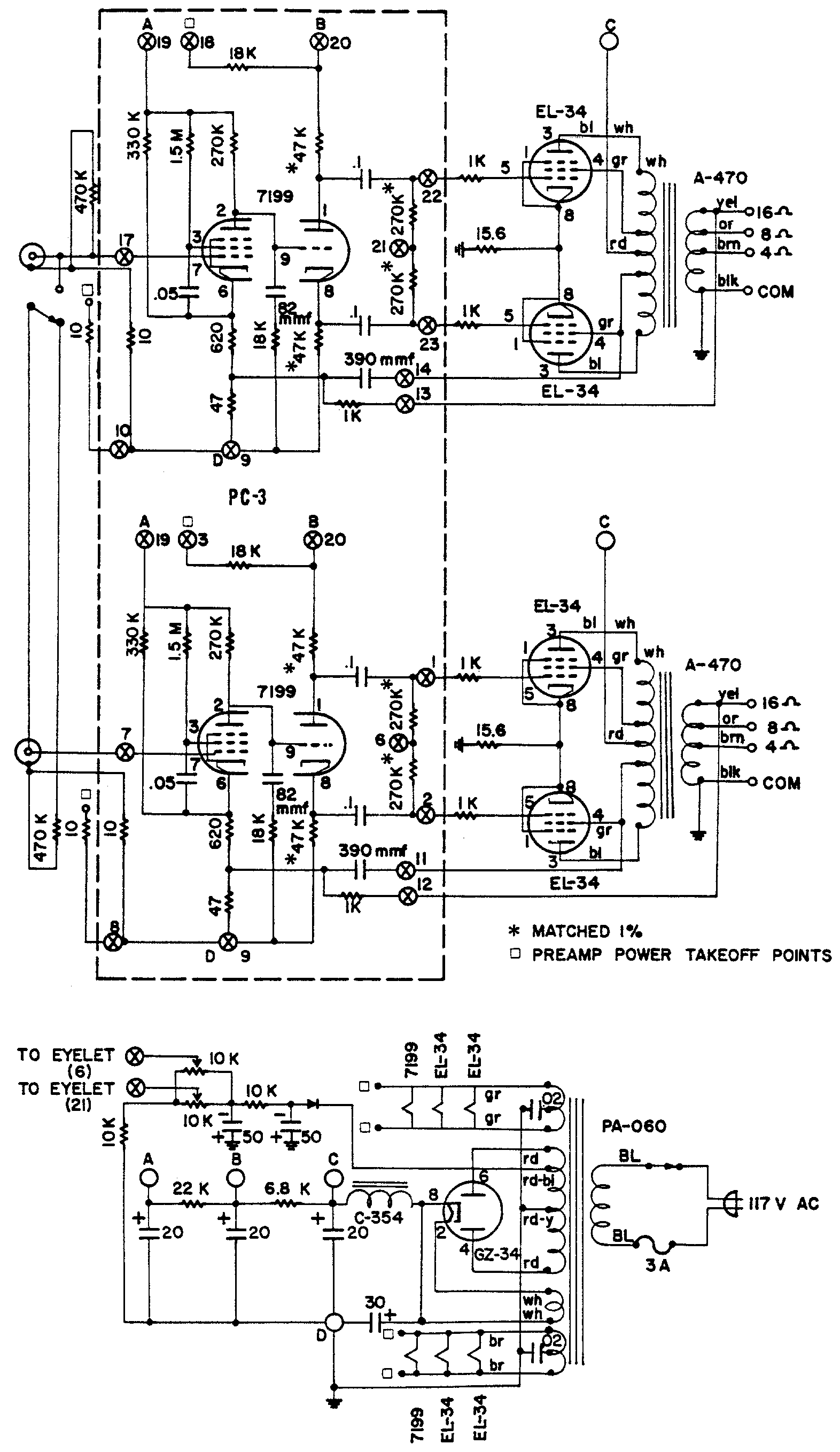
Phase Splitter

1. Definition and Purpose of a Phase Splitter

Definition and Purpose of a Phase Splitter

A phase splitter is an electronic circuit that takes a single input signal and produces two output signals of equal amplitude but opposite phase (i.e., 180° apart). This functionality is critical in applications requiring balanced differential signals, such as push-pull amplifiers, bridge circuits, and certain types of analog signal processing systems.

Fundamental Operation

The phase splitter operates by generating two complementary signals from a single input. Mathematically, if the input signal is Vin(t) = A sin(ωt), the outputs are:

$$ V_{out1}(t) = \frac{A}{2} \sin(\omega t) $$ $$ V_{out2}(t) = -\frac{A}{2} \sin(\omega t) $$

These outputs maintain the same frequency and amplitude but differ in phase by 180°. The negative sign in Vout2 indicates the phase inversion.

Key Characteristics

Common Implementations

Several circuit topologies achieve phase splitting, each with distinct advantages:

1. Differential Amplifier

A differential amplifier with a single-ended input naturally produces two anti-phase outputs. The gain and phase accuracy depend on the symmetry of the transistor or op-amp pair.

2. Transformer-Based Splitter

A center-tapped transformer inherently provides two out-of-phase signals. While highly linear, transformers introduce frequency-dependent phase shifts and are bulky for integrated designs.

3. Cathode/Emittor Follower Pair

Used in vacuum tube and bipolar transistor designs, this method employs a common-cathode (or common-emitter) stage followed by a cathode/emitter follower to generate the inverted and non-inverted signals, respectively.

Practical Applications

Phase splitters are indispensable in:

Mathematical Analysis of a Resistive Phase Splitter

Consider a simple resistive phase splitter using an op-amp:

$$ V_{out1} = V_{in} \left(1 + \frac{R_f}{R_i}\right) $$ $$ V_{out2} = -V_{in} \left(\frac{R_f}{R_i}\right) $$

For balanced outputs, the gains must satisfy:

$$ \left|1 + \frac{R_f}{R_i}\right| = \left|\frac{R_f}{R_i}\right| $$

This condition is met when Rf = Ri, yielding equal-magnitude outputs of opposite phase.

1.2 Key Applications in Electronics

Push-Pull Amplifiers

Phase splitters are fundamental in push-pull amplifier configurations, where they generate two anti-phase signals to drive complementary transistor pairs. The balanced output ensures minimal distortion by canceling even-order harmonics. A common implementation uses a long-tailed pair or a paraphase inverter to achieve the required 180° phase shift. The output impedance matching between the two phases is critical to maintain symmetry and prevent crossover distortion.

Modulation and Demodulation Circuits

In single-sideband (SSB) modulation, phase splitters enable the generation of quadrature signals necessary for phasing-type SSB modulators. The Hilbert transform, approximated using all-pass filter networks, relies on precise phase splitting to isolate the upper and lower sidebands. Similarly, in synchronous demodulation, phase splitters recover the in-phase (I) and quadrature (Q) components of a signal, essential for coherent detection in software-defined radios.

$$ V_{out1} = A \sin(\omega t), \quad V_{out2} = A \sin(\omega t + 180°) = -A \sin(\omega t) $$

Instrumentation and Measurement

Phase splitters are integral to lock-in amplifiers, where they provide reference signals for phase-sensitive detection. By splitting a reference oscillator signal into two paths with a controlled phase relationship, lock-in amplifiers can measure extremely small signals buried in noise. The phase accuracy, typically within ±0.1°, directly impacts the measurement sensitivity.

Audio Engineering

In stereo audio systems, phase splitters create balanced line-level signals to reduce noise pickup in long cable runs. The Schmitt trigger-based phase splitter is particularly effective for converting unbalanced signals to differential outputs while maintaining high common-mode rejection ratios (CMRR > 60 dB). This technique is widely used in professional audio interfaces and microphone preamplifiers.

Power Electronics

Three-phase inverters utilize phase splitters to generate 120°-shifted gate drive signals for MOSFET/IGBT bridges. The splitter’s bandwidth must exceed the switching frequency to prevent timing skew, which can lead to shoot-through currents. Digital implementations using phase-locked loops (PLLs) with fractional-N dividers offer sub-nanosecond phase resolution for high-frequency applications.

Case Study: Phase Splitter in RF Mixers

A double-balanced mixer requires precise anti-phase local oscillator (LO) signals to suppress carrier leakage. A transformer-based phase splitter with center-tapped secondary windings is often employed, providing inherent amplitude balance. For frequencies above 1 GHz, Lange couplers or rat-race hybrids replace traditional splitters, offering broadband performance with phase errors below 5° across octave bandwidths.

In-Phase Output Anti-Phase Output
Phase Splitter Output Waveforms and Applications Diagram showing input sine wave splitting into anti-phase outputs, quadrature signals for SSB modulation, and a simplified push-pull amplifier schematic. Input Waveform V_in Anti-phase Outputs V_out1 (0°) V_out2 (180°) Quadrature Signals (I/Q) I Component Q Component SSB Modulator Push-Pull Amplifier NPN PNP Complementary Transistors
Diagram Description: The section describes anti-phase signals, quadrature generation, and phase-sensitive detection, which are inherently visual concepts involving waveform relationships and spatial configurations.

1.3 Basic Operating Principles

A phase splitter is a circuit that generates two output signals of equal amplitude but opposite phase (180° apart) from a single input signal. This functionality is essential in push-pull amplifiers, balanced modulators, and differential signaling systems. The core principle relies on symmetrical signal inversion and amplification.

Mathematical Derivation of Phase Splitting

Consider an input signal Vin applied to a phase splitter. The outputs Vout1 (non-inverting) and Vout2 (inverting) must satisfy:

$$ V_{out1} = +A_v V_{in} $$ $$ V_{out2} = -A_v V_{in} $$

where Av is the voltage gain. For ideal operation, the magnitudes must match:

$$ |V_{out1}| = |V_{out2}| $$

Common Topologies

1. Differential Pair Phase Splitter

A long-tailed pair with matched transistors generates complementary outputs. The collector currents IC1 and IC2 are related by:

$$ I_{C1} = I_0 \left(1 + \tanh\left(\frac{V_{in}}{2V_T}\right)\right) $$ $$ I_{C2} = I_0 \left(1 - \tanh\left(\frac{V_{in}}{2V_T}\right)\right) $$

where VT is the thermal voltage (≈26 mV at 300 K). The output voltages at the collectors are 180° out of phase.

2. Cathodyne (Split-Load) Phase Splitter

This vacuum tube or FET-based circuit splits phase via a shared load resistor. The voltage division ensures:

$$ V_{out1} = V_{in} \frac{R_L}{R_L + r_p + (\mu + 1)R_k} $$ $$ V_{out2} = -V_{in} \frac{\mu R_k}{R_L + r_p + (\mu + 1)R_k} $$

where μ is amplification factor, rp is plate resistance, and Rk is the cathode resistor.

Practical Considerations

Real-World Applications

Phase splitters are critical in:

Phase Splitter Topologies Comparison Side-by-side comparison of Differential Pair and Cathodyne phase splitter circuits with labeled nodes and components. V_out1 V_out2 V_in R_L R_L R_k Q1 Q2 I_C1 I_C2 Differential Pair V_out1 V_in R_L R_k V_out2 r_p μ Cathodyne
Diagram Description: The section describes circuit topologies (Differential Pair and Cathodyne) with mathematical relationships that would be clearer with visual representation of the components and signal paths.

2. Transformer-Based Phase Splitters

Transformer-Based Phase Splitters

Transformer-based phase splitters leverage the inherent electromagnetic coupling of transformers to generate two or more output signals with precise phase relationships. These circuits are widely used in power electronics, audio amplification, and RF systems where phase accuracy and isolation are critical.

Operating Principle

A transformer with a center-tapped secondary winding naturally produces two outputs that are 180° out of phase. When an AC signal is applied to the primary winding, the induced voltages at the secondary winding's ends (relative to the center tap) exhibit equal magnitude but opposite polarity. The phase relationship arises from the winding direction and transformer action.

$$ V_{out1} = \frac{N_2}{N_1} V_{in} $$ $$ V_{out2} = -\frac{N_2}{N_1} V_{in} $$

Here, N1 and N2 represent the primary and secondary turns, respectively. The negative sign indicates the phase inversion.

Key Design Considerations

Transformer-based phase splitters must account for several critical parameters:

Practical Implementation

In push-pull audio amplifiers, transformer phase splitters ensure balanced drive signals for the output stage. The center-tapped secondary provides a reference point, while the outer terminals deliver anti-phase signals to the power transistors. Proper impedance matching is essential to minimize reflections and maintain signal fidelity.

$$ Z_{in} = \left(\frac{N_1}{N_2}\right)^2 Z_{load} $$

This equation ensures optimal power transfer by matching the reflected impedance to the source.

Advantages and Limitations

Advantages:

Limitations:

Historical Context

Early vacuum tube amplifiers relied heavily on transformer phase splitters due to their simplicity and effectiveness. Modern applications often replace them with active circuits in low-power scenarios, but they remain indispensable in high-voltage and high-current systems.

Modern Applications

Transformer phase splitters are still prevalent in:

Transformer Phase Splitter Operation A schematic diagram of a transformer phase splitter with a center-tapped secondary winding, showing the input AC signal and the resulting anti-phase output voltage waveforms. N1 N2 Center Tap Vout1 Vout2 AC Input Vout1 Vout2 180° phase shift
Diagram Description: The diagram would show the transformer's center-tapped secondary winding configuration and the resulting anti-phase voltage waveforms.

2.2 Transistor-Based Phase Splitters

Basic Operating Principle

A transistor-based phase splitter generates two output signals of equal amplitude but opposite phase (180° apart) from a single input signal. The most common configurations use bipolar junction transistors (BJTs) or field-effect transistors (FETs) in a common-emitter (CE) or common-source (CS) arrangement with a split-load resistor network. The phase inversion arises from the inherent property of CE/CS amplifiers, where the output at the collector/drain is inverted relative to the input, while the emitter/source follows the input signal in phase.

Common-Emitter Phase Splitter

The simplest BJT phase splitter consists of a single transistor in a CE configuration with two load resistors: one at the collector (RC) and one at the emitter (RE). For symmetric outputs, RC = RE. The voltage gain from base to collector is:

$$ A_{v,C} = -\frac{R_C}{r_e + R_E} $$

where re is the transistor's intrinsic emitter resistance (~25 mV/IE). The emitter follower gain is:

$$ A_{v,E} \approx \frac{R_E}{r_e + R_E} $$

When RC = RE, the magnitudes of Av,C and Av,E become nearly equal, producing balanced anti-phase outputs. However, the emitter output has a lower output impedance (Zout,E ≈ RE || (re + Rsource/β)) compared to the collector output (Zout,C ≈ RC).

Differential Pair Phase Splitter

For improved balance and linearity, a differential pair can be used. Here, the input drives one transistor's base, while the other base is grounded (or biased at a fixed voltage). The outputs taken from the two collectors provide inherently anti-phase signals. The differential gain is:

$$ A_{v,diff} = -g_m R_C $$

where gm is the transconductance. This configuration minimizes even-order harmonics and offers better common-mode rejection compared to single-transistor splitters.

Practical Considerations

Real-World Applications

Transistor phase splitters are fundamental in push-pull amplifiers, balanced modulators, and bridge output stages. For instance, the Long-Tailed Pair (a differential pair with a constant current source) is widely used in operational amplifier input stages and RF mixers where phase accuracy is critical. Modern implementations often replace discrete transistors with integrated op-amps or dedicated driver ICs for improved performance.

Common-Emitter Phase Splitter Circuit Schematic diagram of a common-emitter phase splitter circuit showing BJT transistor with input signal, resistors, power supply, and anti-phase outputs at collector and emitter. Q1 V_in R_C V_CC V_out (C) R_E V_out (E)
Diagram Description: The diagram would show the physical arrangement of a common-emitter phase splitter circuit with labeled resistors and transistor terminals, illustrating how the input signal splits into two anti-phase outputs.

2.3 Op-Amp-Based Phase Splitters

Operational amplifiers (op-amps) provide a precise and flexible means of generating phase-split signals, particularly in applications requiring high input impedance, low output impedance, and minimal phase error. Unlike passive phase splitters, op-amp-based designs mitigate loading effects and maintain signal integrity across a broad frequency range.

Basic Inverting and Non-Inverting Configuration

The simplest op-amp phase splitter employs an inverting amplifier alongside a unity-gain buffer. The inverting amplifier produces a 180° phase shift with gain determined by the feedback network, while the non-inverting path preserves the original phase. For a balanced output, the inverting stage is configured with a gain of −1.

$$ V_{out1} = -\left(\frac{R_f}{R_{in}}\right)V_{in} $$ $$ V_{out2} = V_{in} $$

where Rf and Rin are the feedback and input resistors, respectively. Matching Rf = Rin ensures equal magnitudes for both outputs.

Differential Amplifier as a Phase Splitter

A differential amplifier configuration inherently generates complementary outputs. By setting R1 = R2 and R3 = R4, the circuit yields:

$$ V_{out}^+ = \left(1 + \frac{R_2}{R_1}\right)\left(\frac{R_4}{R_3 + R_4}\right)V_{in} $$ $$ V_{out}^- = -\left(\frac{R_2}{R_1}\right)V_{in} $$

For symmetric outputs (Vout+ = −Vout−), the resistor ratios must satisfy R2/R1 = R4/R3.

Active Wien Bridge Phase Splitter

For applications requiring quadrature outputs (90° phase difference), an active Wien bridge network combines an op-amp integrator and differentiator. The integrator introduces a −90° phase shift, while the differentiator provides +90°:

$$ V_{out1} = \frac{-1}{RCs}V_{in} \quad \text{(Integrator)} $$ $$ V_{out2} = RCs \cdot V_{in} \quad \text{(Differentiator)} $$

where s = jω. At the center frequency (ω = 1/RC), the magnitudes are equal, and the phase difference is exactly 90°.

Practical Considerations

Applications

Op-amp phase splitters are critical in:

Op-Amp Phase Splitter Configurations Side-by-side comparison of three op-amp phase splitter configurations: inverting/non-inverting, differential amplifier, and Wien bridge, with labeled signal paths and phase annotations. Inverting/Non-Inverting V_in V_out1 V_out2 R_f R_in Differential Amplifier V_in1 V_in2 V_out1 V_out2 R1 R2 R3 R4 Wien Bridge V_in V_out1 V_out2 R_f R_in Integrator Differentiator Phase Inversion Differential Phase Bridge Balance
Diagram Description: The section describes multiple circuit configurations (inverting/non-inverting, differential amplifier, Wien bridge) where spatial relationships between components and signal paths are critical.

3. Circuit Configurations and Schematics

3.1 Circuit Configurations and Schematics

Common-Cathode (Differential) Phase Splitter

The common-cathode phase splitter, also known as a differential amplifier, utilizes two active devices (vacuum tubes or transistors) with a shared cathode (or emitter) resistor. The input signal is applied to one device, while the other provides an inverted output. The phase inversion arises due to the balanced current flow through the shared resistor, ensuring equal but opposite voltage swings at the anodes (or collectors).

$$ V_{out}^+ = -g_m R_L V_{in} $$ $$ V_{out}^- = +g_m R_L V_{in} $$

where gm is the transconductance and RL the load resistor. The circuit’s symmetry ensures minimal phase distortion, making it ideal for push-pull amplifier stages.

Paraphase (Split-Load) Phase Splitter

In a paraphase configuration, a single active device drives two outputs: one taken directly from the anode (or collector) and another from a voltage divider at the cathode (or emitter). The cathode follower action provides a non-inverted signal, while the anode output is inverted. The voltage divider ratio must precisely match the gain to ensure equal-amplitude outputs.

$$ \frac{R_1}{R_2} = \frac{\mu}{\mu + 1} $$

for vacuum tubes, where μ is the amplification factor. Transistor implementations replace μ with β (current gain).

Long-Tailed Pair Phase Splitter

This variant enhances common-mode rejection by using a high-impedance tail resistor (or current source) in the shared cathode/emitter path. The tail forces constant combined current, ensuring that input signals drive the devices differentially. The outputs remain 180° out of phase, with amplitude matching dependent on device symmetry.

$$ A_d = \frac{g_m R_L}{1 + g_m R_k} $$

where Ad is the differential gain and Rk the tail resistance. Modern implementations often replace Rk with a current mirror for improved stability.

Transformer-Based Phase Splitters

Center-tapped transformers inherently provide anti-phase signals due to their winding configuration. While less common in solid-state designs, they remain prevalent in tube amplifiers for impedance matching and galvanic isolation. The output phases are inherently balanced, though bandwidth limitations arise from parasitic inductance and capacitance.

Practical Considerations

Phase Splitter Circuit Configurations Side-by-side comparison of common-cathode, paraphase, and long-tailed pair phase splitter configurations with labeled components and signals. V_in V_out+ V_out- R_k Common Cathode V_in V_out+ V_out- Paraphase V_in V_out+ V_out- R_k Long-Tailed Pair g_m: Transconductance | μ/β: Amplification Factor R_L: Load Resistor (not shown for clarity)
Diagram Description: The section describes multiple circuit configurations with shared components and phase relationships that are inherently spatial and visual.

3.2 Signal Fidelity and Phase Accuracy

Fundamental Constraints in Phase Splitting

The primary challenge in phase splitting lies in maintaining signal fidelity while ensuring precise phase accuracy between the outputs. Any deviation from ideal behavior introduces distortion, compromising the integrity of the signal in applications such as quadrature modulation or differential signaling. The two critical parameters governing performance are:

Mathematical Analysis of Phase Error

For a differential phase splitter, the outputs Vout+ and Vout− should ideally satisfy:

$$ V_{out+} = -V_{out-} = A e^{j(\omega t + \phi)} $$

However, non-idealities introduce amplitude imbalance (ΔA) and phase error (Δϕ):

$$ V_{out+} = (A + \Delta A) e^{j(\omega t + \phi + \Delta \phi)} $$ $$ V_{out-} = -A e^{j(\omega t + \phi)} $$

The resulting phase imbalance is quantified as the deviation from the ideal 180° separation. For small errors (Δϕ ≪ 1 rad), the normalized phase error is:

$$ \epsilon_{\phi} \approx \frac{\Delta \phi}{\pi} \times 100\% $$

Sources of Degradation

1. Component Tolerances

Passive splitters (e.g., resistor-based or transformer-coupled) suffer from parasitic capacitance (Cp) and inductance (Lp), causing frequency-dependent phase shifts. For a resistive splitter with mismatched resistors R1 ≠ R2, the phase error scales as:

$$ \Delta \phi \approx \arctan\left(\frac{\omega (C_{p1} R_1 - C_{p2} R_2)}{1 + \omega^2 R_1 R_2 C_{p1} C_{p2}}\right) $$

2. Active Circuit Limitations

In op-amp or transistor-based splitters, finite bandwidth and slew rate induce phase lag. For a differential amplifier with pole frequency fp, the phase error at frequency f is:

$$ \Delta \phi = \tan^{-1}\left(\frac{f}{f_p}\right) $$

Mitigation Techniques

Case Study: High-Fidelity Splitting in RF Systems

In a 2.4 GHz quadrature splitter, a 5° phase error degrades image rejection by 20 dB. Modern designs use lumped-element Wilkinson dividers with λ/4 transmission lines to achieve Δϕ < 1° up to 6 GHz. Calibration algorithms further correct residual errors using DSP-based feedback.

Phase Splitter Error Visualization A combined vector diagram and synchronized oscilloscope-style waveform plot showing ideal vs. actual output vectors, time-domain waveforms for Vout+ and Vout-, phase deviation angle (Δϕ), and amplitude imbalance (ΔA). 180° Vout+ (ideal) Vout- (ideal) Vout+ (actual) Vout- (actual) Δϕ ΔA ΔA ωt Vout+ (ideal) Vout- (ideal) Vout+ (actual) Vout- (actual) ΔA Δϕ Phase Splitter Error Visualization
Diagram Description: The section involves vector relationships (phase/amplitude errors) and time-domain behavior of split signals, which are inherently visual concepts.

3.3 Load Considerations and Impedance Matching

Output Loading Effects

The performance of a phase splitter is critically dependent on the load impedance presented at its outputs. An unbalanced load disrupts the symmetry of the anti-phase signals, introducing amplitude and phase errors. For a differential output stage, the output impedance Zout of each leg must satisfy:

$$ Z_{out} \ll Z_L $$

where ZL is the load impedance. For a cathode-follower-based phase splitter, the output impedance is approximated by:

$$ Z_{out} = \frac{1}{g_m} \parallel r_p $$

where gm is the transconductance and rp is the plate resistance. Loading effects become pronounced when driving capacitive loads, as the high-frequency roll-off is asymmetrically affected.

Impedance Matching Techniques

To maintain signal integrity, the following matching strategies are employed:

For transformer-coupled designs, the turns ratio N must be selected to match the load impedance ZL to the optimal plate load Zopt:

$$ N = \sqrt{\frac{Z_{opt}}{Z_L}} $$

Case Study: Long-Tailed Pair Phase Splitter

In a long-tailed pair configuration, the tail current IT and load resistors RL determine the output balance. Mismatched loads cause differential gain asymmetry, quantified by:

$$ \Delta G = \frac{R_{L1} - R_{L2}}{R_{L1} + R_{L2}} $$

Modern implementations often use current mirrors to enforce symmetry, achieving load-independent phase splitting up to the mirror's bandwidth limit.

Phase Splitter Output Loading Effects Schematic of a phase splitter circuit with mismatched load impedances (ZL1 ≠ ZL2), showing asymmetric waveforms at outputs. ZL1 ZL2 Vout1 Vout2 Zout ΔG Vout1 Vout2
Diagram Description: The section discusses impedance relationships and asymmetrical loading effects, which are spatial concepts best shown with a labeled schematic of a phase splitter circuit under mismatched load conditions.

4. Common Design Challenges

4.1 Common Design Challenges

Phase Imbalance Due to Component Tolerances

A fundamental challenge in phase splitter design is maintaining precise 180° phase separation between outputs. Passive component mismatches—particularly in resistors and capacitors—introduce phase errors. For a differential amplifier-based splitter, even a 1% tolerance mismatch in collector or drain resistors (RC or RD) can degrade phase accuracy by several degrees. The phase error (Δϕ) scales with the mismatch ratio:

$$ \Delta \phi \approx \arctan\left(\frac{\Delta R}{R}\right) $$

Where ΔR is the resistance deviation. In high-frequency applications (>10 MHz), parasitic capacitances exacerbate this effect by introducing additional phase shifts.

Output Amplitude Mismatch

Achieving equal-amplitude antiphase signals requires symmetric gain paths. In transistor-based splitters (e.g., long-tailed pair), VBE mismatches or Early voltage effects cause amplitude asymmetry. For FET implementations, threshold voltage (Vth) variations produce similar imbalances. The normalized amplitude error (εA) is:

$$ \epsilon_A = \frac{|V_{\text{out+}}| - |V_{\text{out-}}|}{\sqrt{V_{\text{out+}}^2 + V_{\text{out-}}^2}} $$

Practical mitigation: Degeneration resistors reduce sensitivity to device mismatches but at the cost of reduced gain.

Power Supply Rejection Ratio (PSRR) Limitations

Phase splitters in single-supply configurations suffer from poor PSRR, as supply noise couples asymmetrically into the differential outputs. A 100 mV ripple on a 5V supply may induce >5° phase skew in uncompensated designs. The PSRR degradation factor (KPSRR) for a BJT differential pair is:

$$ K_{\text{PSRR}} = \frac{g_m \cdot r_o}{1 + g_m \cdot R_E} $$

Where gm is transconductance, ro the output impedance, and RE the emitter degeneration resistance.

Thermal Drift Effects

Temperature gradients across the splitter circuit cause differential thermal drift. In IC implementations, localized heating from adjacent components can create >0.1%/°C gain variation between phases. For precision applications, thermal symmetry must be maintained through layout techniques like common-centroid placement or dummy structures.

High-Frequency Phase Error

Above the circuit's dominant pole frequency, parasitic phase shifts accumulate. The cumulative phase error (ϕerr) at frequency f is:

$$ \phi_{\text{err}} = 180° - \sum_{n=1}^{N} \arctan\left(\frac{f}{f_{pn}}\right) + \sum_{m=1}^{M} \arctan\left(\frac{f}{f_{zm}}\right) $$

Where fpn and fzm are pole and zero frequencies. Above 100 MHz, transmission line effects in PCB traces further degrade performance unless matched-length routing is employed.

Load Sensitivity

Uneven loading between outputs disrupts phase balance. A 10% difference in load impedance can introduce 2–8° phase error depending on topology. Active buffering or impedance scaling networks (e.g., L-pads) are often necessary for driving mismatched loads.

Phase and Amplitude Mismatch in Differential Outputs Two sinusoidal waveforms (V_out+ and V_out-) showing phase error (Δϕ) and amplitude mismatch (ε_A), with a reference 180° marker. Time Amplitude 180° Reference Δϕ ε_A V_out+ V_out-
Diagram Description: The section discusses phase imbalance and amplitude mismatch, which are highly visual concepts best shown with labeled waveforms or vector diagrams.

4.2 Performance Optimization Techniques

Balanced Output Impedance

For optimal performance in a phase splitter, the output impedances of the inverting and non-inverting outputs must be matched. Mismatched impedances introduce phase and amplitude errors, degrading signal fidelity. Consider a differential amplifier phase splitter with emitter resistors RE. The output impedance at each terminal is given by:

$$ Z_{out} = R_C \parallel \left( r_o + R_E (1 + g_m r_o) \right) $$

where RC is the collector resistor, ro is the transistor's output resistance, and gm is the transconductance. To balance the outputs, RC and RE must be carefully selected such that Zout+ ≈ Zout-.

Minimizing Phase Error

Phase error arises from unequal propagation delays between the two outputs. In a long-tailed pair configuration, this can be mitigated by:

The phase error Δφ can be approximated as:

$$ \Delta \phi \approx \arctan\left( \frac{\omega \Delta C}{g_m} \right) $$

where ΔC is the capacitance mismatch and ω is the angular frequency.

Amplitude Matching

Amplitude imbalance is primarily caused by resistor tolerances and transistor mismatches. For a differential phase splitter, the gain mismatch ΔA between outputs is:

$$ \Delta A = \frac{A_+ - A_-}{A_+ + A_-} \approx \frac{\Delta R_C}{2 R_C} + \frac{\Delta g_m}{2 g_m} $$

To minimize ΔA:

Frequency Response Optimization

The bandwidth of a phase splitter is limited by the dominant pole formed by the load resistance and parasitic capacitance. The -3 dB frequency f3dB is:

$$ f_{3dB} = \frac{1}{2 \pi R_L C_{total}} $$

where Ctotal = Cout + Cstray + Cload. Techniques to extend bandwidth include:

Noise Reduction Strategies

Phase splitters in low-noise applications require careful attention to thermal and flicker noise. The input-referred noise voltage spectral density is:

$$ v_n^2 = 4kT \left( R_B + \frac{1}{2g_m} \right) + K_f \frac{I_B^a}{f} $$

where Kf and a are flicker noise coefficients. Noise can be reduced by:

Power Supply Rejection Ratio (PSRR) Enhancement

Poor PSRR allows supply noise to modulate the output phases. For a resistively-loaded differential pair, the PSRR is:

$$ PSRR \approx 20 \log_{10} \left( \frac{g_m R_C}{1 + g_m R_E} \right) $$

Improvements can be achieved by:

4.3 Debugging and Signal Integrity Issues

Common Signal Integrity Challenges in Phase Splitters

Phase splitters, particularly those based on differential amplifiers or transformer-coupled designs, are susceptible to signal integrity degradation due to mismatched impedances, parasitic capacitances, and inductive coupling. The primary issues include:

Quantifying Phase and Amplitude Errors

The phase error (Δφ) and amplitude imbalance (ΔA) between outputs can be modeled as:

$$ \Delta \phi = \tan^{-1}\left(\frac{Z_{diff}}{2Z_{cm}}\right) $$
$$ \Delta A = 20 \log_{10}\left(\frac{V_{out+}}{V_{out-}}\right) $$

where Zdiff and Zcm are the differential and common-mode impedances respectively. For transformer-based splitters, the phase error is dominated by the winding capacitance (Cw) and leakage inductance (Llk):

$$ \Delta \phi \approx \frac{\omega L_{lk}}{R_L} - \omega C_w R_s $$

Debugging Methodology

Time-domain analysis: Use differential probes to capture both outputs simultaneously, checking for:

Frequency-domain analysis: Network analyzer measurements reveal:

Layout Considerations for High-Fidelity Splitting

For PCB implementations, maintain:

$$ R_T = \sqrt{L_{trace}/C_{trace}} $$

Case Study: Debugging a 100MHz Differential Phase Splitter

A common failure mode involves degraded CMRR above 50MHz due to:

Mitigation strategies include:

Phase Splitter Signal Integrity Analysis A diagram showing dual output waveforms (Vout+ and Vout-) with phase difference markers, amplitude mismatch indicators, and parasitic components (Cw, Llk). Phase Splitter Signal Integrity Analysis 0V Time (ns) Voltage (V) Vout+ Vout- Δφ ΔA Rise Time Fall Time Cw Llk Zdiff Zcm Vout+ Vout-
Diagram Description: The section discusses phase imbalance, amplitude mismatch, and time-domain analysis which are highly visual concepts requiring waveform comparisons and spatial relationships.

5. Recommended Textbooks and Papers

5.1 Recommended Textbooks and Papers

5.2 Online Resources and Tutorials

5.3 Advanced Topics for Further Study