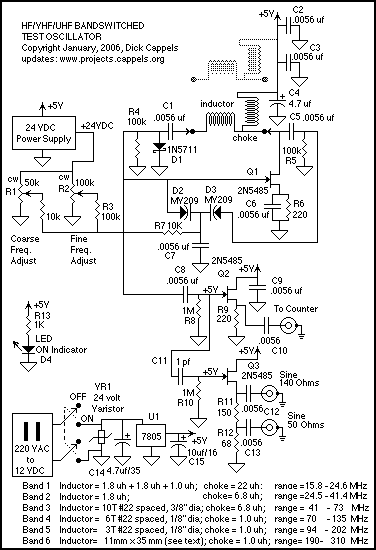
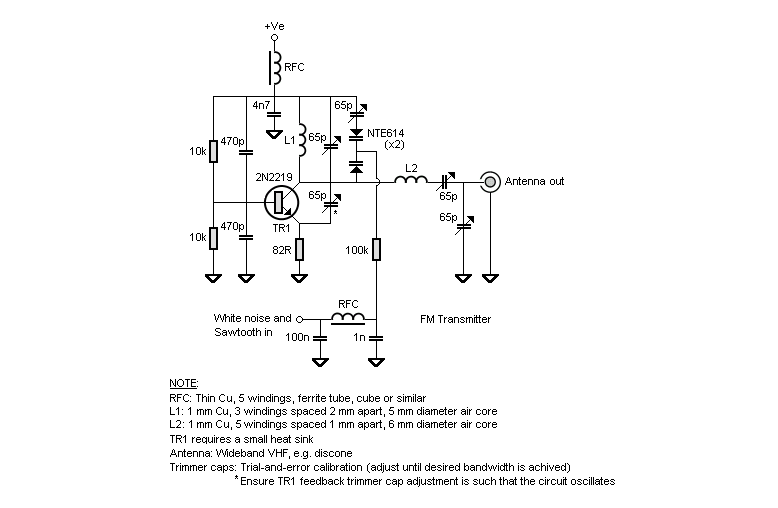
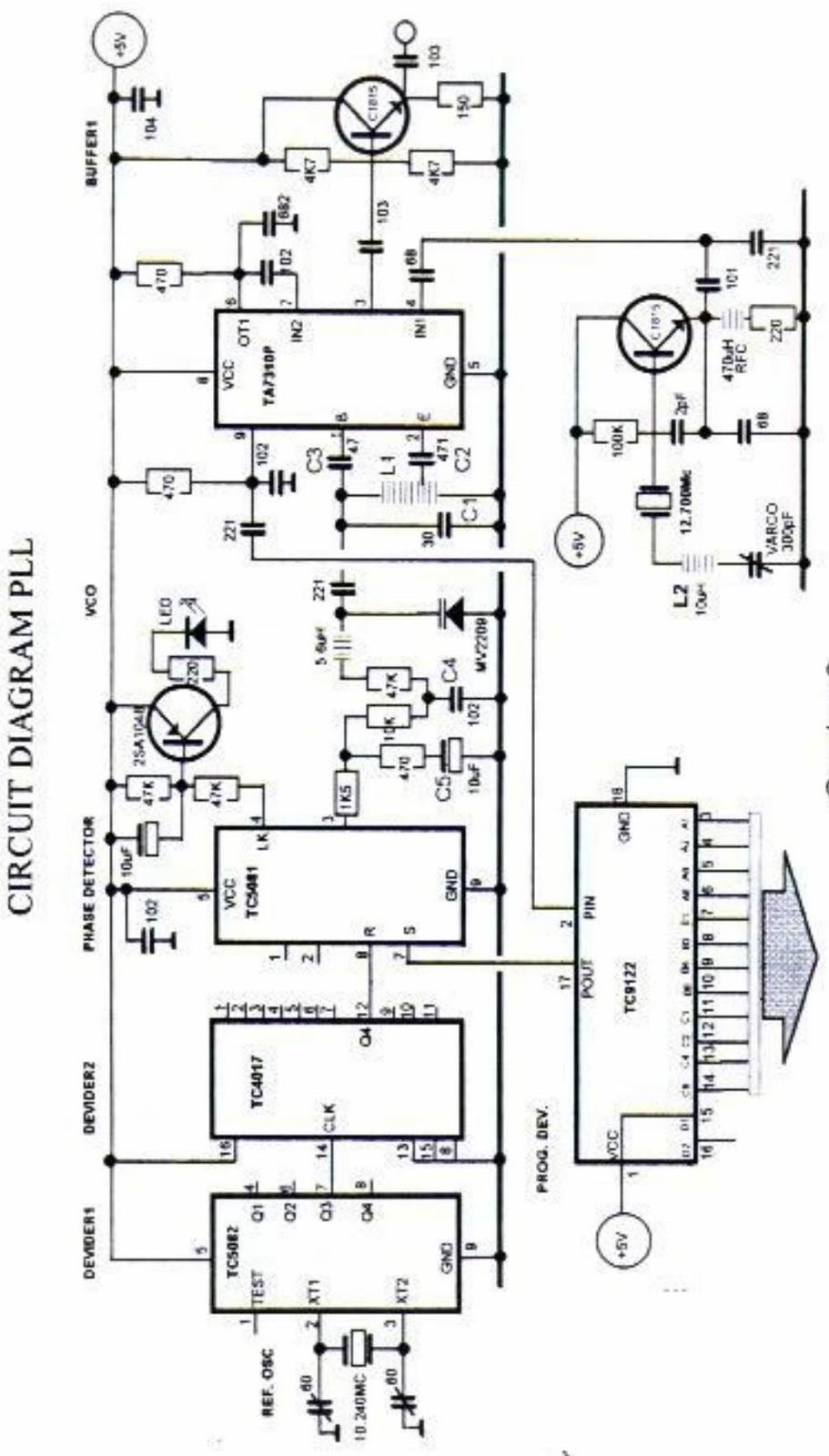
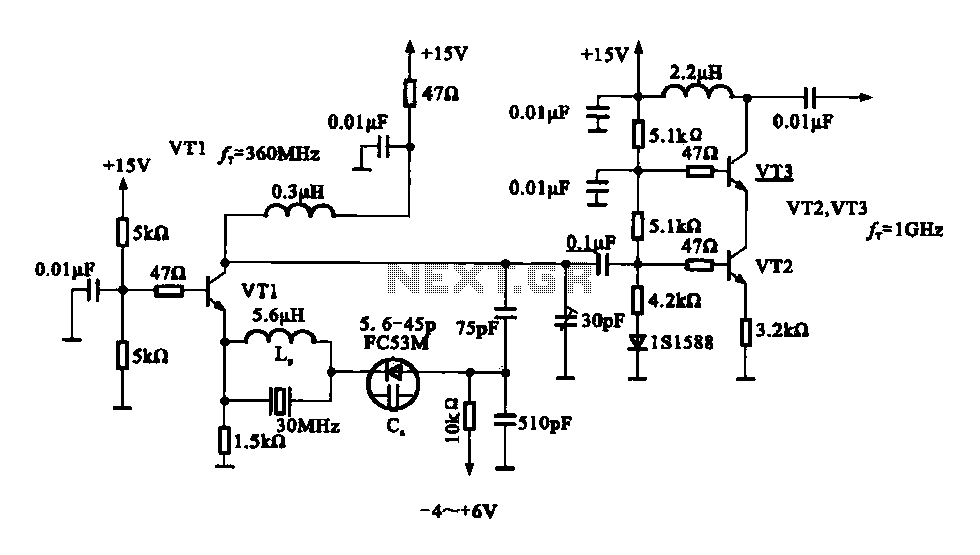
Varactor Diodes

1. Definition and Basic Principle

Varactor Diodes: Definition and Basic Principle

A varactor diode, also known as a varicap diode or voltage-variable capacitor diode, is a semiconductor device whose junction capacitance varies with the applied reverse bias voltage. Unlike conventional diodes optimized for rectification, varactors exploit the voltage-dependent width of the depletion region to function as electrically tunable capacitors.

Physical Operating Principle

The capacitance-voltage relationship arises from the modulation of the depletion region in a reverse-biased p-n junction. Under reverse bias, majority carriers are pulled away from the junction, widening the depletion region and reducing capacitance. The governing equation for the junction capacitance Cj is derived from Poisson's equation and the abrupt junction approximation:

$$ C_j(V) = \frac{C_{j0}}{\left(1 - \frac{V}{\phi_0}\right)^\gamma} $$

where:

Material Considerations

Modern varactors use hyperabrupt doping profiles (γ ≈ 0.5–2) to achieve steeper capacitance-voltage characteristics. Gallium arsenide (GaAs) varactors exhibit higher Q-factors than silicon at microwave frequencies due to lower series resistance. Heterojunction varactors (e.g., AlGaAs/GaAs) further enhance tuning range through bandgap engineering.

Practical Parameter Space

Key performance metrics include:

The capacitance-voltage characteristic exhibits nonlinearity, necessitating Taylor series analysis for small-signal applications:

$$ C_j(V) \approx C_{j0} \left[ 1 + \frac{\gamma V}{\phi_0} + \frac{\gamma(\gamma+1)}{2} \left(\frac{V}{\phi_0}\right)^2 \right] $$

This nonlinearity generates harmonic distortion, which becomes significant in wideband voltage-controlled oscillators (VCOs) and requires compensation in precision RF systems.

Varactor Diode Structure and Depletion Region Modulation Cross-sectional schematic of a varactor diode showing the P-N junction and depletion region under zero bias and reverse bias conditions. P-region N-region Depletion Region Cj0 Zero Bias (V=0) P-region N-region Depletion Region Vreverse γ Reverse Bias Variable Capacitance (Cj) Varactor Diode Structure and Depletion Region Modulation
Diagram Description: The diagram would show the physical structure of a varactor diode and how the depletion region width changes with reverse bias voltage.

1.2 Symbol and Circuit Representation

The varactor diode, also known as a voltage-variable capacitor or varicap diode, is represented in circuit schematics by a modified diode symbol that emphasizes its capacitive behavior. The standard symbol consists of a conventional diode shape with a capacitor-like addition at the cathode end, distinguishing it from rectifier or signal diodes.

Varactor

Key Symbol Features

Equivalent Circuit Model

Under reverse bias, a varactor's behavior is modeled by a voltage-dependent capacitance Cj(V) in parallel with a series resistance Rs (representing bulk semiconductor resistance) and a shunt resistance Rp (modeling leakage current). The small-signal equivalent circuit includes:

$$ C_j(V) = \frac{C_{j0}}{(1 - V/\phi)^n} $$

where Cj0 is the zero-bias capacitance, φ is the built-in potential (~0.7V for Si), V is the applied reverse bias, and n is the grading coefficient (0.5 for abrupt junctions, 0.33 for graded).

Cj(V) Rp Rs

Practical Implementation Notes

In RF circuits, varactors are often paired with inductors to form voltage-controlled oscillators (VCOs). The symbol's capacitor emphasis directly correlates with the diode's role in LC tank circuits, where the capacitance tuning range (Cmax/Cmin) determines the frequency agility. Modern SPICE models (e.g., BSIM4) incorporate these nonlinear effects through physics-based parameters like:

$$ Q = \frac{1}{2\pi f R_s C_j} $$

where Q defines the diode's quality factor at operating frequency f.

Varactor Diode Symbol and Equivalent Circuit A schematic diagram showing the varactor diode symbol on the left and its equivalent circuit model on the right, with labeled components. A K Cj(V) Rs Rp Cj(V) = C₀ / (1 - V/V₀)ⁿ Varactor Diode Symbol and Equivalent Circuit Symbol Equivalent Circuit
Diagram Description: The section includes both the varactor diode symbol and its equivalent circuit model, which are inherently visual concepts that require graphical representation to fully understand the spatial relationships and components.

1.3 Key Electrical Characteristics

Capacitance-Voltage Relationship

The fundamental property of a varactor diode is its voltage-dependent junction capacitance, governed by the abrupt or graded doping profile. For an abrupt junction, the capacitance C as a function of reverse bias VR is derived from Poisson's equation:

$$ C(V_R) = \frac{C_0}{(1 + V_R / \phi)^n} $$

where C0 is the zero-bias capacitance, φ is the built-in potential (~0.7V for Si), and n is the grading coefficient (0.5 for abrupt junctions, 0.33 for linearly graded). Hyperabrupt junctions exhibit n > 0.5, enabling wider tuning ranges in VCO applications.

Quality Factor (Q)

The quality factor quantifies energy storage efficiency at RF frequencies:

$$ Q = \frac{1}{2\pi f C R_s} $$

where Rs is the series resistance. High-Q (>100 at 1 GHz) is critical for low-phase-noise oscillators. Epitaxial silicon varactors achieve Q~200 at 2 GHz, while GaAs devices exceed 500 due to lower Rs.

Tuning Ratio and Linearity

The capacitance ratio Cmax/Cmin defines the tuning range. Commercial varactors typically achieve 2:1 to 10:1 ratios. For phase-locked loops, linear C-V characteristics (achieved through doping profile engineering) reduce distortion in the error signal.

Breakdown Voltage

The reverse breakdown voltage VBR limits the maximum tuning range. It's empirically related to doping concentration Nd:

$$ V_{BR} \approx 60\left(\frac{E_g}{1.1\text{eV}}\right)^{3/2}\left(\frac{N_d}{10^{16}\text{cm}^{-3}}\right)^{-3/4} $$

where Eg is the bandgap. Si varactors typically withstand 15-30V, while GaN devices exceed 100V for high-power RF applications.

Temperature Coefficients

The temperature dependence of capacitance arises from:

Compensation techniques include series/parallel combinations with opposite TC or monolithic integration with temperature-stable inductors.

Noise Mechanisms

Flicker noise (1/f) in varactors modulates oscillator phase noise. The noise power spectral density follows:

$$ S_v(f) = \frac{K_f}{C^2 f^\alpha} $$

where Kf is the flicker noise coefficient (10-22 to 10-24 F2/Hz for Si) and α ≈ 1. Low-noise designs use guard rings and optimized passivation layers to suppress surface states.

Varactor Diode C-V Characteristics Semi-log plot showing capacitance-voltage (C-V) curves for abrupt, graded, and hyperabrupt varactor diodes. Reverse Bias Voltage (VR) 0 φ 2φ 3φ Capacitance (C) 10C0 C0 0.1C0 0.01C0 Abrupt (n=0.5) Graded (n=0.33) Hyperabrupt (n>0.5) (φ, C0) (VR, C(VR))
Diagram Description: A diagram would visually show the nonlinear C-V relationship curves for abrupt, graded, and hyperabrupt junctions, which is central to understanding varactor behavior.

2. Voltage-Dependent Capacitance

2.1 Voltage-Dependent Capacitance

The capacitance of a varactor diode is fundamentally governed by the width of its depletion region, which varies with the applied reverse bias voltage. Unlike conventional diodes, varactors are engineered to maximize this voltage-capacitance relationship, making them essential in tuning and frequency-control applications.

Depletion Region and Junction Capacitance

Under reverse bias, the depletion region widens as the applied voltage increases, reducing the junction capacitance. This behavior is described by the abrupt junction approximation for uniformly doped diodes:

$$ C_j = \frac{C_{j0}}{\left(1 + \frac{V_R}{\phi_0}\right)^\gamma} $$

where:

Grading Coefficient and Doping Profile

The value of γ depends on the doping profile:

$$ \gamma = \frac{1}{2 + m} $$

where m describes the doping gradient. Hyperabrupt designs use m < 0 to invert the capacitance-voltage slope.

Practical Implications

The voltage-capacitance nonlinearity introduces harmonic distortion in RF systems. Designers mitigate this by:

Varactor Capacitance vs. Reverse Bias Abrupt (γ=0.5) Hyperabrupt (γ=1.5) VR Cj

Temperature Dependence

The built-in potential φ0 decreases with temperature (~2 mV/°C for silicon), causing capacitance drift. Compensation techniques include:

2.2 Depletion Region and Capacitance Variation

The capacitance of a varactor diode arises from the space-charge region (depletion region) formed at the p-n junction under reverse bias. As the reverse voltage increases, the depletion region widens, reducing the junction capacitance. This voltage-dependent behavior is governed by the principles of semiconductor physics and can be derived from Poisson's equation.

Depletion Width and Applied Voltage

The width of the depletion region (W) depends on the applied reverse bias (VR) and the built-in potential (Vbi). For an abrupt junction, the depletion width is given by:

$$ W = \sqrt{\frac{2\epsilon_s (V_{bi} + V_R)}{q} \left( \frac{1}{N_A} + \frac{1}{N_D} \right)} $$

where εs is the semiconductor permittivity, q is the electron charge, and NA and ND are the acceptor and donor concentrations, respectively.

Junction Capacitance Derivation

The junction capacitance (Cj) is modeled as a parallel-plate capacitor with the depletion region acting as the dielectric. The capacitance per unit area is inversely proportional to the depletion width:

$$ C_j = \frac{\epsilon_s}{W} $$

Substituting the expression for W yields the voltage-dependent capacitance:

$$ C_j(V_R) = \frac{C_{j0}}{\left(1 + \frac{V_R}{V_{bi}}\right)^{1/2}} $$

where Cj0 is the zero-bias junction capacitance. For hyperabrupt junctions, the doping profile is engineered to achieve a steeper capacitance-voltage relationship:

$$ C_j(V_R) = \frac{C_{j0}}{\left(1 + \frac{V_R}{V_{bi}}\right)^n} $$

where n ranges from 0.5 (abrupt junction) to >1 (hyperabrupt).

Practical Implications

In RF applications, varactors are operated in reverse bias to minimize conductive losses. The tuning ratio (Cmax/Cmin) is a critical figure of merit, determined by the doping profile and breakdown voltage. Modern varactors achieve tuning ratios exceeding 10:1 using epitaxial growth techniques to precisely control the doping gradient.

Varactor Diode C-V Characteristic Reverse Bias Voltage (V) Capacitance (pF)

Non-Ideal Effects

Varactor Diode C-V Characteristic A graph showing the relationship between reverse bias voltage and capacitance in a varactor diode, illustrating the C-V characteristic curve. VR Cj Reverse Bias Voltage (V) Capacitance (F) Cj0 Vbi Cmax Cmin
Diagram Description: The diagram would physically show the relationship between reverse bias voltage and capacitance in a varactor diode, illustrating the C-V characteristic curve.

2.3 Reverse Bias Operation

Varactor diodes operate exclusively under reverse bias, where the applied voltage increases the depletion region width, modulating the junction capacitance. Unlike forward bias, which induces high conduction current, reverse bias ensures minimal leakage current while enabling precise voltage-controlled capacitance tuning.

Depletion Region and Capacitance Modulation

Under reverse bias (VR > 0), the depletion region widens as majority carriers are pushed away from the junction. The resulting capacitance (Cj) follows the abrupt junction approximation for a one-sided step junction:

$$ C_j = \frac{C_{j0}}{\left(1 + \frac{V_R}{\phi_0}\right)^n} $$

where:

The nonlinear relationship between Cj and VR is critical for frequency tuning applications, such as voltage-controlled oscillators (VCOs).

Breakdown Voltage and Practical Limits

As reverse bias increases, the electric field across the depletion region intensifies. At the breakdown voltage (VBR), impact ionization triggers avalanche breakdown, causing uncontrolled current flow. Varactors are designed to operate well below VBR, with typical working ranges of 5–30 V for silicon and 15–60 V for GaAs devices.

Quality Factor (Q) and Series Resistance

The quality factor quantifies energy loss and is frequency-dependent:

$$ Q = \frac{1}{2\pi f C_j R_s} $$

where Rs is the series resistance (dominated by undepleted epitaxial layer resistance). High-Q varactors (Q > 100 at 1 GHz) are essential for low-phase-noise oscillators. Advanced fabrication techniques, such as hyperabrupt doping profiles, optimize Q by minimizing Rs.

Temperature Dependence

The reverse-bias capacitance exhibits temperature sensitivity due to:

Compensation techniques include using temperature-stable dopants (e.g., platinum in silicon) or differential pair configurations in circuit designs.

Applications in Tuning Circuits

Reverse-biased varactors enable:

Varactor C-V Curve (Reverse Bias) Capacitance (Cj) Reverse Voltage (VR)
Varactor Diode C-V Characteristic A graph showing the nonlinear relationship between reverse voltage (VR) and junction capacitance (Cj) in a varactor diode, illustrating how capacitance decreases with increasing reverse bias. VR (Reverse Voltage) Cj (Junction Capacitance) 0 φ0 VBR Cj0 Cj0 φ0 VBR Depletion Region
Diagram Description: The section explains the nonlinear relationship between reverse voltage and capacitance, which is best visualized with a C-V curve showing how capacitance decreases with increasing reverse bias.

3. Tuning Circuits in RF Applications

3.1 Tuning Circuits in RF Applications

Varactor diodes serve as voltage-controlled capacitors in radio frequency (RF) tuning circuits, enabling precise frequency adjustments without mechanical components. Their nonlinear capacitance-voltage (C-V) characteristic allows them to replace traditional variable capacitors in oscillators, filters, and phase-locked loops (PLLs). The capacitance C(v) of a varactor diode is governed by:

$$ C(v) = \frac{C_0}{(1 + v/\phi)^n} $$

where C0 is the zero-bias capacitance, v is the reverse bias voltage, φ is the built-in potential (~0.7 V for silicon), and n is the junction grading coefficient (0.5 for abrupt junctions, 0.33 for graded).

Resonant Frequency Tuning

In an LC tank circuit, the resonant frequency fr is modulated by varying the varactor’s capacitance:

$$ f_r = \frac{1}{2\pi\sqrt{LC(v)}} $$

For a series resonant circuit, the quality factor Q is critical for selectivity and is expressed as:

$$ Q = \frac{1}{R}\sqrt{\frac{L}{C(v)}} $$

where R represents the equivalent series resistance (ESR) of the diode and inductor. High-Q varactors (e.g., gallium arsenide (GaAs) types) minimize losses in RF front-ends.

Practical Implementation in VCOs

Voltage-controlled oscillators (VCOs) leverage varactors for frequency agility. A Colpitts oscillator with a varactor tuning network exemplifies this:

L1 Varactor BJT

The tuning range Δf is constrained by the varactor’s capacitance ratio Cmax/Cmin and the circuit’s parasitic capacitances. For a 1:3 capacitance ratio, the theoretical frequency ratio is:

$$ \frac{f_{max}}{f_{min}} = \sqrt{\frac{C_{max}}{C_{min}}} \approx 1.73 $$

Linearity and Distortion Mitigation

Varactor nonlinearity introduces harmonic distortion in wideband tuning. Predistortion techniques or back-to-back diode configurations improve linearity by canceling even-order harmonics. The effective capacitance of a back-to-back pair becomes:

$$ C_{eff}(v) = \frac{C(v)}{2} $$

Temperature stability is another critical factor; silicon varactors exhibit a temperature coefficient of ~100 ppm/°C, while GaAs variants offer better performance (~30 ppm/°C).

Case Study: FM Transmitter Tuning

In FM transmitters, varactors modulate the carrier frequency directly. A 100 MHz VCO with a varactor tuning sensitivity of 10 MHz/V requires a capacitance swing of 2.5 pF to 10 pF for ±75 kHz deviation, adhering to FCC bandwidth regulations.

Colpitts Oscillator with Varactor Tuning A schematic diagram of a Colpitts oscillator circuit featuring a varactor diode for tuning, with labeled components including inductor L1, capacitors C1 and C2, BJT transistor, and tuning voltage input V_tune. B C E L1 C1 C2 Varactor V_tune
Diagram Description: The section includes a Colpitts oscillator schematic with a varactor, which is a highly visual and spatial concept that would benefit from a detailed, labeled diagram.

3.2 Voltage-Controlled Oscillators (VCOs)

Fundamental Operating Principle

The core function of a voltage-controlled oscillator (VCO) is to generate an output signal whose frequency is a function of an applied control voltage. Varactor diodes serve as the key tuning element, leveraging their voltage-dependent capacitance to adjust the resonant frequency of an LC tank circuit. The relationship between the control voltage Vctrl and the output frequency fout is given by:

$$ f_{out} = \frac{1}{2\pi \sqrt{L C(V_{ctrl})}} $$

where L is the fixed inductance and C(Vctrl) is the varactor's junction capacitance, which varies nonlinearly with Vctrl.

Varactor Tuning Characteristics

The capacitance-voltage (C-V) relationship of a varactor diode is derived from the abrupt or hyperabrupt junction doping profiles. For an abrupt junction, the capacitance follows:

$$ C_j(V) = \frac{C_{j0}}{\left(1 + \frac{V_{ctrl}}{V_{bi}}\right)^\gamma} $$

where Cj0 is the zero-bias capacitance, Vbi is the built-in potential (~0.7 V for silicon), and γ is the grading coefficient (0.5 for abrupt junctions, 0.5–2 for hyperabrupt). Hyperabrupt varactors provide a more linear fout vs. Vctrl response, critical for wide-tuning-range VCOs.

Circuit Topologies

Colpitts VCO with Varactor Tuning

A common implementation uses a Colpitts oscillator with the varactor placed in parallel with the tank circuit. The feedback network (capacitors C1 and C2) sets the oscillation condition, while the varactor adjusts the resonant frequency. The effective tank capacitance becomes:

$$ C_{eq} = \left(\frac{1}{C_1} + \frac{1}{C_2} + \frac{1}{C_j(V_{ctrl})}\right)^{-1} $$

Negative Resistance VCOs

At microwave frequencies, negative-resistance oscillators (e.g., using Gunn diodes) paired with varactors enable tuning ranges exceeding 10 GHz. The Kurokawa stability criterion must be satisfied to ensure sustained oscillations.

Phase Noise Considerations

Phase noise in VCOs is dominated by the Leeson effect, modeled as:

$$ \mathcal{L}(\Delta f) = 10 \log \left[ \frac{2FkT}{P_{sig}} \left(1 + \frac{f_0^2}{4Q_L^2 \Delta f^2}\right) \left(1 + \frac{\Delta f_{1/f^3}}{|\Delta f|}\right) \right] $$

where QL is the loaded quality factor of the tank, and Δf1/f³ is the flicker noise corner frequency. Varactors contribute to phase noise through their Q-factor and flicker noise, necessitating high-Q hyperabrupt designs for low-noise applications.

Practical Design Example

A 2.4 GHz VCO for Wi-Fi 6E might use a SMV1234 hyperabrupt varactor (Cj0 = 2.2 pF, γ = 0.9) with a 5 nH inductor. For Vctrl = 0–5 V, the tuning range is:

$$ \Delta f = \frac{1}{2\pi \sqrt{5 \text{nH} \cdot 0.5 \text{pF}}} - \frac{1}{2\pi \sqrt{5 \text{nH} \cdot 2.2 \text{pF}}} \approx 1.8 \text{GHz} \text{ to } 3.6 \text{GHz} $$

Applications

Colpitts VCO with Varactor Tuning Schematic diagram of a Colpitts Voltage-Controlled Oscillator (VCO) using a varactor diode for tuning. The LC tank circuit includes inductor L, capacitors C1 and C2, and a varactor diode controlled by voltage Vctrl. Output signal fout is labeled. L C1 C2 Cj(Vctrl) Vctrl fout
Diagram Description: The section describes circuit topologies (Colpitts VCO) and tuning characteristics that involve spatial relationships between components and voltage-dependent capacitance changes.

3.3 Frequency Modulators and Phase Shifters

Varactor Diodes in Frequency Modulation

Varactor diodes, also known as varicap diodes, exploit voltage-dependent capacitance to enable frequency modulation (FM) in RF circuits. The junction capacitance \( C_j \) of a varactor diode varies with the applied reverse bias voltage \( V_R \), following the relationship:

$$ C_j = \frac{C_0}{(1 + V_R / \phi)^n} $$

where \( C_0 \) is the zero-bias capacitance, \( \phi \) is the built-in potential (~0.7V for silicon), and \( n \) is the doping profile exponent (typically 0.5 for abrupt junctions, 0.33 for graded junctions). In FM applications, the modulating signal \( V_m(t) \) is superimposed on the DC bias \( V_{DC} \), causing a time-varying capacitance:

$$ C_j(t) = \frac{C_0}{\left(1 + \frac{V_{DC} + V_m(t)}{\phi}\right)^n} $$

When integrated into an LC tank circuit, this modulates the resonant frequency \( f_r \):

$$ f_r(t) = \frac{1}{2\pi \sqrt{L C_j(t)}} $$

Phase Shifting with Varactors

Varactors enable precise phase control in RF systems by altering the propagation delay through a transmission line or resonator. In a reflection-type phase shifter, a varactor-terminated transmission line reflects signals with a phase shift \( \Delta \phi \) given by:

$$ \Delta \phi = 2 \arctan\left(\frac{2\pi f Z_0 C_j}{1 + (2\pi f Z_0 C_j)^2}\right) $$

where \( Z_0 \) is the characteristic impedance of the line. For small phase shifts (\( \Delta \phi \ll \pi/2 \)), this simplifies to:

$$ \Delta \phi \approx 4\pi f Z_0 C_j $$

Practical implementations often use quadrature hybrid couplers with varactor-loaded branches to achieve 0°–180° continuous phase tuning while maintaining impedance matching.

Nonlinearity and Distortion

The nonlinear \( C_j(V_R) \) characteristic introduces harmonic distortion in wideband FM systems. The third-order intermodulation distortion (IMD3) can be derived from a Taylor expansion of \( C_j \):

$$ \text{IMD3} \approx \frac{3}{8} \left(\frac{n(n+1)}{\phi^2}\right) V_{m,\text{rms}}^2 $$

This necessitates careful bias point selection and predistortion techniques in high-linearity applications like cellular base stations.

Practical Implementation Example

A common VCO (Voltage-Controlled Oscillator) topology using a Clapp oscillator with varactor tuning:

The tuning sensitivity \( K_V \) (Hz/V) is determined by:

$$ K_V = \frac{\partial f_r}{\partial V_R} = -\frac{n f_r}{2\phi} \left(1 + \frac{V_R}{\phi}\right)^{-(n+1)} $$

Modern implementations often use hyperabrupt junction varactors (n ≈ 0.75–1.5) for improved linearity and tuning range.

Varactor-Based VCO Circuit Schematic diagram of a varactor-based voltage-controlled oscillator (VCO) circuit, showing BJT active device, LC tank with varactor, feedback network, and DC bias components. Q L Cj VR Feedback Path VDC Output
Diagram Description: The section describes practical implementations like VCOs and phase shifters, which involve spatial relationships between components and signal flow.

4. Capacitance Ratio (Cmax/Cmin)

4.1 Capacitance Ratio (Cmax/Cmin)

The capacitance ratio (Cmax/Cmin) is a critical figure of merit for varactor diodes, defining their tuning range in voltage-controlled applications. This ratio quantifies the maximum achievable variation in junction capacitance as a function of applied reverse bias voltage. A higher ratio allows for broader frequency tuning in oscillators, filters, and phase-locked loops.

Mathematical Derivation of Capacitance Ratio

The junction capacitance Cj of a varactor diode follows the relationship:

$$ C_j(V) = \frac{C_{j0}}{\left(1 + \frac{V}{V_0}\right)^n} $$

where:

The maximum capacitance Cmax occurs at zero bias (V = 0), reducing to:

$$ C_{max} = C_{j0} $$

The minimum capacitance Cmin is achieved at the maximum rated reverse bias Vmax:

$$ C_{min} = \frac{C_{j0}}{\left(1 + \frac{V_{max}}{V_0}\right)^n} $$

Thus, the capacitance ratio simplifies to:

$$ \frac{C_{max}}{C_{min}} = \left(1 + \frac{V_{max}}{V_0}\right)^n $$

Practical Implications

For abrupt junction varactors (n = 0.5), a Vmax of 20 V and V0 of 0.7 V yields:

$$ \frac{C_{max}}{C_{min}} = \left(1 + \frac{20}{0.7}\right)^{0.5} \approx 5.5 $$

Hyperabrupt designs (n ≈ 0.33) trade reduced ratio for linearized tuning characteristics. Modern GaAs varactors achieve ratios exceeding 10:1, enabling wideband RF applications.

Trade-offs and Design Considerations

Varactor Capacitance vs. Reverse Bias Cmax Cmin Reverse Bias Voltage (V)
Varactor Capacitance vs. Reverse Bias Voltage A graph showing the nonlinear relationship between varactor capacitance and reverse bias voltage, with labeled points for Cmax, Cmin, V0, and Vmax. Capacitance (C) Reverse Bias Voltage (V) Cmax 0 Cmin V0 Vmax
Diagram Description: The diagram would physically show the nonlinear relationship between varactor capacitance and reverse bias voltage, illustrating how Cmax and Cmin are defined at specific voltage points.

4.2 Quality Factor (Q)

The quality factor (Q) of a varactor diode quantifies its efficiency in storing and releasing energy at a given frequency. It is a critical parameter in tuning circuits, oscillators, and filters, where high Q values indicate lower energy losses. The Q factor is defined as the ratio of the reactance to the series resistance at the operating frequency:

$$ Q = \frac{X_s}{R_s} = \frac{1}{2\pi f R_s C_j} $$

Here, Xs is the reactance of the varactor, Rs is the series resistance, f is the operating frequency, and Cj is the junction capacitance. A higher Q implies lower resistive losses and better frequency selectivity.

Derivation of Q for a Varactor Diode

The quality factor can be derived by analyzing the equivalent circuit of a varactor diode, which consists of a voltage-dependent capacitance Cj(V) in series with a resistance Rs (representing bulk and contact resistances). The impedance Z of the diode is:

$$ Z = R_s + \frac{1}{j\omega C_j} $$

The magnitude of the reactance |Xs| is 1/(ωCj), leading to the standard definition of Q. For practical varactors, Rs is minimized to maximize Q, especially in high-frequency applications.

Frequency Dependence of Q

The Q factor is frequency-dependent due to the interplay between Cj and Rs. At low frequencies, Q tends to be high because the reactance dominates. However, as frequency increases, parasitic effects (e.g., series inductance, skin effect) degrade Q. The cutoff frequency fc, where Q drops to unity, is a key metric:

$$ f_c = \frac{1}{2\pi R_s C_j} $$

Beyond fc, the varactor becomes ineffective as a tuning element.

Practical Implications

In voltage-controlled oscillators (VCOs) and phase-locked loops (PLLs), a high Q ensures lower phase noise and better stability. For example, in a VCO operating at 2 GHz, a varactor with Q > 50 is typically required to minimize signal degradation. Modern hyperabrupt junction varactors achieve Q values exceeding 100 at microwave frequencies by optimizing doping profiles and minimizing Rs.

Trade-offs and Limitations

While high Q is desirable, it often conflicts with other parameters like tuning range (Cmax/Cmin). Hyperabrupt varactors, for instance, offer wide tuning but at the cost of reduced Q. Designers must balance these factors based on application requirements.

Quality Factor (Q) vs. Frequency Frequency (f) Q

The figure illustrates the typical Q-frequency relationship, showing peak Q at mid-range frequencies before parasitic effects dominate.

Quality Factor (Q) vs. Frequency A line graph showing the relationship between Quality Factor (Q) and Frequency, with a peak Q at mid-range frequencies and a drop due to parasitic effects, including a labeled cutoff frequency point. Frequency (f) Quality Factor (Q) 0 f₁ f₂ f₃ 0 Q₁ Q₂ Q₃ f_c (cutoff) Peak Q
Diagram Description: The diagram would show the relationship between Q and frequency, illustrating the peak Q at mid-range frequencies and the drop due to parasitic effects.

4.3 Breakdown Voltage and Leakage Current

Breakdown Voltage in Varactor Diodes

The breakdown voltage (VBR) of a varactor diode is the reverse-bias voltage at which the depletion region experiences avalanche or Zener breakdown, leading to a sharp increase in current. Unlike conventional diodes, varactors are optimized for capacitive tuning rather than rectification, so VBR must be carefully considered to avoid catastrophic failure in voltage-controlled oscillators (VCOs) or tunable filters.

The breakdown mechanism is governed by the electric field (E) in the depletion region. For abrupt junction varactors, the critical field strength (Ecrit) is approximated by:

$$ E_{crit} = \sqrt{\frac{2qN_D}{\epsilon_s} V_{BR}} $$

where q is the electron charge, ND is the doping concentration, and ϵs is the semiconductor permittivity. Hyperabrupt junctions exhibit a lower VBR due to their nonlinear doping profile, trading off tuning range for voltage sensitivity.

Leakage Current and Reverse-Bias Behavior

Under reverse bias, varactors exhibit leakage current (IR) due to minority carrier diffusion and generation-recombination in the depletion region. This current follows the Shockley diode equation modified for reverse bias:

$$ I_R = I_S \left( e^{\frac{qV}{nkT}} - 1 \right) + \frac{qn_i W A}{\tau_g} $$

where IS is the saturation current, ni is the intrinsic carrier concentration, W is the depletion width, A is the junction area, and Ï„g is the generation lifetime. At high temperatures, leakage increases exponentially, degrading the diode's Q-factor in RF applications.

Practical Implications

Measurement Techniques

Breakdown voltage is measured using a curve tracer with current compliance to prevent damage. Leakage current requires sensitive picoammeters due to its low magnitude (nA–pA range). For high-precision applications, guarding techniques eliminate parasitic currents in test fixtures.

Varactor Diode Breakdown Voltage and Leakage Current A diagram showing the relationship between reverse-bias voltage and leakage current in a varactor diode, highlighting the breakdown voltage point and the exponential increase in current. Reverse-Bias Voltage (V) Leakage Current (I_R) 0 V₁ V₂ V_BR 0 I₁ I₂ I_R Breakdown (V_BR) E_crit Safe Operating Region Avalanche Breakdown Region
Diagram Description: A diagram would visually show the relationship between reverse-bias voltage and leakage current, including the breakdown voltage point and the exponential increase in current.

5. Temperature Effects on Performance

5.1 Temperature Effects on Performance

The performance of varactor diodes is highly sensitive to temperature variations due to their dependence on semiconductor material properties, junction capacitance, and reverse bias characteristics. Temperature-induced changes affect key parameters such as capacitance-voltage (C-V) characteristics, quality factor (Q), and tuning linearity.

Thermal Dependence of Junction Capacitance

The junction capacitance Cj of a varactor diode is given by:

$$ C_j(V, T) = \frac{C_{j0}(T)}{\left(1 + \frac{V}{\phi(T)}\right)^\gamma} $$

where Cj0(T) is the zero-bias capacitance, V is the applied reverse bias, φ(T) is the temperature-dependent built-in potential, and γ is the grading coefficient. The built-in potential φ(T) decreases with temperature due to increased intrinsic carrier concentration ni(T):

$$ \phi(T) = \phi_0 - \alpha (T - T_0) $$

where α is the temperature coefficient (typically ~2 mV/°C for silicon). This results in a net increase in Cj at higher temperatures for a fixed bias voltage.

Temperature Effects on Quality Factor (Q)

The quality factor Q degrades with temperature due to increased series resistance Rs(T) and altered dielectric losses. The relationship is given by:

$$ Q(T) = \frac{1}{2\pi f R_s(T) C_j(T)} $$

For silicon varactors, Rs(T) increases approximately linearly with temperature due to reduced carrier mobility:

$$ R_s(T) = R_{s0} \left[1 + \beta (T - T_0)\right] $$

where β ≈ 0.008 °C-1 for lightly doped silicon. At high frequencies (>1 GHz), this can lead to significant Q degradation in uncooled applications.

Tuning Nonlinearity and Temperature Compensation

Temperature-induced nonlinearity in tuning response can be mitigated through:

Advanced designs often integrate temperature-stable varactors with negative temperature coefficient (NTC) components to maintain consistent tuning characteristics across operating conditions.

Practical Considerations in RF Systems

In voltage-controlled oscillators (VCOs), temperature-dependent varactor behavior causes frequency drift. The normalized frequency sensitivity is:

$$ \frac{\Delta f}{f_0} = -\frac{1}{2} \frac{\Delta C}{C_0} \approx \frac{\gamma}{2} \frac{\alpha (T - T_0)}{\phi_0 + V} $$

For a typical hyperabrupt junction (γ = 0.5) at V = 5V, this results in ~100 ppm/°C frequency drift—critical in precision RF systems requiring <50 ppm stability.

5.2 Nonlinearity and Distortion

The voltage-dependent capacitance of a varactor diode inherently introduces nonlinear behavior, leading to harmonic distortion and intermodulation products in RF systems. This nonlinearity arises from the depletion region's dependence on the applied reverse bias, governed by the doping profile and semiconductor physics.

Mathematical Modeling of Nonlinear Capacitance

The junction capacitance Cj follows a power-law relationship with reverse voltage VR:

$$ C_j(V_R) = \frac{C_{j0}}{(1 - V_R/\phi)^\gamma} $$

where Cj0 is the zero-bias capacitance, φ is the built-in potential (~0.7V for Si), and γ is the grading coefficient (0.5 for abrupt junctions, 0.33 for graded junctions). Expanding this as a Taylor series around the bias point V0 reveals the nonlinear terms:

$$ C_j(V) \approx C_0 + C_1(v) + C_2(v^2) + C_3(v^3) + \cdots $$

where v = VR - V0 is the AC signal component, and the coefficients Cn are voltage-dependent derivatives of the capacitance function.

Harmonic Generation Mechanism

When a sinusoidal signal v(t) = Vmsin(ωt) is applied, the nonlinear capacitance produces current components at multiples of the fundamental frequency:

$$ i(t) = \frac{d}{dt}[C(v) \cdot v(t)] $$

The quadratic (C2) and cubic (C3) terms generate second and third harmonics at 2ω and 3ω respectively. For a two-tone input at frequencies ω1 and ω2, intermodulation products appear at 2ω1 ± ω2 and 2ω2 ± ω1.

Distortion Metrics in Practical Applications

Key figures of merit for varactor linearity include:

In voltage-controlled oscillators (VCOs), these nonlinearities contribute to phase noise through AM-to-PM conversion, where amplitude fluctuations modulate the varactor capacitance, creating frequency deviations.

Linearity Improvement Techniques

Several methods mitigate varactor distortion:

Varactor Diode Nonlinear Effects Linear C-V Actual C-V Bias Point
Varactor Nonlinearity Effects A combined plot showing the nonlinear C-V characteristic of a varactor diode, time-domain waveforms, and frequency-domain distortion products. V C Ideal Linear Actual Nonlinear Cj0 VR φ γ C-V Characteristic t V Input Output Time-Domain Waveforms f P ω 2ω 3ω ω1±ω2 IP3 Frequency-Domain Distortion
Diagram Description: The diagram would physically show the nonlinear C-V curve versus an ideal linear response, harmonic distortion products in the frequency domain, and intermodulation effects from two-tone signals.

5.3 Handling and Biasing Best Practices

Reverse Biasing and Voltage Control

Varactor diodes operate exclusively under reverse bias, where the depletion region width modulates with applied voltage, altering capacitance. The junction capacitance \( C_j \) follows:

$$ C_j = \frac{C_0}{\left(1 + \frac{V_R}{\phi}\right)^n} $$

Here, \( C_0 \) is the zero-bias capacitance, \( V_R \) is the reverse voltage, \( \phi \) is the built-in potential (~0.7 V for silicon), and \( n \) is the grading coefficient (0.5 for abrupt junctions, 0.33 for hyperabrupt). Exceeding the breakdown voltage \( V_{BR} \) causes irreversible damage.

Thermal Management

Leakage current \( I_R \) increases exponentially with temperature, degrading the quality factor \( Q \):

$$ Q = \frac{1}{2\pi f C_j R_s} $$

where \( R_s \) is the series resistance. For high-frequency applications (e.g., VCOs), maintain junction temperatures below 85°C using:

ESD Protection

Varactors are sensitive to electrostatic discharge (ESD) due to thin depletion layers. Mitigation strategies include:

Bias Network Design

To minimize parasitic effects in RF circuits:

$$ Z_{bias} = \frac{1}{j\omega C} + j\omega L $$

where \( Z_{bias} \) should present > 1 kΩ impedance at the operating frequency.

Practical Tuning Considerations

For linear frequency tuning in VCOs, hyperabrupt varactors (\( n \approx 0.33 \)) are preferred. The tuning sensitivity \( K_V \) is:

$$ K_V = \frac{df}{dV} = \frac{-n f_0}{2 \phi \left(1 + \frac{V_R}{\phi}\right)^{n+1}} $$

where \( f_0 \) is the center frequency. For minimal phase noise, avoid operating near \( V_R = 0 \) due to higher \( R_s \).

Varactor Diode C-V Characteristics Graph showing the relationship between reverse bias voltage (V_R) and junction capacitance (C_j) for abrupt (n=0.5) and hyperabrupt (n=0.33) junctions, including breakdown voltage (V_BR) marker. Junction Capacitance (C_j) 10 1 0.1 0.01 Reverse Bias Voltage (V_R) 0 φ V_BR V_R C_0 n=0.5 n=0.33 Breakdown (V_BR)
Diagram Description: A diagram would visually show the relationship between reverse bias voltage and junction capacitance, including the breakdown threshold.

6. Key Research Papers and Books

6.1 Key Research Papers and Books

6.2 Manufacturer Datasheets

6.3 Online Resources and Tutorials