digital to analog converters

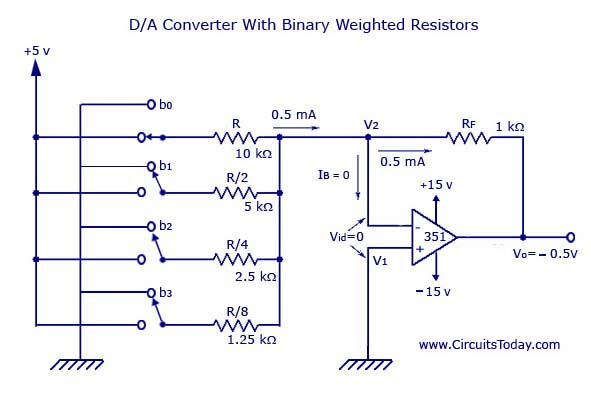
A digital-to-analog (D/A) converter utilizing binary-weighted resistors is illustrated in the accompanying figure. In this circuit, the operational amplifier (op-amp) is configured in inverting mode, although it may also be arranged in non-inverting mode. The schematic represents a 4-bit converter, meaning it has four binary inputs. Consequently, a 4-bit converter will yield 2^4 = 16 combinations of output, leading to 16 corresponding analog outputs for the binary inputs. Depending on the ON/OFF status of the switches (b0-b3), the respective binary-weighted currents will be generated at the input resistor. The current flowing through the feedback resistor (Rf) will be the aggregate of these currents, which is then converted into a proportional output voltage. The output will reach its maximum when all switches (b0-b3) are closed. The output manifests as a negative-going staircase waveform with 15 steps of -0.5V each. In practical applications, due to variations in the logic HIGH voltage levels, the step sizes may not be uniform. The value of the feedback resistor (Rf) influences the magnitude of these steps; thus, selecting an appropriate feedback resistor allows for the desired step size. It is essential to ensure that the maximum output voltage does not surpass the saturation levels of the op-amp. Metal-film resistors are recommended for achieving accurate outputs. If the number of inputs exceeds four or the combinations exceed sixteen, binary-weighted resistors may not be readily available. Therefore, the R and 2R method is preferred, as it requires only two sets of precision resistance values. A D/A converter using R and 2R resistors is also depicted in the figure. Similar to the binary-weighted resistor method, the binary inputs are represented by switches (b0-b3), and the output remains proportional to these binary inputs. The binary inputs can be in either the HIGH (+5V) or LOW (0V) state, with b3 designated as the most significant bit, connected to +5V, while all other switches are grounded.

The circuit operates by converting binary input signals into a corresponding analog voltage level. Each switch (b0 to b3) represents a binary digit, where the state of each switch determines whether it contributes to the output voltage. When a switch is in the ON position, it connects to the logic HIGH voltage level, contributing a weighted current based on its binary significance. The feedback resistor (Rf) plays a critical role in scaling the output voltage; its value must be chosen to ensure that the output remains within the operational limits of the op-amp.

In the binary-weighted resistor configuration, each resistor is typically chosen to be half the value of the previous one, allowing for precise control over the current contributions from each switch. As the number of inputs increases, the complexity of using binary-weighted resistors grows, leading to potential inaccuracies due to resistor tolerances. The R and 2R method simplifies this by using only two resistor values, which reduces the number of components needed and enhances reliability.

The output waveform produced by the D/A converter is a staircase function, which is a common characteristic of such converters. Each step represents a discrete voltage level corresponding to a unique binary input combination. The accuracy of the output can be affected by several factors, including the quality of the resistors used, the stability of the power supply, and the op-amp's characteristics. To ensure optimal performance, careful consideration must be given to the component selection and circuit layout.A D/A converter using binary-weighted resistors is shown in the figure below. In the circuit, the op-amp is connected in the inverting mode. The op-amp can also be connected in the non-inverting mode. The circuit diagram represents a 4-digit converter. Thus, the number of binary inputs is four. We know that, a 4-bit converter will have 24 = 16 com binations of output. Thus, a corresponding 16 outputs of analog will also be present for the binary inputs. Thus, according to the position (ON/OFF) of the switches (bo-b3), the corresponding binary-weighted  currents will be obtained in the input resistor. The current through Rf will be the sum of these currents. This overall current is then converted to its proportional output voltage. Naturally, the output will be maximum if the switches (b0-b3) are closed The output is a negative going staircase waveform with 15 steps of -).

5V each. In practice, due to the variations in the logic HIGH voltage levels, all the steps will not have the same size. The value of the feedback resistor Rf changes the size of the steps. Thus, a desired size for a step can be obtained by connecting the appropriate feedback resistor. The only condition to look out for is that the maximum output voltage should not exceed the saturation levels of the op-amp.

Metal-film resistors are more preferred for obtaining accurate outputs. If the number of inputs (>4) or combinations (>16) is more, the binary-weighted resistors may not be readily available. This is why; R and 2R method is more preferred as it requires only two sets of precision resistance values.

A D/A converter with R and 2R resistors is shown in the figure below. As in the binary-weighted resistors method, the binary inputs are simulated by the switches (b0-b3), and the output is proportional to the binary inputs. Binary inputs can be either in the HIGH (+5V) or LOW (0V) state. Let b3 be the most significant bit and thus is connected to the +5V and all the other switchs are connected to the ground.

🔗 External reference