Q-Meter
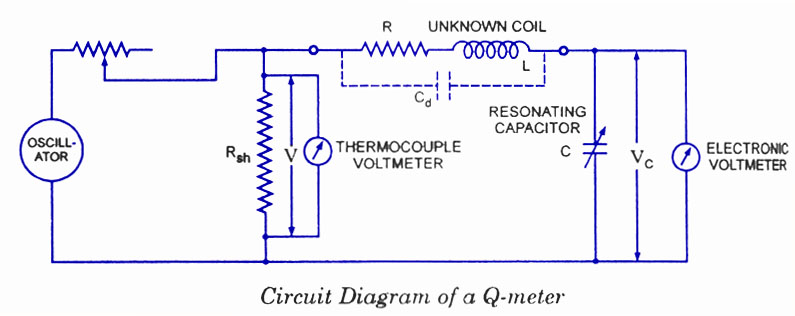
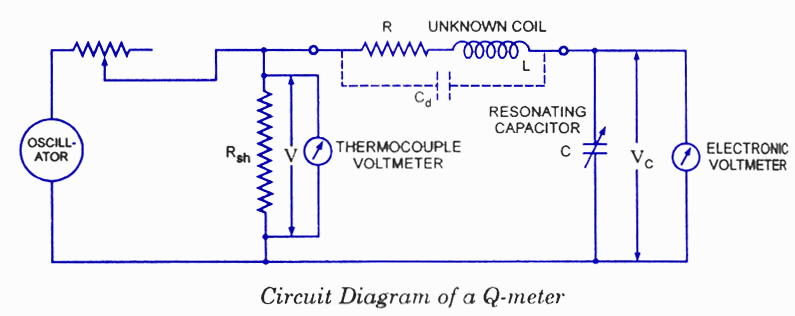
Every inductor coil possesses a certain amount of resistance, and it is essential for the coil to have the lowest possible resistance. The quality factor, or Q-factor, of the coil is the ratio of the inductive reactance to the effective resistance. A high Q value is desirable as it indicates high inductive reactance and low resistance. Conversely, a low Q value signifies a relatively high resistance component, resulting in a significant power loss. The effective resistance of the coil differs from its DC resistance due to eddy currents and skin effects, and it varies in a complex manner with frequency. Consequently, Q is seldom calculated using R and L alone. One method to determine Q is through the use of an inductance bridge; however, these bridge circuits often fail to provide accurate measurements at high Q values. Therefore, specialized meters are employed for precise Q-factor determination. The Q-meter is an instrument designed to measure the Q-factor of coils and the electrical properties of coils and capacitors. This instrument operates on the principle of series resonance; at resonance in an AC series circuit, the voltage across the capacitor equals the applied voltage times the Q of the circuit. When the applied voltage is kept constant, a voltmeter connected across the capacitor can be calibrated to indicate Q directly. A circuit diagram of a Q-meter typically includes a wide-range oscillator with a frequency range from 50 kHz to 50 MHz, serving as the power supply for the circuit. The output of the oscillator is shorted by a low-value resistance, Rsh, usually around 0.02 ohms, which introduces negligible resistance into the oscillatory circuit and acts as a voltage source with minimal internal resistance. The voltage across the low-value shunt resistance Rsh is measured by a thermocouple meter, while the voltage across the capacitor, Vc, is measured by an electronic voltmeter. To perform the measurement, the unknown coil is connected to the test terminals of the instrument, and the circuit is tuned to resonance by either varying the frequency of the oscillator or adjusting the resonating capacitor C. Voltage readings across capacitor C and shunt resistance Rsh are obtained, allowing the Q-factor of the coil to be determined. This Q-factor is referred to as the circuit Q because it accounts for the losses in the resonating capacitor, voltmeter, and shunt resistor Rsh. As a result, the actual Q-factor of the coil will be slightly greater than the calculated value, although this difference is typically negligible unless the resistance of the coil under test is small compared to the shunt resistance Rsh.
The Q-meter is a crucial instrument in the field of electronics, particularly for applications involving inductors and capacitors where precise measurements of Q-factor are vital for performance evaluation. The operational principle of the Q-meter leverages the resonant behavior of circuits, which is foundational in RF design and analysis.
In practice, the Q-meter's oscillator generates a stable sine wave signal, which is essential for accurate resonance tuning. The choice of a wide frequency range (50 kHz to 50 MHz) allows for versatility in testing various components, accommodating different inductance values and resonant frequencies. The shunt resistor Rsh, being of low resistance, ensures minimal impact on the circuit's performance, allowing for more accurate readings of the voltage across the capacitor.
The calibration of the voltmeter to directly indicate the Q-factor simplifies the measurement process, making it accessible for engineers and technicians. The tuning process, which can be performed either by adjusting the oscillator frequency or the capacitor value, enables precise resonance conditions to be achieved, thereby enhancing the reliability of the Q-factor measurement.
Furthermore, the inclusion of a thermocouple meter for measuring the voltage across Rsh ensures that temperature variations do not significantly affect the readings, thus maintaining the accuracy of the Q-factor determination. The overall design of the Q-meter integrates these elements to create a robust and effective tool for evaluating the quality of inductive components, which is critical in the development and testing of electronic circuits.We know that every inductor coil has a certain amount of resistance and the coil should have lowest possible resistance. The ratio of the inductive reactance to the effective resistance of the coil is called the quality factor or Q-factor of the coil.
A high value of Q is always desirable as it means high inductive reactance and low resistance. A low value of Q indicates that the resistance component is relatively high and so there is a comparatively large loss of power. The effective resistance of the coil differs from its dc resistance because of eddy current and skin effects and varies in a highly complex manner with the frequency.
For this reason Q is rarely computed by determination of R and L. One possible way for determination of Q is by using the inductance bridge but such bridge circuits are rarely capable of giving accurate measurements, when Q is high. So special meters are used for determination of Q accurately. The Q-meter is an instrument designed for the measurement of Q-factor of the coil as well as for the measurement of electrical properties of coils and capacitors.
-This instru ment operates on the principle of series resonance i. e. at resonate condition of an ac series circuit voltage across the capacitor is equal to the applied voltage times of Q of the circuit. If the voltage applied across the circuit is kept-constant then voltmeter connected across the capacitor can be calibrated to indicate Q directly.
Circuit diagram of a Q-meter is shown is figure. A wide-range os cillator with frequency range from 50 kHz to 50 MHz is used as a power supply to the cir cuit. The output of the oscillator is shorted by a low-value resistance, Rsh usually of the or der of 0. 02 ohm. So it in troduces almost no resistance into the oscillatory circuit and represents a voltage source with a very small or of almost negligible internal resistance.
The voltage across the low-value shunt resistance Rsh, V is measured by a thermo-couple meter and the voltage across the capacitor, Vc is measured by an electronic voltmeter. For carrying out the measurement, the unknown coil is connected to the test termi nals of the instrument, and the circuit is tuned to resonance either by varying the fre quency of the oscillator or by varying the resonating capacitor C.
Readings of voltages across capacitor C and shunt resistance Rsh are obtained and Q-factor of the coil is deter mined as follows : This Q-factor is called the circuit Q because this measurement includes the losses of the resonating capacitor, voltmeter and the shunt resistor Rsh. So, the actual Q-factor of the coil will be somewhat greater than the calculated Q-factor. This difference is usually very small and maybe neglected. , except when the resistance of the coil under test is relatively small in comparison to the shunt resistance Rsh.
🔗 External reference
The Q-meter is a crucial instrument in the field of electronics, particularly for applications involving inductors and capacitors where precise measurements of Q-factor are vital for performance evaluation. The operational principle of the Q-meter leverages the resonant behavior of circuits, which is foundational in RF design and analysis.
In practice, the Q-meter's oscillator generates a stable sine wave signal, which is essential for accurate resonance tuning. The choice of a wide frequency range (50 kHz to 50 MHz) allows for versatility in testing various components, accommodating different inductance values and resonant frequencies. The shunt resistor Rsh, being of low resistance, ensures minimal impact on the circuit's performance, allowing for more accurate readings of the voltage across the capacitor.
The calibration of the voltmeter to directly indicate the Q-factor simplifies the measurement process, making it accessible for engineers and technicians. The tuning process, which can be performed either by adjusting the oscillator frequency or the capacitor value, enables precise resonance conditions to be achieved, thereby enhancing the reliability of the Q-factor measurement.
Furthermore, the inclusion of a thermocouple meter for measuring the voltage across Rsh ensures that temperature variations do not significantly affect the readings, thus maintaining the accuracy of the Q-factor determination. The overall design of the Q-meter integrates these elements to create a robust and effective tool for evaluating the quality of inductive components, which is critical in the development and testing of electronic circuits.We know that every inductor coil has a certain amount of resistance and the coil should have lowest possible resistance. The ratio of the inductive reactance to the effective resistance of the coil is called the quality factor or Q-factor of the coil.
A high value of Q is always desirable as it means high inductive reactance and low resistance. A low value of Q indicates that the resistance component is relatively high and so there is a comparatively large loss of power. The effective resistance of the coil differs from its dc resistance because of eddy current and skin effects and varies in a highly complex manner with the frequency.
For this reason Q is rarely computed by determination of R and L. One possible way for determination of Q is by using the inductance bridge but such bridge circuits are rarely capable of giving accurate measurements, when Q is high. So special meters are used for determination of Q accurately. The Q-meter is an instrument designed for the measurement of Q-factor of the coil as well as for the measurement of electrical properties of coils and capacitors.
-This instru ment operates on the principle of series resonance i. e. at resonate condition of an ac series circuit voltage across the capacitor is equal to the applied voltage times of Q of the circuit. If the voltage applied across the circuit is kept-constant then voltmeter connected across the capacitor can be calibrated to indicate Q directly.
Circuit diagram of a Q-meter is shown is figure. A wide-range os cillator with frequency range from 50 kHz to 50 MHz is used as a power supply to the cir cuit. The output of the oscillator is shorted by a low-value resistance, Rsh usually of the or der of 0. 02 ohm. So it in troduces almost no resistance into the oscillatory circuit and represents a voltage source with a very small or of almost negligible internal resistance.
The voltage across the low-value shunt resistance Rsh, V is measured by a thermo-couple meter and the voltage across the capacitor, Vc is measured by an electronic voltmeter. For carrying out the measurement, the unknown coil is connected to the test termi nals of the instrument, and the circuit is tuned to resonance either by varying the fre quency of the oscillator or by varying the resonating capacitor C.
Readings of voltages across capacitor C and shunt resistance Rsh are obtained and Q-factor of the coil is deter mined as follows : This Q-factor is called the circuit Q because this measurement includes the losses of the resonating capacitor, voltmeter and the shunt resistor Rsh. So, the actual Q-factor of the coil will be somewhat greater than the calculated Q-factor. This difference is usually very small and maybe neglected. , except when the resistance of the coil under test is relatively small in comparison to the shunt resistance Rsh.
🔗 External reference
Related Circuits
Every inductor coil possesses a certain amount of resistance, and it is essential for the coil to have the lowest possible resistance. The ratio of inductive reactance to the effective resistance of the coil is referred to as the...