capacity Computing Current from known Capacitance and Time to Charge to Known Voltage

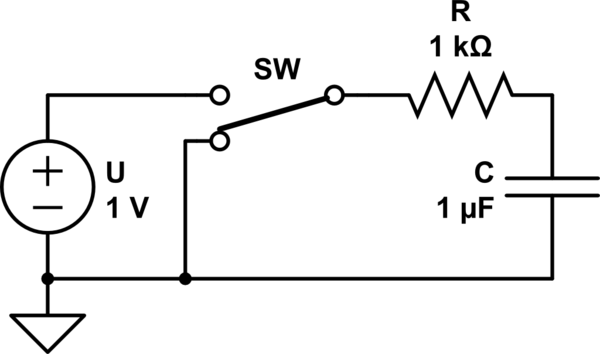
Consider a capacitor with capacitance C that charges to a voltage V and discharges to 0V at a frequency of 5 times per second. To estimate the average current, also referred to as the RMS current, a rough approximation can be made. The average current is calculated as the change in charge divided by the time taken. The change in charge is determined by the capacitance multiplied by the change in voltage. It is important to note that the average current is not necessarily the same as the RMS current, as the latter is influenced by how the current varies over time. Additional information about the nature of the charging and discharging process, such as whether it occurs at a constant current or through a constant resistance to a specified voltage, is required for a precise calculation. During a cycling charge/discharge process, the long-term average current will be zero, while the RMS current will remain non-zero.

In an electrical circuit involving a capacitor, the charging and discharging cycles can be analyzed to determine current characteristics. The capacitor, with capacitance C, stores energy when charged to a voltage V. The charge (Q) stored in the capacitor can be expressed as Q = C * V. During the charging phase, the capacitor receives a certain amount of charge over a defined time interval. The average current (I_avg) during this phase can be approximated using the formula:

I_avg = ΔQ / Δt

where ΔQ is the change in charge and Δt is the time taken for the change. For a capacitor charging from 0V to V, ΔQ is C * V, and if the charging occurs over a half-cycle (0.1 seconds for a 5 Hz frequency), then:

I_avg = (C * V) / (0.1 seconds)

This gives a rough estimate of the average current during the charging phase.

The discharging phase, where the capacitor releases its stored energy back to 0V, follows a similar approach. The average current during discharging will also be calculated over the same time interval, resulting in a similar formula.

It is crucial to differentiate between average current and RMS current (I_rms). The RMS current is a measure of the effective value of the varying current and is calculated over a complete cycle. For a sinusoidal waveform, the RMS current can be derived from the average current and the shape of the waveform. For a capacitor discharging and charging in a square wave manner, the RMS current can be calculated as:

I_rms = I_max / √2

where I_max is the peak current during either the charging or discharging phase.

In summary, while the average current provides a basic understanding of the capacitor's behavior during charge and discharge cycles, the RMS current offers a more comprehensive view of the effective current flowing through the circuit, especially when considering the waveform's characteristics.Assume a capacitor of capacity C is charging to voltage V and discharging to 0v at a rate of 5 times per second. How do I figure out the average current or what you might call RMS current I don`t need this to be exact - very rough approximation.

In general, the average current is equal to the change in charge divided by the time taken. But the change in charge is equal to the capacitance C multiplied by the change in voltage, which you know :- Your last paragraph is harder to address. The average current is not usually the same as the RMS current which will depend on the how the current varies with time. We would need to know how the charging/discharging takes place, for example constant current or through a constant resistance to a known voltage.

If you are cycling the charge/discharge process, the long-term average current will of course be zero but the RMS current will be non-zero. 🔗 External reference