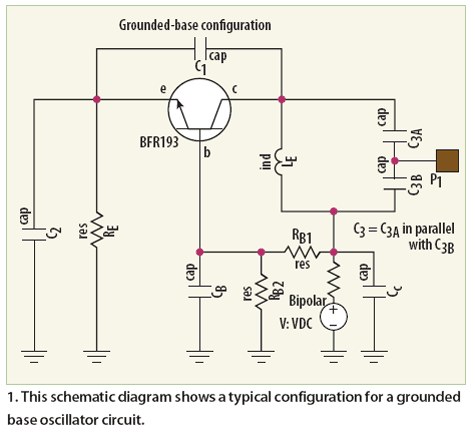
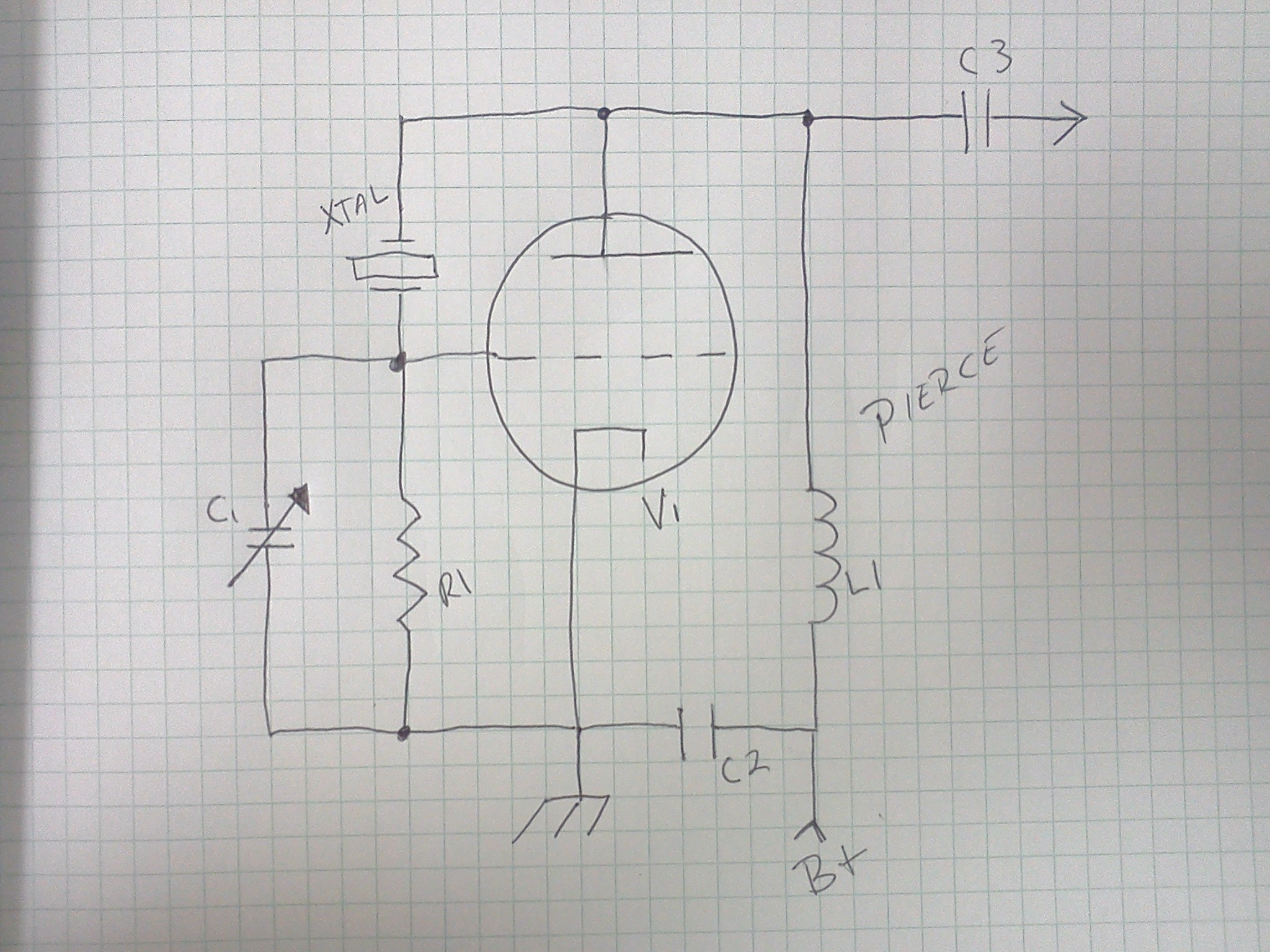
Oscillators and Triggers

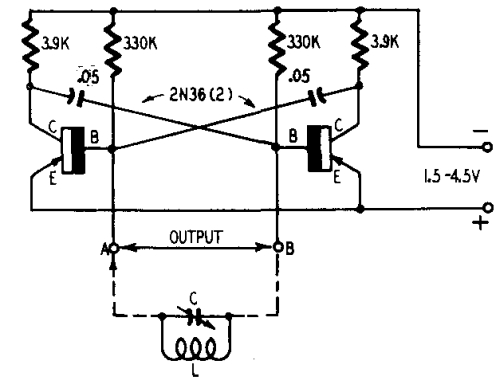
Transistors are increasingly being utilized in various applications. Many circuits employing transistors have been developed based on previous designs that used vacuum tubes. The following chapters present unique applications that leverage the advantageous properties of transistors. The described circuits are practical, have been constructed, tested, and are currently operational. Although transistors typically require specific circuitry, some effective vacuum tube circuits can function well with transistor substitutions. The phase relationships of a grounded-emitter transistor are similar to those of a grounded-cathode vacuum tube, allowing junction transistors to replace vacuum tubes in most oscillator circuits. The multivibrator and two-terminal sine-wave generator operate effectively with junction transistors, with the transistorized two-terminal L-C circuit offering significant advantages over other transistor oscillators. Like vacuum tubes, these circuits oscillate at higher frequencies than single-transistor oscillators using the same components. While specific transistors must be chosen for certain oscillators, many transistors, including those not used in single oscillators, perform well in RF applications within this circuit. The multivibrator is fundamentally a two-stage R-C coupled amplifier. The output voltage from the second stage is fed back to the grid of the first stage, initiating oscillation at a frequency determined by the time constants of R and C. This circuit will also oscillate if a component with similar amplification and phase-shift characteristics replaces the vacuum tubes. Before exploring additional circuitry, it is essential to discuss the principle of analogy. A junction transistor and a vacuum-tube triode are illustrated in common-emitter and common-cathode configurations. The emitter, collector, and base of the transistor correspond to the cathode, plate, and grid of the vacuum tube, respectively. An AC signal experiences a phase reversal between the input and output electrodes—base and collector for the transistor, grid and plate for the tube. When the grid of the vacuum tube is made more positive, the plate current increases, which in turn causes the plate to become less positive due to the voltage drop across the load resistor. Consequently, the peaks of a sine wave applied to the grid transform into troughs of the anode voltage. In a grounded-emitter circuit of a p-n-p junction transistor, making the base more negative relative to the emitter increases the collector current, resulting in a higher positive voltage at the collector due to the load resistor's voltage drop. Thus, a sine wave is inverted, producing the same 180° phase shift as a grounded-cathode vacuum tube. The grounded-emitter circuit of a junction transistor parallels the grounded-cathode circuit of a vacuum tube, although there are notable differences. In typical operation, the grid is connected to a negative source with zero grid current, while the base return in transistors with a positive collector is positive and does not have zero base current. This results in a finite, relatively low input resistance for the transistor, unlike the infinite input resistance of the vacuum tube. Additionally, the output resistances differ, with transistors exhibiting significantly higher output resistance than tubes. However, as the alpha ratio (the change in collector current to the change in emitter current) approaches unity, these differences diminish, and the analogy becomes more accurate. The input resistance of the transistor increases while its output resistance decreases correspondingly.

The circuit design utilizing transistors, particularly in oscillators, capitalizes on their ability to handle high frequencies and their compatibility with existing vacuum tube circuits. The multivibrator circuit, for instance, demonstrates how feedback mechanisms can effectively initiate oscillations, with the frequency determined by the reactive components in the circuit. The transition from vacuum tubes to transistors not only enhances performance but also allows for more compact designs with greater efficiency. The grounding configurations play a crucial role in determining the phase relationships and overall functionality of the circuit, making the understanding of these principles essential for effective circuit design. The advantages of transistors, such as improved frequency response and higher output resistance, continue to drive innovation in electronic circuit design, leading to more robust and versatile applications in modern electronics.Transistors are finding new and ever-increasing uses. Some of the circuits in which transistors are being applied are well-known through prior employment in conjunction with vacuum tubes. The applications shown in the chapters which follow illustrate some of the more unique ways in which to take advantage of the desirable properties of transistors

. The circuits that are described are practical, have been built, tested, and are now in use. THOUGH transistors generally demand their own circuitry, there are some good vacuum-tube circuits that function nicely when transistors are substituted. As the phase relations of a grounded-emitter transistor resemble those of a grounded-cathode vacuum tube, it is possible to use junction transistors for vacuum tubes in most oscillator circuits.

The multivibrator and the two-terminal sine-wave generator perform well with junction transistors, with the transistorized two-terminal L-C having a great advantage over other transistor oscillators. As with vacuum tubes, the circuit oscillates on higher frequencies than single-transistor oscillators with the same components.

While transistors must be selected for other oscillators, most transistors, even those not oscillating in single oscillators, work well on rf in this circuit. The multivibrator is in principle a two-stage R-C coupled amplifier (Fig. 501). The output voltage of the second tube is fed back to the grid of the first tube. Because of this feedback the circuit starts to oscillate at a frequency determined by the time constants of R and C.

The circuit will also oscillate if you substitute an element with similar amplifying and phase-shift relations in place of the vacuum tubes. Before getting into further circuitry, let`s discuss the principle of analogy. Fig. 502 shows a junction transistor and a vacuum-tube triode in common-emitter and common-cathode connections.

The symbols e, c and b represent the emitter, collector and base, respectively. We can immediately draw an analogy between the terminals of the two devices: The emitter corresponds to the cathode, the collector to the plate, the base to the grid. The analogy may be extended by noting that an ac signal undergoes a phase reversal between input and output electrodes ”base and collector for the transistor, grid and plate for the tube.

If you make the grid of the vacuum tube more positive, plate current increases and as the plate current increases the plate becomes less positive due to the voltage drop across the load resistor. Thus, all "mountains" of a sine wave applied to the grid are trans ¬formed into "valleys" of the anode voltage.

Now look at the grounded-emitter circuit (Fig. 503) of a p-n-p junction transistor. If the base is made more negative with respect to the emitter, col ¬lector current increases and the voltage drop across the load resistor causes the collector to become more positive. So, a sine wave is "turned over" the same as with a vacuum tube, a grounded-emitter transistor producing the same 180 ° phase shift as a grounded cathode vacuum tube.

Fig 503 shows how the grounded-emitter circuit of a junction transistor corresponds to the grounded-cathode circuit of the vacuum tube. There are, of course, certain differences between the two. Whereas the grid is returned to a negative source and Ig is zero for normal operation, the base return is positive (in transistors with a positive collector) and I is not zero.

This means that the input resistance of the transistor is not infinite, as is the tube`s, but has a finite, actually low, value. The magnitudes of the output resistances are also dissimilar, the transistors` being much higher than that of the tube.

However, as alpha, the ratio of a change in Ic to a change in Ie, increases to unity, the differences decrease and the analogy becomes closer. The input resistance of the transistor rises and its output resistance drops correspondingly. An importan 🔗 External reference