Capacitance multiplier

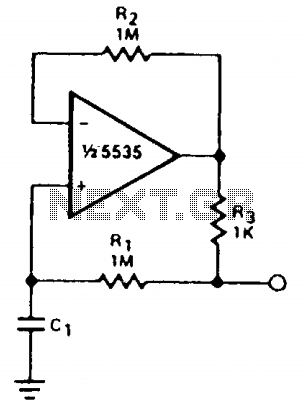
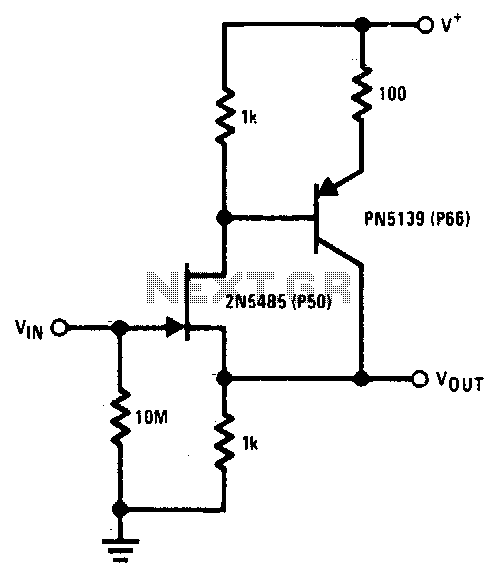
The circuit can be utilized to simulate large capacitances using components of smaller values. With the specified values and a capacitance of 10 µF, an effective capacitance of 10,000 µF was achieved. The quality factor (Q) is constrained by the effective series resistance. Therefore, R1 should be as large as practically possible.

The described circuit functions by employing a technique known as capacitance multiplication, which allows for the simulation of high capacitance values using lower-rated capacitors and resistors. This principle is particularly advantageous in applications where space and component size are critical.

In this configuration, a small capacitor (C) of 10 µF is used in conjunction with a resistor (R1) to create a network that effectively multiplies the capacitance. The effective capacitance (C_eff) achieved can be significantly higher than the actual capacitance of the capacitor used. The multiplication factor is determined by the values of the resistors and capacitors in the circuit, as well as the frequency of operation.

The effective series resistance (ESR) plays a crucial role in determining the quality factor (Q) of the circuit. A lower ESR results in a higher Q, which is desirable for applications requiring minimal energy loss and improved performance. Consequently, R1 should be chosen to be as large as feasible without compromising the circuit's operational stability or responsiveness.

In practical implementation, careful consideration must be given to the selection of components to ensure that they can handle the voltage and current requirements of the application. The circuit can be employed in various scenarios, including power supply filtering, energy storage systems, and timing applications where large capacitance values are required but larger physical components are not feasible.

Overall, this circuit design demonstrates an effective method for achieving high capacitance values through strategic component selection and configuration, enhancing both performance and versatility in electronic applications.The circuit can be used to simulate large capacitances using small value components. With the values shown and C - 10 µ¥, an effective capacitance of 10,000 µF was obtained. The Q available is limited by the effective series resistance. So R1 should be as large as practical.