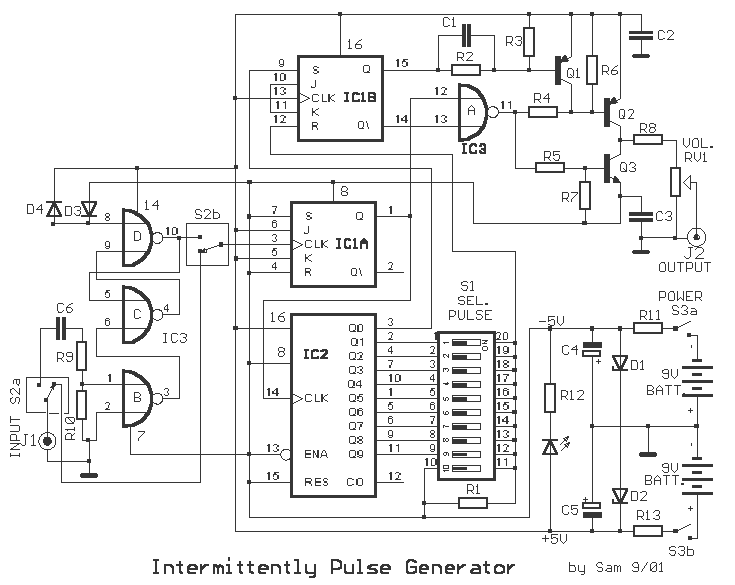
Colpitts harmonic oscillator 100Mhz

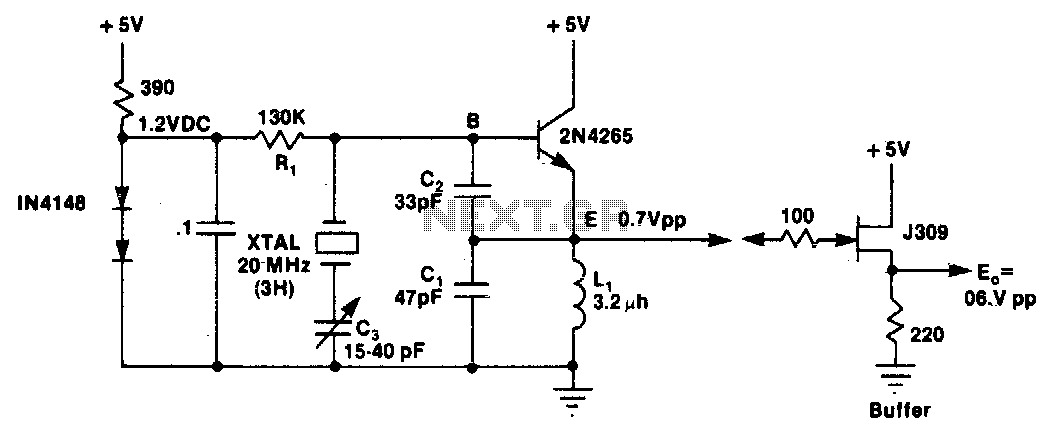
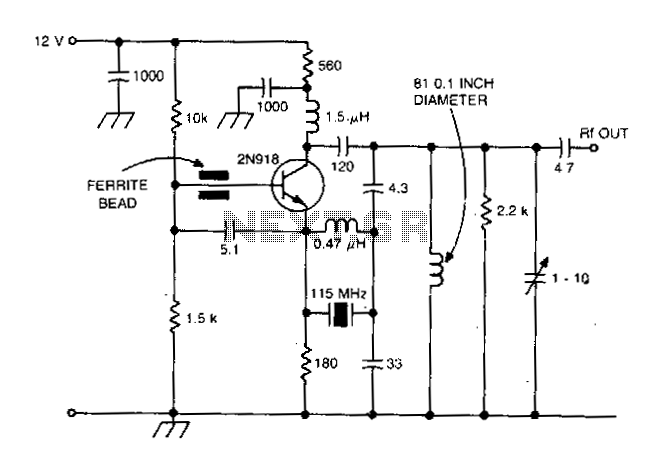
L1 and C1 are selected to resonate at a frequency below the desired crystal harmonic but above the crystal's next lower odd harmonic. Capacitor C2 should have a value between 30-70 pF, independent of the oscillation frequency. There is no specific requirement for the ratio of C1 to C2, but practical harmonic circuits tend to perform optimally when C1 is approximately 1-3 times the value of C2. Diodes D1 to D3 provide a simple regulated bias supply. The resistance of R1 should be maximized, as it influences the crystal's in-circuit quality factor (Q).

In this circuit design, the resonant components L1 and C1 are crucial for establishing the desired oscillation frequency. The selection of L1 and C1 ensures that the resonance occurs at a frequency that is strategically placed below the intended crystal harmonic, thereby allowing for effective operation while avoiding interference from the next lower odd harmonic. The specified range for C2, between 30-70 pF, is designed to maintain a stable oscillation frequency across varying conditions, ensuring reliable performance of the oscillator circuit.

The choice of C1 relative to C2 is flexible; however, it is recommended that C1 be approximately 1-3 times the capacitance of C2 to achieve optimal performance in harmonic circuits. This relationship helps to stabilize the oscillation and improve the efficiency of the circuit.

The inclusion of diodes D1, D2, and D3 serves to create a regulated bias supply, which is essential for maintaining consistent voltage levels throughout the circuit. This regulation helps to mitigate fluctuations that could adversely affect the performance of the oscillator.

Furthermore, the resistance of R1 plays a significant role in determining the quality factor (Q) of the crystal when it is in-circuit. A higher resistance value for R1 is advantageous as it enhances the Q factor, leading to sharper resonance and improved selectivity in frequency response. This is particularly important in applications where precision frequency control is required.

Overall, this circuit configuration is designed to optimize the performance of the crystal oscillator by carefully selecting component values and configurations to achieve desired operational characteristics.L1C1 are selected to be resonant at a frequency below the desired crystal harmonic but above the crystal"s next lower odd harmonic. C2 should have a value of 30-70 pF, independent of the oscillation frequency. There is no requirement for any specific ratio of C1/C2, but practical harmonic circuits seem to work best when Cl is approximately 1-3 times the value of C2.

Diodes D1-D3 provide a simple regulated bias supply The resistance of Rl should be as high as possible, as it affects the crystal"s in-circuit Q. 🔗 External reference