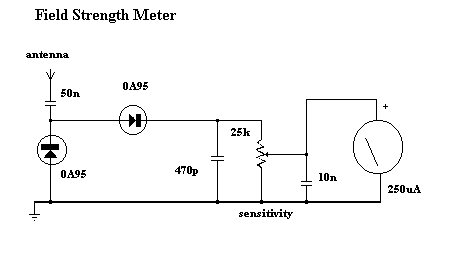
Crystals

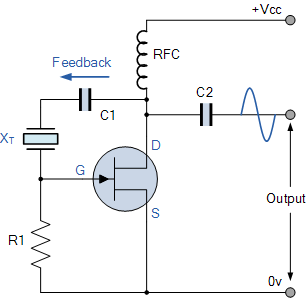
Another frequency-determining device is the crystal. The crystal may be used with a tank circuit, or it may function independently. Crystals exhibit a characteristic known as the piezoelectric effect, which is the property of a crystal that allows mechanical forces to produce electrical charges and, conversely, electrical charges to generate mechanical forces. This effect results in oscillation similar to the flywheel effect of a tank circuit. The piezoelectric effect is observed in various crystal substances, with quartz and Rochelle salt being the most significant. Although quartz does not exhibit the piezoelectric effect to the same extent as Rochelle salt, it is preferred for frequency control in oscillators due to its superior mechanical strength. Another mineral, tourmaline, is physically strong like quartz; however, its higher cost limits its use. This discussion will focus solely on quartz crystals. The crystals utilized in oscillator circuits are thin sheets or wafers cut from natural or synthetic quartz, precisely ground to a specific thickness to achieve the desired resonant frequency. These crystals are mounted in holders that provide physical support and electrodes for voltage application, while allowing the crystals to vibrate freely. Various types of holders exist, one of which is illustrated in the accompanying figure. The frequency for which a crystal is ground is termed the natural resonant frequency. When voltage is applied to the crystal, it generates mechanical vibrations that produce an output voltage at this natural resonant frequency. A vibrating crystal can be represented by an equivalent electrical circuit comprising capacitance, inductance, and resistance. The accompanying figure illustrates the symbol for a crystal, an equivalent circuit for the crystal, and another equivalent circuit that includes the holder; C1 represents the capacitance between the metal plates of the holder. The quality factor (Q) of a crystal is significantly higher than that of an LC tank circuit, with values ranging from 5,000 to 30,000 in commercially produced crystals. This high Q factor results in enhanced frequency stability compared to ordinary LC tank circuits, which explains the widespread use of crystals in sine-wave generator circuits. A basic oscillator can be divided into three main sections: a frequency-determining device, an amplifier, and a feedback circuit. In an LC oscillator, the frequency-determining device is typically an LC tank circuit, which is usually found in the input circuit of an oscillator, although it may also appear in the output circuit. Design considerations regarding the magnitude of plate and collector currents and shunting impedances influence the placement of these tank circuits. In both solid-state and electron tube circuits, oscillations occur within the tuned circuit. Both electron tubes and transistors function primarily as electrical valves that amplify and automatically provide the necessary energy to sustain oscillations in the input circuit. The feedback circuit in both tube and transistor oscillators couples energy of the appropriate amount and phase from the output back to the input circuit to maintain oscillations. Previous tutorials on tuned circuits have described the resonant or tank circuit and the generation of a sinusoidal signal through the interaction of an inductor and a capacitor. The feedback signal can be coupled from this circuit using one of two methods, the first of which involves extracting some energy from the inductor.
The crystal oscillator is a critical component in various electronic applications, providing precise frequency control essential for timing and synchronization in circuits. The operation of a crystal oscillator relies on the piezoelectric properties of the quartz crystal, which allows it to convert electrical energy into mechanical vibrations and vice versa. The natural resonant frequency is determined by the physical dimensions and the cut of the crystal, which can be optimized for specific applications.
In practical implementations, the crystal is often part of a feedback loop in an oscillator circuit, where it ensures stable oscillation frequencies. The equivalent circuit model of the crystal includes series and parallel resonant frequencies, which are crucial for understanding its behavior in different circuit configurations. The high Q factor indicates a narrow bandwidth around the resonant frequency, contributing to the oscillator's ability to maintain frequency stability over temperature variations and aging.
The design of the holder for the crystal is also significant, as it must not only support the crystal physically but also allow for its mechanical vibrations without introducing damping that could affect performance. The choice of materials for the holder and the method of mounting the crystal can influence the overall performance of the oscillator.
In summary, quartz crystals are indispensable in modern electronics, enabling precise frequency generation and control through their unique piezoelectric properties and high Q factors. Their application extends to various fields, including telecommunications, computing, and signal processing, where accurate timing is paramount.Another frequency-determining device is the CRYSTAL. The crystal may be used with a tank circuit, or it may perform alone. Crystals exhibit a characteristic known as the PIEZOELECTRIC EFFECT. The piezoelectric effect is the property of a crystal by which mechanical forces produce electrical charges and, conversely, electrical charges produce mecha nical forces. This effect is a form of oscillation similar to the flywheel effect of a tank circuit. The piezoelectric effect can be seen in a number of crystal substances. The most important of these are the minerals quartz and Rochelle salt. Although quartz does not exhibit the piezoelectric effect to the degree that Rochelle salt does, quartz is used for frequency control in oscillators because of its greater mechanical strength. Another mineral, tourmaline, is physically strong like quartz; but because it is more expensive, it is not used extensively as an fdd.
This discussion will deal only with the quartz crystal. The crystals used in oscillator circuits are thin sheets, or wafers, cut from natural or synthetic quartz and ground to a specific thickness to obtain the desired resonant frequency. The crystals are mounted in holders, which support them physically and provide electrodes by which voltage is applied.
The holder must allow the crystals freedom for vibration. There are many different types of holders. One type is shown in the figure below. The frequency for which a crystal is ground is referred to as the NATURAL RESONANT FREQUENCY of the crystal. Voltage applied to the crystal produces mechanical vibrations which, in turn, produce an output voltage at the natural resonant frequency of the crystal.
A vibrating crystal can be represented by an equivalent electrical circuit composed of capacitance, inductance, and resistance. The figure below, view (A), illustrates the symbol of a crystal; view (B) shows an equivalent circuit for the crystal.
View (C) shows an equivalent circuit for the crystal and the holder; C1 represents the capacitance between the metal plates of the holder The Q (discussed in the tutorials on "Tuned Circuits") of a crystal is many times greater than that of an LC tank circuit. The high Q is present because the resistance in the crystal is extremely small. Commercially produced crystals range in Q from 5, 000 to 30, 000. The high Q causes the frequency stability to be much greater than that of an ordinary LC tank circuit.
This is the reason a crystal is used in many sine-wave generator circuits. As you have just studied, a basic oscillator can be broken down into three main sections: a frequency-determining device, an amplifier, and a feedback circuit. The frequency-determining device in an LC oscillator is usually an LC tank circuit. Although the tank circuit is normally found in the input circuit of an oscillator (both electron tube and transistor), it sometimes appears in the output circuit.
The differences in magnitude of plate and collector currents and shunting impedances are considerations in the designed locations of such tank circuits. In both solid-state and electron tube circuits, oscillations take place in the tuned circuit. Both the electron tube and the transistor function primarily as electrical valves that amplify and automatically deliver to the input circuit the proper amount of energy to sustain oscillations.
In both tube and transistor oscillators, the feedback circuit couples energy of the proper amount and of the correct phase from the output to the input circuit to sustain oscillations. The previous tutorials on "Tuned Circuits". described the resonant or tank circuit and how a sinusoidal signal is generated by the action of an inductor and a capacitor.
The feedback signal is coupled from this circuit by either of two means. The first method is to take some of the energy from the inductor. This can be done by any one of the three ways shown in the figure below, views 🔗 External reference
The crystal oscillator is a critical component in various electronic applications, providing precise frequency control essential for timing and synchronization in circuits. The operation of a crystal oscillator relies on the piezoelectric properties of the quartz crystal, which allows it to convert electrical energy into mechanical vibrations and vice versa. The natural resonant frequency is determined by the physical dimensions and the cut of the crystal, which can be optimized for specific applications.
In practical implementations, the crystal is often part of a feedback loop in an oscillator circuit, where it ensures stable oscillation frequencies. The equivalent circuit model of the crystal includes series and parallel resonant frequencies, which are crucial for understanding its behavior in different circuit configurations. The high Q factor indicates a narrow bandwidth around the resonant frequency, contributing to the oscillator's ability to maintain frequency stability over temperature variations and aging.
The design of the holder for the crystal is also significant, as it must not only support the crystal physically but also allow for its mechanical vibrations without introducing damping that could affect performance. The choice of materials for the holder and the method of mounting the crystal can influence the overall performance of the oscillator.
In summary, quartz crystals are indispensable in modern electronics, enabling precise frequency generation and control through their unique piezoelectric properties and high Q factors. Their application extends to various fields, including telecommunications, computing, and signal processing, where accurate timing is paramount.Another frequency-determining device is the CRYSTAL. The crystal may be used with a tank circuit, or it may perform alone. Crystals exhibit a characteristic known as the PIEZOELECTRIC EFFECT. The piezoelectric effect is the property of a crystal by which mechanical forces produce electrical charges and, conversely, electrical charges produce mecha nical forces. This effect is a form of oscillation similar to the flywheel effect of a tank circuit. The piezoelectric effect can be seen in a number of crystal substances. The most important of these are the minerals quartz and Rochelle salt. Although quartz does not exhibit the piezoelectric effect to the degree that Rochelle salt does, quartz is used for frequency control in oscillators because of its greater mechanical strength. Another mineral, tourmaline, is physically strong like quartz; but because it is more expensive, it is not used extensively as an fdd.
This discussion will deal only with the quartz crystal. The crystals used in oscillator circuits are thin sheets, or wafers, cut from natural or synthetic quartz and ground to a specific thickness to obtain the desired resonant frequency. The crystals are mounted in holders, which support them physically and provide electrodes by which voltage is applied.
The holder must allow the crystals freedom for vibration. There are many different types of holders. One type is shown in the figure below. The frequency for which a crystal is ground is referred to as the NATURAL RESONANT FREQUENCY of the crystal. Voltage applied to the crystal produces mechanical vibrations which, in turn, produce an output voltage at the natural resonant frequency of the crystal.
A vibrating crystal can be represented by an equivalent electrical circuit composed of capacitance, inductance, and resistance. The figure below, view (A), illustrates the symbol of a crystal; view (B) shows an equivalent circuit for the crystal.
View (C) shows an equivalent circuit for the crystal and the holder; C1 represents the capacitance between the metal plates of the holder The Q (discussed in the tutorials on "Tuned Circuits") of a crystal is many times greater than that of an LC tank circuit. The high Q is present because the resistance in the crystal is extremely small. Commercially produced crystals range in Q from 5, 000 to 30, 000. The high Q causes the frequency stability to be much greater than that of an ordinary LC tank circuit.
This is the reason a crystal is used in many sine-wave generator circuits. As you have just studied, a basic oscillator can be broken down into three main sections: a frequency-determining device, an amplifier, and a feedback circuit. The frequency-determining device in an LC oscillator is usually an LC tank circuit. Although the tank circuit is normally found in the input circuit of an oscillator (both electron tube and transistor), it sometimes appears in the output circuit.
The differences in magnitude of plate and collector currents and shunting impedances are considerations in the designed locations of such tank circuits. In both solid-state and electron tube circuits, oscillations take place in the tuned circuit. Both the electron tube and the transistor function primarily as electrical valves that amplify and automatically deliver to the input circuit the proper amount of energy to sustain oscillations.
In both tube and transistor oscillators, the feedback circuit couples energy of the proper amount and of the correct phase from the output to the input circuit to sustain oscillations. The previous tutorials on "Tuned Circuits". described the resonant or tank circuit and how a sinusoidal signal is generated by the action of an inductor and a capacitor.
The feedback signal is coupled from this circuit by either of two means. The first method is to take some of the energy from the inductor. This can be done by any one of the three ways shown in the figure below, views 🔗 External reference
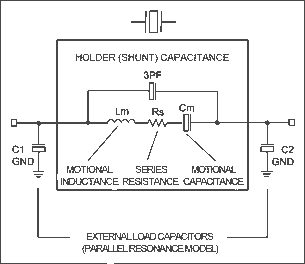
.jpg)